Quote: OnceDearWhat do you have in mind for ballpark min/max bets?
And will you both have the right to call a halt?
link to original post
Based on PM's, I have a high degree of confidence this challenge with LoquaciousMoFW will go off. It's possible we may need to use a proxy, if he can't make it person. Betting has been agreed to at $5 a bet. Of course, anything can be changed by mutual agreement. I never stated my own quitting point, but I am prepared to go for hours and will bring at least $1,000.
Wizard will roll two dice in a cup. If there are no 2s, he will reroll. If there is at least one 2, he will remove a die with a 2 and show it to you. There will be one die still under the cup.
You can bet if that second die is a 2. Wizard will pay you 7:1 if itís a 2.
Would you make that bet?
Quote: OnceDearwhat would be your motivation for playing this slightly -ev proposition?
To demonstrate that hit and run is a winning strategy.
The relationship between the 2-Dice problem and the Gambler's Fallacy is interesting to ponder.
Gambler's Fallacy: Separate rolls/trials are statistically independent. Given fair systems, the past record does not affect the future. What happens on one dice roll does not affect another dice roll.
Two-Dice Problem: Information about the outcome of an ensemble of distinct events can alter the probability of an individual event's outcome.
And what I am struck by is the similarity between the 2 Dice problem, and the concept of quantum entanglement in physics.
Quantum entanglement: the phenomenon whereby a pair of particles are generated in such a way that the individual quantum states of each are indefinite until measured, and the act of measuring one determines the result of measuring the other, even when at a distance from each other.
Quote: unJonAlan here is a bet for you.
Wizard will roll two dice in a cup. If there are no 2s, he will reroll. If there is at least one 2, he will remove a die with a 2 and show it to you. There will be one die still under the cup.
You can bet if that second die is a 2. Wizard will pay you 7:1 if itís a 2.
Would you make that bet?
link to original post
Unjon I've got a bet for you. Meet me at a craps table with $300. We will both shoot and bet only on our own shooting. After three turns each we'll see who has more money. You can bet pass or DP and as much or as little on yourself as you like.
Oh, the stakes? How about winner takes all?
Your maximum out of pocket is $300 but if one or both of us have winning rolls the winner could walk away with $600 plus the casino's money.
Now that's a worthwhile bet.
Quote: gordonm888Let me try to write something worth reading,
The relationship between the 2-Dice problem and the Gambler's Fallacy is interesting to ponder.
Gambler's Fallacy: Separate rolls/trials are statistically independent. Given fair systems, the past record does not affect the future. What happens on one dice roll does not affect another dice roll.
Two-Dice Problem: Information about the outcome of an ensemble of distinct events can alter the probability of an individual event's outcome.
And what I am struck by is the similarity between the 2 Dice problem, and the concept of quantum entanglement in physics.
Quantum entanglement: the phenomenon whereby a pair of particles are generated in such a way that the individual quantum states of each are indefinite until measured, and the act of measuring one determines the result of measuring the other, even when at a distance from each other.
link to original post
So funny, Gordon. I was actually pondering something similar this morning. I was wondering if a mechanic similar to the two dice problem could serve as the basis for a hidden variable that breaches Bellís Inequality.
Quote: AlanMendelsonQuote: unJonAlan here is a bet for you.
Wizard will roll two dice in a cup. If there are no 2s, he will reroll. If there is at least one 2, he will remove a die with a 2 and show it to you. There will be one die still under the cup.
You can bet if that second die is a 2. Wizard will pay you 7:1 if itís a 2.
Would you make that bet?
link to original post
Unjon I've got a bet for you. Meet me at a craps table with $300. We will both shoot and bet only on our own shooting. After three turns each we'll see who has more money. You can bet pass or DP and as much or as little on yourself as you like.
Oh, the stakes? How about winner takes all?
Your maximum out of pocket is $300 but if one or both of us have winning rolls the winner could walk away with $600 plus the casino's money.
Now that's a worthwhile bet.
link to original post
No thanks. I am just trying to engage you to try to get you to see 1/6 vs 1/11 difference. Your bet doesnít do anything for me. $300 is less than a passline bet with odds.
Quote: UnJonSo funny, Gordon. I was actually pondering something similar this morning. I was wondering if a mechanic similar to the two dice problem could serve as the basis for a hidden variable that breaches Bellís Inequality.
Hidden variables to undermine Bell's Inequality? Mic drop. Wow! Impressive. Sounds crazy, but it's fun to think about. And you never know . . .
I was wondering whether the probability of an ensemble of seemingly discrete random events that originate at the same point/time could be written as a probabilistic wavefunction in a way that would produce behavior that mirrors the behavior of quantumly entangled particles. Probably not, but . . .
I think your crazy daydream is a bit more fundamental than mine. Shows how a ridiculous thread like this could potentially have value.
Quote: unJonQuote: AlanMendelsonQuote: unJonAlan here is a bet for you.
Wizard will roll two dice in a cup. If there are no 2s, he will reroll. If there is at least one 2, he will remove a die with a 2 and show it to you. There will be one die still under the cup.
You can bet if that second die is a 2. Wizard will pay you 7:1 if itís a 2.
Would you make that bet?
link to original post
Unjon I've got a bet for you. Meet me at a craps table with $300. We will both shoot and bet only on our own shooting. After three turns each we'll see who has more money. You can bet pass or DP and as much or as little on yourself as you like.
Oh, the stakes? How about winner takes all?
Your maximum out of pocket is $300 but if one or both of us have winning rolls the winner could walk away with $600 plus the casino's money.
Now that's a worthwhile bet.
link to original post
No thanks. I am just trying to engage you to try to get you to see 1/6 vs 1/11 difference. Your bet doesnít do anything for me. $300 is less than a passline bet with odds.
link to original post
You're a much bigger gambler than I am, and at a -EV game. So you must be a DI to bet so much.
Quote: AlanMendelsonQuote: unJonQuote: AlanMendelsonQuote: unJonAlan here is a bet for you.
Wizard will roll two dice in a cup. If there are no 2s, he will reroll. If there is at least one 2, he will remove a die with a 2 and show it to you. There will be one die still under the cup.
You can bet if that second die is a 2. Wizard will pay you 7:1 if itís a 2.
Would you make that bet?
link to original post
Unjon I've got a bet for you. Meet me at a craps table with $300. We will both shoot and bet only on our own shooting. After three turns each we'll see who has more money. You can bet pass or DP and as much or as little on yourself as you like.
Oh, the stakes? How about winner takes all?
Your maximum out of pocket is $300 but if one or both of us have winning rolls the winner could walk away with $600 plus the casino's money.
Now that's a worthwhile bet.
link to original post
No thanks. I am just trying to engage you to try to get you to see 1/6 vs 1/11 difference. Your bet doesnít do anything for me. $300 is less than a passline bet with odds.
link to original post
You're a much bigger gambler than I am, and at a -EV game. So you must be a DI to bet so much.
link to original post
Despite my best efforts, I cannot lay claim to being a DI.
Sounds like a good plan. It is. I do it a lot online (For modest stakes)Quote: coachbellyQuote: OnceDearwhat would be your motivation for playing this slightly -ev proposition?
To demonstrate that hit and run is a winning strategy.
link to original post
So far as I look at my casino stats, I'm net positive for the last 24h, for the last 7 days, for the last 30 days AND for the last 3 months. It's fun..... Don't ask about the last six months or YTD though :o)
Good luck against Wizard. I hope it happens and goes well for you.
I would delight in seeing Wizard reporting such a session in which Alan had a lucky streak and won.Quote: unJonAlan here is a bet for you.
Wizard will roll two dice in a cup. If there are no 2s, he will reroll. If there is at least one 2, he will remove a die with a 2 and show it to you. There will be one die still under the cup.
You can bet if that second die is a 2. Wizard will pay you 7:1 if itís a 2.
Would you make that bet?
link to original post
But I seem to recall that last time plans fell through. Or was it that Alan doesn't generally do personal wagers.
That game will have a lot of pushes 25/36.
I reckon Alan has acknowledged that that bet is an 11:1 proposition and thus -EV. So I doubt he would. Maybe seeing the deuce will clinch it and they can play for nickels? It would make a fun trip report.
Quote: OnceDearI would delight in seeing Wizard reporting such a session in which Alan had a lucky streak and won.Quote: unJonAlan here is a bet for you.
Wizard will roll two dice in a cup. If there are no 2s, he will reroll. If there is at least one 2, he will remove a die with a 2 and show it to you. There will be one die still under the cup.
You can bet if that second die is a 2. Wizard will pay you 7:1 if itís a 2.
Would you make that bet?
link to original post
But I seem to recall that last time plans fell through. Or was it that Alan doesn't generally do personal wagers.
That game will have a lot of pushes 25/36.
I reckon Alan has acknowledged that that bet is an 11:1 proposition and thus -EV. So I doubt he would. Maybe seeing the deuce will clinch it and they can play for nickels? It would make a fun trip report.
link to original post
As I said the Wizard will win his bet. Also, as I said, it doesnt relate to the language of the original post.
So everyone is clear I'm disagreeing with the language, phrasing, diction of the post.
You disagree with me.
It won't be resolved by any bet.
I would still like the Wizard to present the original post to English professors to let them report on what it says.
If you don't think I havent already had two PhDs look at it you'd be seriously mistaken.
Quote: AlanMendelsonI would still like the Wizard to present the original post to English professors to let them report on what it says.
link to original post
I am done discussing the original wording. About the revised wording, I'm willing to have that reviewed. I think if I showed it to an English professor, they would want to know what I am asking of them.
To be honest, I don't know any English professors. Will professors or teachers in other topics do? How about our own published author, darkoz?
Quote:As I said the Wizard will win his bet.
You also said the answer, per the revised wording, is still 1/6. Why would I win if I'm paying 8 to 1 on something with a 1/6 chance of winning?
Indeed, We agree at last. Actually on two things. "It won't be resolved by any bet."Quote: AlanMendelsonYou disagree with me.
link to original post
Alan, if you are in Vegas, I'm confident, Wizard would love you to join him and LoquasciousMoFW and maybe coachbelly. You could be the official reporter.
Think how funny it would be if Wizard lost to an unlucky streak
See you in three days. Please don't change you mind in my absence.
Quote: AlanMendelsonI am with my ex wife who is getting leukemia treatments in Salt Lake City. My ability to be in any casino is limited to what she wants and can do.
link to original post
I'm sorry to hear that, Alan. Leukemia is not good. A friend of mine just died from that yesterday. May your wife beat it and as painlessly as possible.
Quote: WizardQuote: AlanMendelsonI am with my ex wife who is getting leukemia treatments in Salt Lake City. My ability to be in any casino is limited to what she wants and can do.
link to original post
I'm sorry to hear that, Alan. Leukemia is not good. A friend of mine just died from that yesterday. May your wife beat it and as painlessly as possible.
link to original post
Janet beat lung cancer 5 years ago when we were married. She was hit with leukemia in January of last year. I've been her caregiver while she's been at a cancer center here in Salt Lake City.
Sorry to here this, Alan. Wishing you both the best possible outcome.Quote: AlanMendelson
Janet beat lung cancer 5 years ago when we were married. She was hit with leukemia in January of last year. I've been her caregiver while she's been at a cancer center here in Salt Lake City.
link to original post
Don't worry about the damn dice.
Quote: gordonm888Quote: UnJonSo funny, Gordon. I was actually pondering something similar this morning. I was wondering if a mechanic similar to the two dice problem could serve as the basis for a hidden variable that breaches Bellís Inequality.
Hidden variables to undermine Bell's Inequality? Mic drop. Wow! Impressive. Sounds crazy, but it's fun to think about. And you never know . . .
I was wondering whether the probability of an ensemble of seemingly discrete random events that originate at the same point/time could be written as a probabilistic wavefunction in a way that would produce behavior that mirrors the behavior of quantumly entangled particles. Probably not, but . . .
I think your crazy daydream is a bit more fundamental than mine. Shows how a ridiculous thread like this could potentially have value.
link to original post
Probably needs a new thread if this has legs (probably doesnít).
Iím not sure we are saying different things. If you can create an ensemble of classical processes that mimics a harmonic oscillator that follows Bornís rule (thatís the hard part), then it should be able to be manipulated into violating Bellís inequality.
There is a germ of something here. Thereís an idea of why a quantum particle is different than a classical particle, because a quantum particle is fully defined by its quantum numbers, and a quantum system is identical if you swap quantum particles among each other. Thatís almost sorta like saying quantum particles are indistinguishable in the same way that you donít know ďwhichĒ die shows a 2, when all you know is that at least one die shows a 2.
Anyway.
Quote: AlanMendelsonJanet beat lung cancer 5 years ago when we were married. She was hit with leukemia in January of last year. I've been her caregiver while she's been at a cancer center here in Salt Lake City.
link to original post
Again, I'm very sorry Alan. I feel like quite an ass now for the mean things I said to you.
Quote: WizardQuote: AlanMendelsonJanet beat lung cancer 5 years ago when we were married. She was hit with leukemia in January of last year. I've been her caregiver while she's been at a cancer center here in Salt Lake City.
link to original post
Again, I'm very sorry Alan. I feel like quite an ass now for the mean things I said to you.
link to original post
A lot of people are surprised I dropped everything about a year and a half ago when Janet called me and said she needed my help. After all, we had a brief and very turbulent marriage. But God was good to me with a blessed career and a miraculous kidney and pancreas transplant and it was my turn to give back and give to her in spite of what happened five years earlier.
Besides that... the nurses say we make a cute couple.
And... she also believes in quitting when ahead, that the answer is 1/6, she plays keno and knows how to milk the casinos for comps and free play. She's also a former beauty queen. What more could I want?
Please undo the suspensions. There's no need for them.
I'm a big boy. And I'm sure the correct response to the original question as it was written is 1/6.
There are twelve sides the dice can land on. We know at least one landed on a six so we have eleven sides unseen and only one Six so the odds the other landed on Six are 1:11. But are the other sides all unseen? Don't we know what is opposite the Six? If one of the twelve sides is a six, then another of those 12 has to be a One. So do we have eleven unknown sides or ten?
Quote: billryanSo I know I'm wrong, but don't know why I'm wrong.
There are twelve sides the dice can land on. We know at least one landed on a six so we have eleven sides unseen and only one Six so the odds the other landed on Six are 1:11. But are the other sides all unseen? Don't we know what is opposite the Six? If one of the twelve sides is a six, then another of those 12 has to be a One. So do we have eleven unknown sides or ten?
link to original post
You are visualizing it wrong. Itís not 1/11 because of 11 unknown faces. Itís 1/11 because there are 36 ways to roll two dice, and 11 of those 36 ways have at least one 6 facing up. Of those 11, only 1 way has two 6s facing up.
Here is a pic of the 36 outcomes of rolling two dice. Iíve circled the 11 that contain at least one 2. And the intersection of the red circles shows the 1/11 with two 2s.
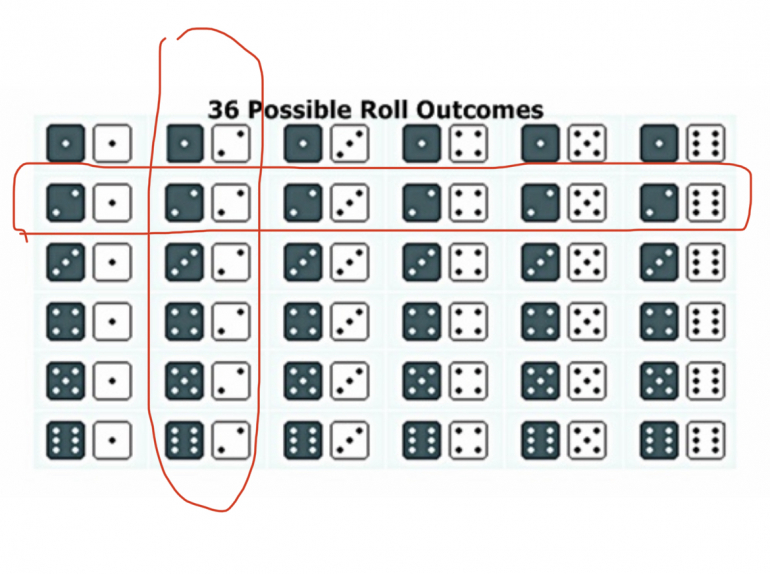
You could do the same for any particular number.
Billy is pointing out that we know that opposite faces of each die add up to 7.
So when a die shows a 6 we know it can't show a 1 because the 1 is opposite the six.
So it eliminates a face to be considered.
This goes beyond looking at a graphic. You have to think about it.
Quote: AlanMendelsonBilly brought up a good point and I have to say that it supports my 1/6.
Billy is pointing out that we know that opposite faces of each die add up to 7.
So when a die shows a 6 we know it can't show a 1 because the 1 is opposite the six.
So it eliminates a face to be considered.
This goes beyond looking at a graphic. You have to think about it.
link to original post
When a die shows a 6 we know it canít show a 1 or a 2, 3, 4 or 5. Because we know it shows a 6.
Quote: unJonQuote: AlanMendelsonBilly brought up a good point and I have to say that it supports my 1/6.
Billy is pointing out that we know that opposite faces of each die add up to 7.
So when a die shows a 6 we know it can't show a 1 because the 1 is opposite the six.
So it eliminates a face to be considered.
This goes beyond looking at a graphic. You have to think about it.
link to original post
When a die shows a 6 we know it canít show a 1 or a 2, 3, 4 or 5. Because we know it shows a 6.
link to original post
Wow.
I want to defend Billy here. He is raising the point of excluding a number on a die in a problem where it is said "we don't know which die is viewed" (or words to that effect).
This is the argument used to justify the 1/11 answer when a 2 shows in the 2 dice problem.
Following Billy's suggestion, the 5 face should also be eliminated because its the opposite side of any 2.
This would change an answer from 1/11 to 1/10 as Billy pointed out.
Quote: AlanMendelsonQuote: unJonQuote: AlanMendelsonBilly brought up a good point and I have to say that it supports my 1/6.
Billy is pointing out that we know that opposite faces of each die add up to 7.
So when a die shows a 6 we know it can't show a 1 because the 1 is opposite the six.
So it eliminates a face to be considered.
This goes beyond looking at a graphic. You have to think about it.
link to original post
When a die shows a 6 we know it canít show a 1 or a 2, 3, 4 or 5. Because we know it shows a 6.
link to original post
Wow.
I want to defend Billy here. He is raising the point of excluding a number on a die in a problem where it is said "we don't know which die is viewed" (or words to that effect).
This is the argument used to justify the 1/11 answer when a 2 shows in the 2 dice problem.
Following Billy's suggestion, the 5 face should also be eliminated because its the opposite side of any 2.
This would change an answer from 1/11 to 1/10 as Billy pointed out.
link to original post
Wow.
No it doesnít. Thereís nothing special about the face opposite the 2 vs the face left, right, front or back of the 2. They are all equally not the top face of a die with a 2 on top.
Quote: unJonQuote: AlanMendelsonQuote: unJonQuote: AlanMendelsonBilly brought up a good point and I have to say that it supports my 1/6.
Billy is pointing out that we know that opposite faces of each die add up to 7.
So when a die shows a 6 we know it can't show a 1 because the 1 is opposite the six.
So it eliminates a face to be considered.
This goes beyond looking at a graphic. You have to think about it.
link to original post
When a die shows a 6 we know it canít show a 1 or a 2, 3, 4 or 5. Because we know it shows a 6.
link to original post
Wow.
I want to defend Billy here. He is raising the point of excluding a number on a die in a problem where it is said "we don't know which die is viewed" (or words to that effect).
This is the argument used to justify the 1/11 answer when a 2 shows in the 2 dice problem.
Following Billy's suggestion, the 5 face should also be eliminated because its the opposite side of any 2.
This would change an answer from 1/11 to 1/10 as Billy pointed out.
link to original post
Wow.
No it doesnít. Thereís nothing special about the face opposite the 2 vs the face left, right, front or back of the 2. They are all equally not the top face of a die with a 2 on top.
link to original post
I'm glad you wrote this because this goes hand-in-hand with the reasoning against the 1/6 answer.
I'm flabbergasted.
Quote: AlanMendelsonQuote: unJonQuote: AlanMendelsonQuote: unJonQuote: AlanMendelsonBilly brought up a good point and I have to say that it supports my 1/6.
Billy is pointing out that we know that opposite faces of each die add up to 7.
So when a die shows a 6 we know it can't show a 1 because the 1 is opposite the six.
So it eliminates a face to be considered.
This goes beyond looking at a graphic. You have to think about it.
link to original post
When a die shows a 6 we know it canít show a 1 or a 2, 3, 4 or 5. Because we know it shows a 6.
link to original post
Wow.
I want to defend Billy here. He is raising the point of excluding a number on a die in a problem where it is said "we don't know which die is viewed" (or words to that effect).
This is the argument used to justify the 1/11 answer when a 2 shows in the 2 dice problem.
Following Billy's suggestion, the 5 face should also be eliminated because its the opposite side of any 2.
This would change an answer from 1/11 to 1/10 as Billy pointed out.
link to original post
Wow.
No it doesnít. Thereís nothing special about the face opposite the 2 vs the face left, right, front or back of the 2. They are all equally not the top face of a die with a 2 on top.
link to original post
I'm glad you wrote this because this goes hand-in-hand with the reasoning against the 1/6 answer.
I'm flabbergasted.
link to original post
I can point you to several posts I made to you explaining when itís 1/6 vs 1/11. Itís slightly frustrating because you never responded to them so I assume you didnít read them.
Short answer: if you know which die in particular has the 2, itís 1/6. If you donít know which of the two dice has a 2, itís 1/11.
Exactly.Quote: unJonShort answer: if you know which die in particular has the 2, itís 1/6. If you donít know which of the two dice has a 2, itís 1/11.
Likewise with The Woman with the Two Children problem which I posted earlier.
Census Taker: How many children do you have?
Woman: Two.
Census Taker: And their ages?
Woman: One is five, one is two.
Census Taker: Is one of them a girl?
Woman: Yes.
The answer to the question "What is the probability her other child is a girl?" is 1/3.
However, what if the census taker had asked "Is the five-year old a girl?" and the woman had answered "Yes."
Here, the probability that the two-year old is a girl is indeed 1/2.
And why should asking about their ages make a difference? Because once one of the children is identified, there are only two possibilities for the other child.
Just like with the dice.
After all he brought it up.
Quote: unJon(snip)
Short answer: if you know which die in particular has the 2, itís 1/6. If you donít know which of the two dice has a 2, itís 1/11.
link to original post
I have been following this thread, and even though I haven't read every post, I agree that when the question uses terms*** similar to "...at least one die is a 2..." , then the answer is 1/11.
***: If the question used terms like, "...if Die A is showing a 2, what is the chance that Die B is also showing a 2...". then the answer would be 1/6 for Die B (IMO, this is the gist of what most people have been saying).
Also, there is another reason why I need to understand this kind of problem, and that is because I bet on a lot of "same game multis^^^ ", where I know that "at least one leg out of the two legs" is going to win before the event even starts.
^^^: Recently I had a "same game multi" in the NRL (Rugby League), and received the following: "Team A +44.5 x Over 35.5" at the odds of $1.84 (about -119).
Note 1: If I had the bet as "two single bets", then the "non-correlated " estimated chances were about 86% for "Team A +44.5" and 75% for "Over 35.5".
Note 2: IMO, this kind of "correlation" is an extra step that is not needed for the "two dice puzzle", but I have to account for it in a "same game multi" like the one above, because it is generally assumed that taking the "underdog with the over" has a "negative correlation".
Quote: AlanMendelsonBilly brought up a good point and I have to say that it supports my 1/6.
Billy is pointing out that we know that opposite faces of each die add up to 7.
So when a die shows a 6 we know it can't show a 1 because the 1 is opposite the six.
So it eliminates a face to be considered.
This goes beyond looking at a graphic. You have to think about it.
link to original post
I didn't make a good point. I made a point I knew was wrong but didn't know why. It was explained in simple terms and now I understand it.
Quote: billryanQuote: AlanMendelsonBilly brought up a good point and I have to say that it supports my 1/6.
Billy is pointing out that we know that opposite faces of each die add up to 7.
So when a die shows a 6 we know it can't show a 1 because the 1 is opposite the six.
So it eliminates a face to be considered.
This goes beyond looking at a graphic. You have to think about it.
link to original post
I didn't make a good point. I made a point I knew was wrong but didn't know why. It was explained in simple terms and now I understand it.
link to original post
Except your point was correct. When you know one number on a die the opposite number is also known.
And here's something else our friends overlooked with their 1/11 answer:
In the real world you may even be able to remove more die faces from consideration based on how the die lands. This is because of the numbering system on right handed vs left handed dice.
Quote: unJon
When a die shows a 6 we know it canít show a 1 or a 2, 3, 4 or 5. Because we know it shows a 6.
link to original post
I guess this means that the remaining die in a 2 dice problem is a 1/6 probability?
I guess it also means you can't have a 1/11 probability?
Quote: AlanMendelsonUnjon please consider your quote below. I have some questions.
Quote: unJon
When a die shows a 6 we know it canít show a 1 or a 2, 3, 4 or 5. Because we know it shows a 6.
link to original post
I guess this means that the remaining die in a 2 dice problem is a 1/6 probability?
I guess it also means you can't have a 1/11 probability?
link to original post
Iíll try again. But you wonít like it. Because it will include pictures.
Letís say thereís a white die and a black die. And we roll the two of them. And you canít see the result but I can.
Letís say you ask me: unJon, the white die a 6, and I say yes.
What is the chance that the black die is a 6?
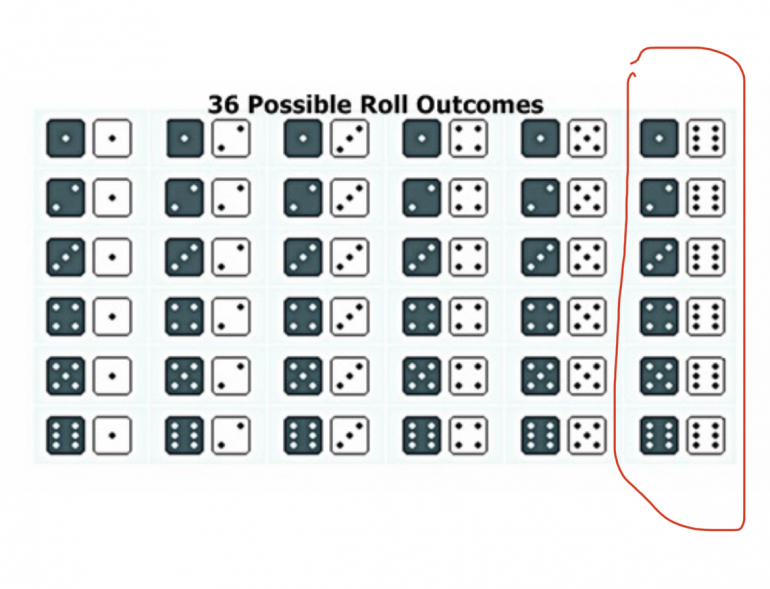
From my answer you know we are in the area of the red circle of 6 possible rolls. 1 of them has a black 6. Therefore 1/6.
And now we roll the die again.
Letís say you ask me: unJon is the black die a 6, and I say yes.
What is the chance that the white die is a 6?
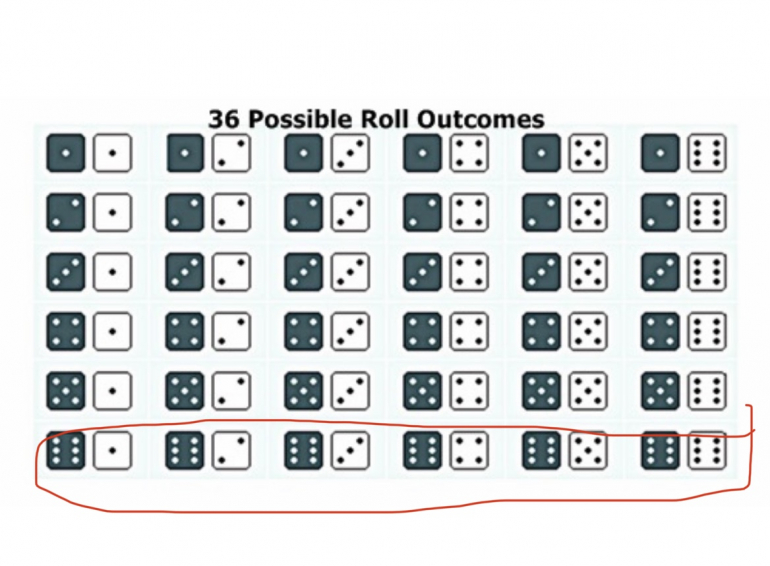
From my answer you know we are now in this circle. Where all the black die are 6. Six possibilities and 1 had a white 6. So 1/6.
And now we roll the dice a third time.
Letís say you ask me: unJon, are at least one of the die a 6, and I say yes.
Now you donít know if the 6 is on the white die or the black die or both dice. You asked a question that gave you less information than the first two rolls. So whatís the probability that the other die is a 6?
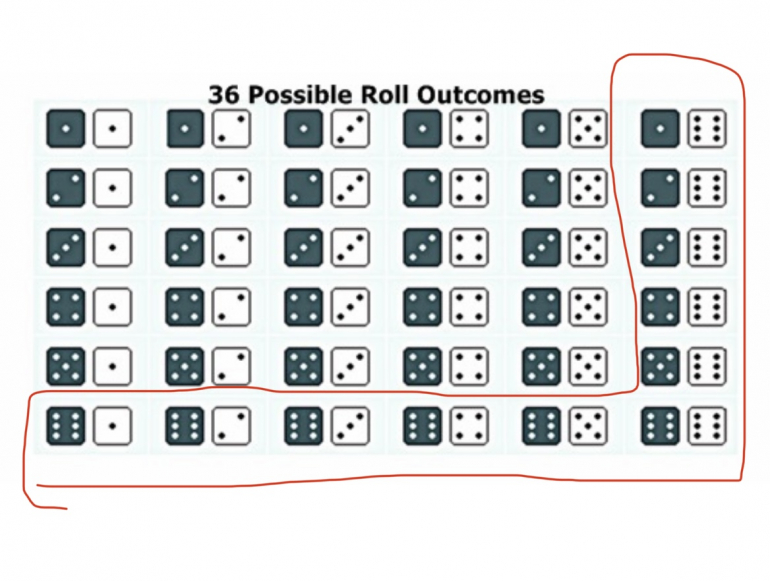
Now you are in this red circle, where one or the other die has a 6. There are 11 possible rolls that fit my answer. 1 of them has two sixes. So 1/11.
I leave as an exercise to the reader to repeat this simple experiment at home or in another place with a friend or spouse or ex-spouse. And see after many rolls if your experiments agree.
Recall the original question was this:
You have two 6-sided dice in a cup. You shake the dice, and slam the cup down onto the table, hiding the result. Your partner peeks under the cup, and tells you, truthfully, At least one of the dice is a 2. What is the probability that both dice are showing a 2?
At least one of the dice is a 2. That's the information we are given. You aren't told which die, the black die or the white die. You aren't told which die, the left die or the right die. You aren't told which die, the large die or the small die. All you are told was at least one of dice was a 2.
What is the probability that both dice are a 2? As has been demonstrated, the answer is clearly 1/11.
A few hundred posts later, almost all the skeptics were converted or they left the thread. Only one or two holdouts remain.
I proposed the following bet- We roll the dice until we have 1000 rolls of one or more sixes. At that point, he gets paid $8 for every pair and he pays $1 for every non-pair.
So he says he did the rolls, only he stipulated that one die was a Six, rolled a single dice a bunch of times, noted the six came up roughly one-sixth of the time and said that he proved the answer was 1/6. Now he is more convinced than ever and pointing to his video as proof.
Quote: EdCollins
At least one of the dice is a 2. That's the information we are given. You aren't told which die, the black die or the white die. You aren't told which die, the left die or the right die. You aren't told which die, the large die or the small die. All you are told was at least one of dice was a 2.
link to original post
I just realized what my missing link is here. I havent been able to grasp why it matters which of the dice it is?
Please explain to me why this matters? Why does it matter which die is first identified?
My brain is able to figure 1/6 with either die.
Quote: AlanMendelsonQuote: EdCollins
At least one of the dice is a 2. That's the information we are given. You aren't told which die, the black die or the white die. You aren't told which die, the left die or the right die. You aren't told which die, the large die or the small die. All you are told was at least one of dice was a 2.
link to original post
I just realized what my missing link is here. I havent been able to grasp why it matters which of the dice it is?
Please explain to me why this matters? Why does it matter which die is first identified?
My brain is able to figure 1/6 with either die.
link to original post
My post explains exactly that. With pictures!
Quote: unJonQuote: AlanMendelsonQuote: EdCollins
At least one of the dice is a 2. That's the information we are given. You aren't told which die, the black die or the white die. You aren't told which die, the left die or the right die. You aren't told which die, the large die or the small die. All you are told was at least one of dice was a 2.
link to original post
I just realized what my missing link is here. I havent been able to grasp why it matters which of the dice it is?
Please explain to me why this matters? Why does it matter which die is first identified?
My brain is able to figure 1/6 with either die.
link to original post
My post explains exactly that. With pictures!
link to original post
I dont understand why you have to know which die.
I'm very content knowing that no matter what die is rolled a second die has a 1/6 chance of matching it.
I'm curious... do you agree with the answer to the Woman with Two Children problem?Quote: AlanMendelsonI dont understand why you have to know which die.
Census Taker: How many children do you have?
Woman: Two.
Census Taker: And their ages?
Woman: One is five, one is two.
Census Taker: Is one of them a girl?
Woman: Yes.
What is the probability that her other child is a girl?
Quote: AlanMendelsonQuote: EdCollins
At least one of the dice is a 2. That's the information we are given. You aren't told which die, the black die or the white die. You aren't told which die, the left die or the right die. You aren't told which die, the large die or the small die. All you are told was at least one of dice was a 2.
link to original post
I just realized what my missing link is here. I havent been able to grasp why it matters which of the dice it is?
Please explain to me why this matters? Why does it matter which die is first identified?
My brain is able to figure 1/6 with either die.
link to original post
We can only use what we know, without making any assumptions.
We know there are 36 possible outcomes from rolling two dice.
We know there is at least one six.
We know there are 25 possible outcomes that involve not having a six, so we eliminate them all.
What do we have left? 11 possible outcomes.
Do any of them matter if the six is on the northernmost die or the southernmost die?
It does not, because whichever die has the six on it, it still leaves only eleven possibilities.
Of the eleven possibilities, there is only one that has two Sixes
If we knew the Six was on the northern die, then the chances of a match with the southern die would be 1/6, but we don't know that.
If we knew the Six was on the southern die, the exact same thing.
In both cases, we have used the knowledge we have to eliminate possibilities.
Since we don't know which die has the Six, we can't make assumptions.
There are exactly 11 combinations that contain at least one six, and only one combination that gives you a pair.
Using only what we know, it doesn't matter which die has the Six.
We don't have to make any assumptions because there is only one possible combination that gives us a pair
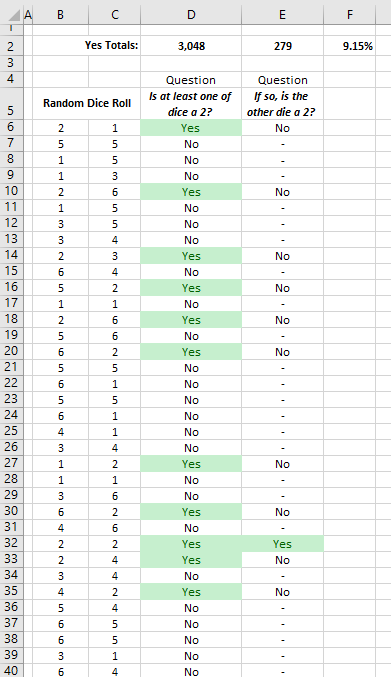
I had it simulate 10,000 random dice rolls.
After each roll, the question was posed, "Is at least one of the dice a 2?" and the program listed the proper answer.
If the answer to that question was "Yes" a second question was asked, "Is the other die a 2?" and the program listed the proper answer.
For this run, only 279 times out of 3,048 (9.15%) was the other die a 2. (1/11 is, of course, 9.09%)
All f the other six or seen times I ran the simulation, resulted in nearly identical percentages.
Space prevents me from listing all 10,000 rolls, but here's a screenshot.
Then I think back to the original question. And the original question seems to ask me the same thing: when you have a face on a die what are the odds another die will match it?
Of course I didnt go to a math class that was trying to teach you some lesson in probability. All I did was use my memory to remember that a die has six faces with a different number, one thru six, on each face.
I'm also not comparing this question to how other questions are asked or answered. I'm not comparing this to census takers or TV hosts checking on kids or goats.
I'm simply remembering that if one die with six faces is showing one number, any other die with six faces will have one face matching that number.
Why is my approach which is so simple so wrong?


