Poll
 | 25 votes (49.01%) | ||
 | 16 votes (31.37%) | ||
 | 7 votes (13.72%) | ||
 | 4 votes (7.84%) | ||
 | 12 votes (23.52%) | ||
 | 3 votes (5.88%) | ||
 | 6 votes (11.76%) | ||
 | 5 votes (9.8%) | ||
 | 12 votes (23.52%) | ||
 | 10 votes (19.6%) |
51 members have voted
ACED is a square of side length 2. AB = BC. What is the area of the blue region?
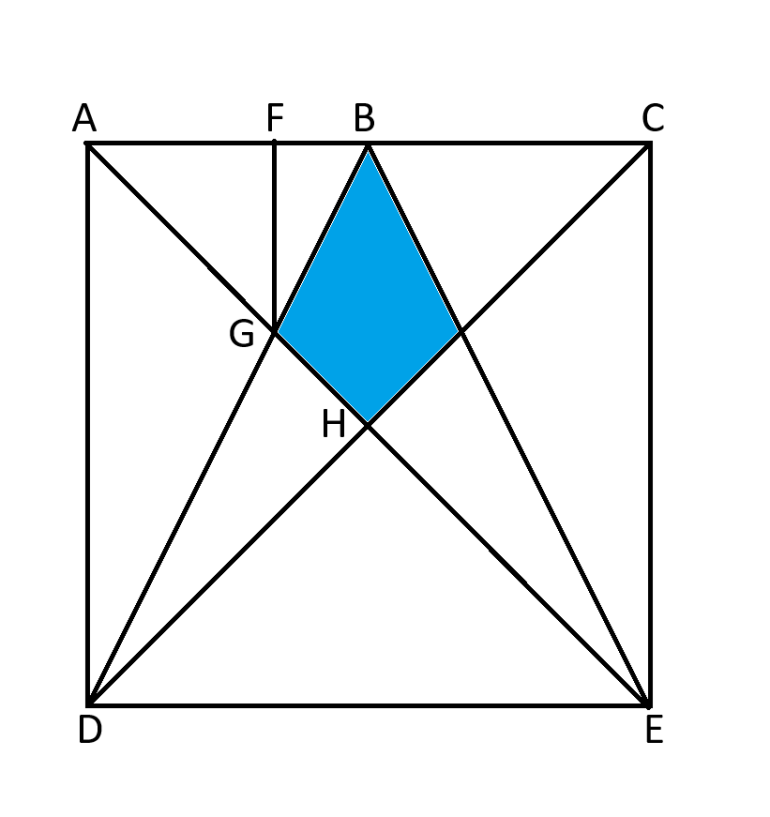
I label some extra points for purposes of discussion.
By similar triangles JKB has sides in the ratio of 2 to 1 (ref BCE), and JKH has equal sides (ref CBH), thus one can say that JK=KH=x and KB=2x. But BH=3x=1. So x=1/3.
So area of JKH = 1/3 * 1/3 * 1/2 = 1/18.
Area of LKB is twice this = 2/18.
So total of the four triangles that make up BGHJ = 6/18 = 1/3.
Another way of looking it is consider copying move BGK to the right of BKJ and similarly KGH moves, to form the rectangle. This rectangle has height of 1 (BH) and width = 1/3; hence the area = 1/3.
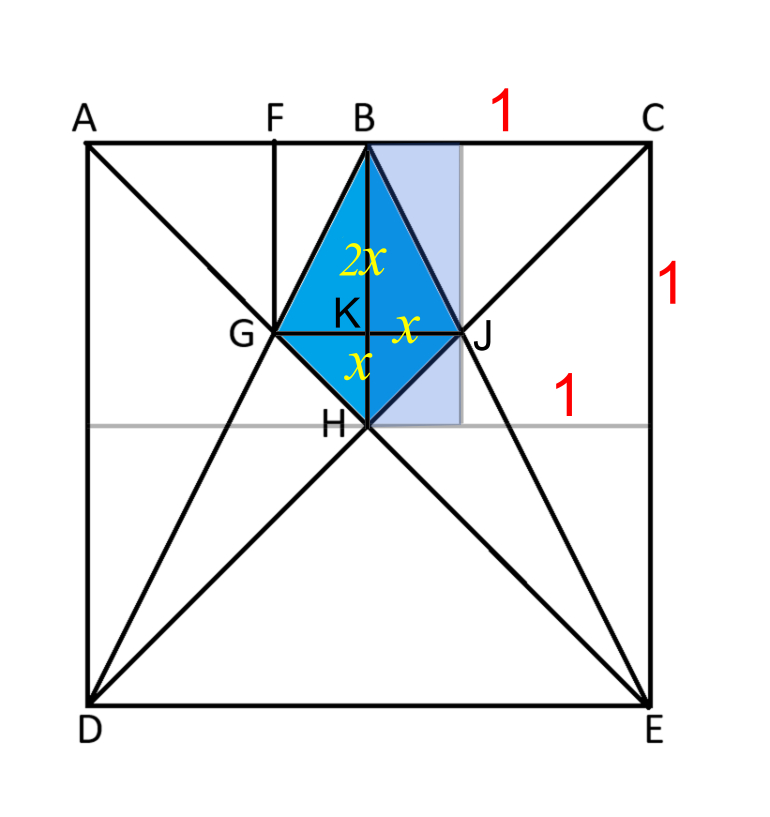
Quote: charliepatrickI've constructed a few more lines.
By similar triangles JKB has sides in the ratio of 2 to 1 (ref BCE), and JKH has equal sides (ref CBH), thus one can say that JK=KH=x and KB=2x. But BH=3x=1. So x=1/3.
So area of JKH = 1/3 * 1/3 * 1/2 = 1/18.
Area of LKB is twice this = 2/18.
So total of the four triangles that make up BGHJ = 6/18 = 1/3.
Another way of looking it is consider copying move BGK to the right of BKJ and similarly KGH moves, to form the rectangle. This rectangle has height of 1 (BH) and width = 1/3; hence the area = 1/3.
link to original post
I agree! Here is my solution (PDF).
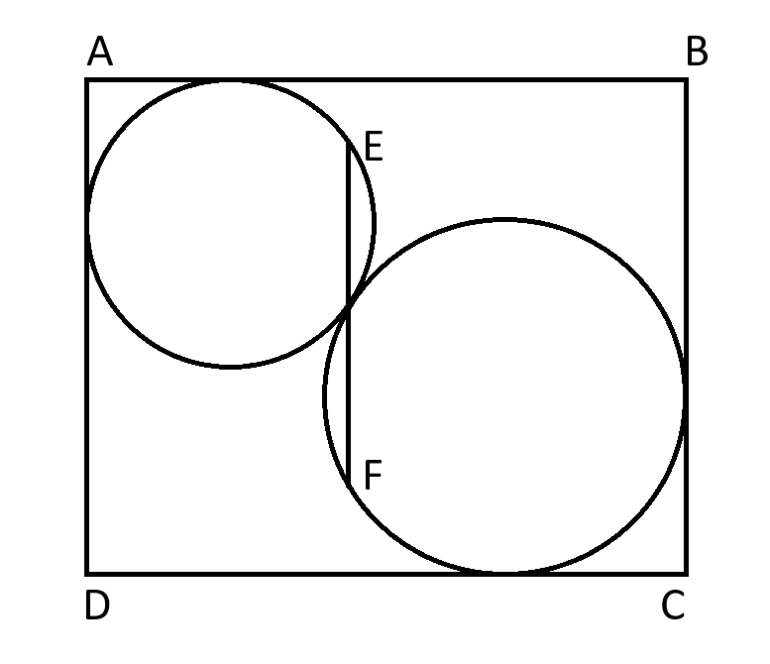
Two circles are inscribed in a rectangle of height 81. There is a line segment of length 56 extending to the edge of both circles and goes through where the circles meet and is parallel to the vertical edge of the rectangle.
How wide is the rectangle?
Solution: Join the centre of both circles. Let the centre of the small circle be C1 and the centre of the large circle be C2. Let the radius of the small circle be R1 and the radius of the large circle be R2. Call the intersection point M.
Draw a line from C1 to meet EF at right angles and call this point X. Draw a line from C2 to meet EF at right angles and call this point Y.
By the law of triangles XY = EF/2 = 56/2 = 28.
By deduction: radius of large circle (R2) = (81 - 28 - R1) = 53 - R1
Distance C1-C2 = 53 - R1 + R1 = 53.
Extend line C2-Y to AD and draw a line from C1 parallel with AD to meet DC. Call the intersection point of the two construction lines point Z.
By deduction: C1-Z = 28,
By deduction: C2-Z = Sq, root (53*53 - 28*28) = 45
Answer: CD = R1 + 45 + 53 - R1
= 98
Quote: davethebuilder
Answer: CD = R1 + 45 + 53 - R1
= 98
link to original post
I agree.
Dave, can you please put answers in the future in spoiler tags.
By the way, there's a typo in the first line, it should read AD=BC=81.
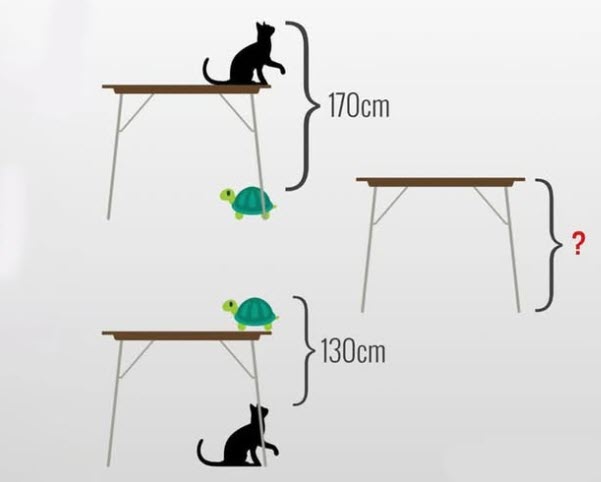
Here is an easy puzzle that came up on my Facebook feed.
Quote: davethebuilderLet the height of the table be x Let the height of the Cat be y Let the height of the Tortoise be z By deduction: x = 170 y + z By deduction: x = 130 + y z Solving: y = z + 20 Substituting: x = 170 (z + 20) + z Answer: x = 150cm Check: x = 130 + (z + 20) z Answer: x = 150cm
link to original post
I agree.
You can download the PDF here: https://www.gchq.gov.uk/news/gchq-christmas-challenge-2024 or https://www.gchq.gov.uk/files/GCHQ%20Christmas%20Challenge%202024.pdf
Edit added SPOILER : I've found, but not read, the answers can be found here : https://www.pressreader.com/uk/daily-mail/20241212/282093462326112
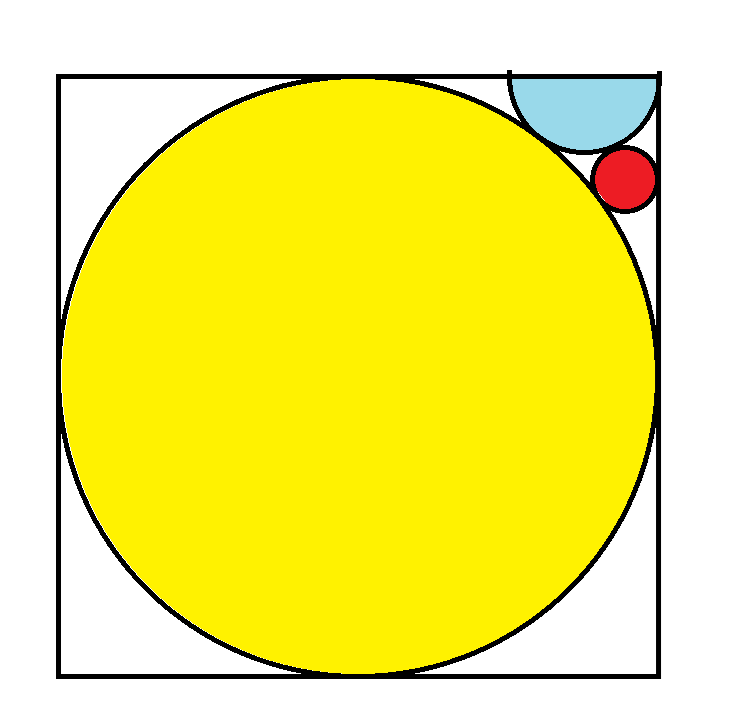
The yellow circle has a radius of 1. What is the area of the red circle?
Let C be the height of the cat, T the height of the turtle, and X the height of the table, which is the desired value
Top image: C + X - T = 170
Bottom image: T + X - C = 130
Add them up: (C - C) + 2X + (T - T) = 300
2X = 300, so X = the height of the table = 150 cm
Quote: charliepatrickThe annual GCHQ puzzle appeared yesterday, I don't know the answers but they were in the paper today.
link to original post
I got into a bit of a discussion about this over on the Straight Dope Message Board:
Santa's trail says, "We Wish You a Merry Christmas" twice, in Morse code - but since it's British (especially as puzzle 2 expects you to know what a Blue Peter Badge and Blackpool Tower are), shouldn't it be, "Happy Christmas"?
Quote: WizardThe yellow circle has a radius of 1. What is the area of the red circle?
link to original post
Let Y be the center of the yellow circle, R the center of the red circle, B the center of the blue semicircle, and P and Q the points of tangency of the yellow circle to the top and right edges of the bounding square.
Let x be the radius of the red circle, and y the radius of the yellow semicircle.
Since the distance from the center of the circle to Q is 1, the distance from P to the upper right corner of the square is also 1, so the distance from P to B is 1 - y.
Also, since the yellow and blue circles are tangent, YB = the sum of their radii = 1 + y.
Pythagorean Theorem: 1^2 + (1 - y)^2 = (1 + y)^2
y = 1/4
Draw a line through R that is perpendicular to the top side (and parallel to the right side) of the square.
Let C be the point where this line intersects the top of the square.
Since the line segment from R to the right edge of the square is a radius of R, its distance is x, which means the distance from C to the upper right corner of the square is also x, and CB has length y - x. Also, since the blue semicircle and red circle are tangent, RB = y + x.
Pythagorean Theorem: (y - x)^2 + z^2 = (y + x)^2, or z^2 = (y + x)^2 - (y - x)^2 = 4xy = x (since y = 1/4).
CR = sqrt(x)
Let D be the point where YQ intersects the line through R parallel to the right edge; RD = 1 - sqrt(x), and YD = 1 - x
Since the yellow and red circles are tangent, YR = 1 + x
Pythagorean Theorem: (1 - sqrt(x))^2 + (1 - x)^2 = (1 + x)^2 = 4x
Take the square root of both sides: 1 - sqrt(x) = 2 sqrt(x), or 1 = 3 sqrt(x)
sqrt(x) = 1/3, so the radius of the red circle = (1/3)^2 = 1/9.
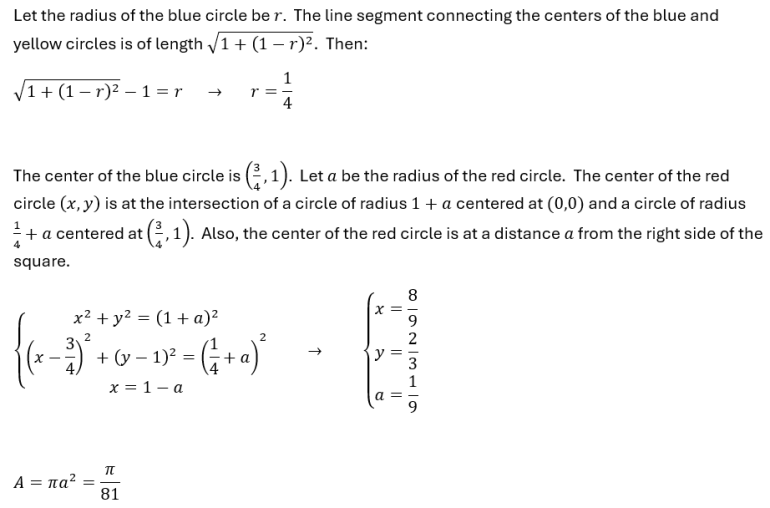
Quote: WizardDon & CD -- I agree!
link to original post
But ChesterDog gets the beer this time because you did ask for the area, and my answer was the radius.
x^2 - y^2 = 64
xy = 8
Find x+y.
Quote: WizardHere is another one that came through my Facebook feed:
x^2 - y^2 = 64
xy = 8
Find x+y.
link to original post
x^2 - (8/x)^2 = 64
x^2 - 64 / x^2 = 64
(x^2)^2 - 64 = 64 x^2
(x^2)^2 - 64 x^2 - 64 = 0
(x^2)^2 - 64 x^2 + 32^2 = 32 * 34 = 64 * 17
(x^2 - 32)^2 = (8 sqrt(17))^2
x^2 = 32 +/- 8 sqrt(17)
y^2 = x^2 - 64 = +/- 8 sqrt(17) - 32
x^2 + y^2 = 16 sqrt(17)
(x + y)^2 = x^2 + 2xy + y^2 = x^2 + y^2 + 16 = 16 + 16 sqrt(17)
x + y = +/- 4 sqrt(1 + sqrt(17))
I know the positive is correct; not sure about the negative
Quote: ThatDonGuy
x^2 - (8/x)^2 = 64
x^2 - 64 / x^2 = 64
(x^2)^2 - 64 = 64 x^2
(x^2)^2 - 64 x^2 - 64 = 0
(x^2)^2 - 64 x^2 + 32^2 = 32 * 34 = 64 * 17
(x^2 - 32)^2 = (8 sqrt(17))^2
x^2 = 32 +/- 8 sqrt(17)
y^2 = x^2 - 64 = +/- 8 sqrt(17) - 32
x^2 + y^2 = 16 sqrt(17)
(x + y)^2 = x^2 + 2xy + y^2 = x^2 + y^2 + 16 = 16 + 16 sqrt(17)
x + y = +/- 4 sqrt(1 + sqrt(17))
I know the positive is correct; not sure about the negative
link to original post
That's not what I got, but maybe I'm in the one in error. Hopefully we get a third opinion.
x² - y² = 64
xy = 8
Find x + y
Solution:
If xy = 8, y = 8/x
x² - (8/x)² = 64
x² - (64/x²) = 64
(x²)² - 64x² - 64 = 0
Convert quartic equation into quadratic form by letting a = x²
a² - 64a - 64 = 0
a = [Sq. root 64 + (Sq. root (64*68))]/2
x = Sq. root {[Sq. root 64 + (Sq. root (64*68))]/2}
x = 8.061317
Substituting: y = 8/x
y = 8/8.061317
= 0.992393
Solution: x + y = 8.061317 + 0.992393
= 9.05371
Using trial and error these values of x and y come close:
x = 8.061315
y = 0.992370661
xy = 7.999812497
x+y = 9.053685661
I was trying to argue why my answer was also right, but in putting it in writing for this post, I caught my error.
1. What is the expected number of rolls, including the ending roll?
2. Is there a formula for the expected rolls for an n-sided die?
Quote: WizardYou roll an icosahedron (20-sided die) until it lands on the same side it already landed on.
1. What is the expected number of rolls?
2. Is there a formula for the expected rolls for an n-sided die?
link to original post
Clarification needed: does it have to be the same side as the previous roll, or do you roll until any number comes up twice (e.g. if you roll 1, 2, 4, 12, 2, you stop at the second 2)?
Quote: ThatDonGuyClarification needed: does it have to be the same side as the previous roll, or do you roll until any number comes up twice (e.g. if you roll 1, 2, 4, 12, 2, you stop at the second 2)?
link to original post
You roll until any number comes up twice. They do not need to occur on consecutive rolls. Be sure to count the final (duplicate) roll. In your example, there were 5 rolls.
p.s. I amended the original wording to be more clear.
Quote: WizardYou roll an icosahedron (20-sided die) until it lands on the same side it already landed on at any point.
1. What is the expected number of rolls, including the ending roll?
2. Is there a formula for the expected rolls for an n-sided die?
link to original post
For N-sided dice from 2 to 20, I get these fractions:
2: 5 / 2
3: 26 / 9
4: 103 / 32
5: 2,194 / 625
6: 1,223 / 324
7: 472,730 / 117,649
8: 556,403 / 131,072
9: 21,323,986 / 4,782,969
10: 7,281,587 / 1,562,500
11: 125,858,034,202 / 25,937,424,601
12: 180,451,625 / 35,831,808
13: 121,437,725,363,954 / 23,298,085,122,481
14: 595,953,719,897 / 110,730,297,608
15: 26,649,932,810,926 / 4,805,419,921,875
16: 3,211,211,914,492,699 / 562,949,953,421,312
17: 285,050,975,993,898,158,530 / 48,661,191,875,666,868,481
18: 549,689,343,118,061 / 91,507,169,819,844
19: 640,611,888,918,574,971,191,834 / 104,127,350,297,911,241,532,841
20: 4,027,894,135,040,576,041 / 640,000,000,000,000,000
I am trying to find a formula, but so far it has eluded me
Quote: ThatDonGuy
For N-sided dice from 2 to 20, I get these fractions:
2: 5 / 2
3: 26 / 9
4: 103 / 32
5: 2,194 / 625
6: 1,223 / 324
7: 472,730 / 117,649
8: 556,403 / 131,072
9: 21,323,986 / 4,782,969
10: 7,281,587 / 1,562,500
11: 125,858,034,202 / 25,937,424,601
12: 180,451,625 / 35,831,808
13: 121,437,725,363,954 / 23,298,085,122,481
14: 595,953,719,897 / 110,730,297,608
15: 26,649,932,810,926 / 4,805,419,921,875
16: 3,211,211,914,492,699 / 562,949,953,421,312
17: 285,050,975,993,898,158,530 / 48,661,191,875,666,868,481
18: 549,689,343,118,061 / 91,507,169,819,844
19: 640,611,888,918,574,971,191,834 / 104,127,350,297,911,241,532,841
20: 4,027,894,135,040,576,041 / 640,000,000,000,000,000
I am trying to find a formula, but so far it has eluded me
link to original post
I agree with your values for 6- and 20-sided dice, the only ones I worked out that you did. For a 100-sided die, I get 13.20996063.
I too have failed at finding a simple formula for even an estimate. It came to me later this is similar to the common birthday problem. As far as I know, nobody has come up with a handy formula for the probability of a common birthday among n people.
- On first count, both players play TWO items, one with each hand.
- On a second count, both players pull back one item, thus playing the other
- Standard rules are then followed to determine the winner.
I'd like to start by stating that I think nobody should ever play the same symbol on both hands, but welcome arguments to the contrary.
Second, let's suppose after the first count the symbols are:
- Player 1: Rock, Paper
- Player 2: Rock, Scissors
The question for the forum is what should each player play at this point?
Quote: WizardI'd like to start by stating that I think nobody should ever play the same symbol on both hands, but welcome arguments to the contrary.
Second, let's suppose after the first count the symbols are:
- Player 1: Rock, Paper
- Player 2: Rock, Scissors
The question for the forum is what should each player play at this point?
link to original post
Your first statement is correct - if you play the same symbol on both hands, your opponent now knows what your will play will be, and act accordingly.
Assuming a Nash equilibruim is in order:
Let p be the probability that P1 plays rock
P(P2 plays rock) = p x 0 + (1-p) x 1 = 1 - p
P(P2 plays scissors) = p x 1 + (1-p) x (-1) = 2p - 1
These are equal when 1 - p = 2p - 1 -> p = 2/3
Player 1 plays rock 2/3 of the time
Let q be the probability that P2 plays rock
P(P1 plays rock) = q x 0 + (1-q) x (-1) = q - 1
P(P2 plays paper) = q x (-1) + (1-q) x 1 = 1 - 2q
These are equal when 1 - 2q = q - 1 -> q = 2/3
Player 2 also plays rock 2/3 of the time
Quote: ThatDonGuy
Assuming a Nash equilibruim is in order:
Let p be the probability that P1 plays rock
P(P2 plays rock) = p x 0 + (1-p) x 1 = 1 - p
P(P2 plays scissors) = p x 1 + (1-p) x (-1) = 2p - 1
These are equal when 1 - p = 2p - 1 -> p = 2/3
Player 1 plays rock 2/3 of the time
Let q be the probability that P2 plays rock
P(P1 plays rock) = q x 0 + (1-q) x (-1) = q - 1
P(P2 plays paper) = q x (-1) + (1-q) x 1 = 1 - 2q
These are equal when 1 - 2q = q - 1 -> q = 2/3
Player 2 also plays rock 2/3 of the time
link to original post
I agree!
Quote: Wizard
I too have failed at finding a simple formula for even an estimate. It came to me later this is similar to the common birthday problem. As far as I know, nobody has come up with a handy formula for the probability of a common birthday among n people.
link to original post
I thought I had one for a minute, but it turned out to be wrong...anyway, I do know that the N-sided die solution is
2
+ (N-1) / N
+ (N-1) / N x (N-2) / N
+ (N-1) / N x (N-2) / N x (N-3) / N
+ ...
+ (N-1) / N x (N-2) / N x (N-3) / N + ... + 2 / N
+ (N-1) / N x (N-2) / N x (N-3) / N + ... + 2 / N + 1 / N
For a minute, I thought that, since this can be rewritten as:
2
+ (N-1)! / (N-2)! x N^(N-2) / N^(N-1)
+ (N-1)! / (N-3)! x N^(N-3) / N^(N-1)
+ ...
+ (N-1)! / 0! x N^0 / N^(N-1)
= 2 + (N-1)! / N^(N-1) x (N^(N-2) / (N-2)! + N^(N-3) / (N-3)! + ... + N^1 / 1! + N^0 / 0!)
and N^(N-2) / (N-2)! + N^(N-3) / (N-3)! + ... + N^1 / 1! + N^0 / 0! are the first N-1 terms of the Taylor expansion of e^N,
then the solution should be approximately 2 + (N-1)! e^N / N^(N-1), but it's not close.
Quote: WizardEpisode 1 of season 2 of The Squid Game features a variant of rock-paper-scissors. Here are the rules:
- On first count, both players play TWO items, one with each hand.
- On a second count, both players pull back one item, thus playing the other
- Standard rules are then followed to determine the winner.
link to original post
In case my rules weren't clear, I stumbled upon this amusing video by the "recruiter" in the Squid Games explaining it.
Direct: https://www.youtube.com/watch?v=Q4auMJndpuI
However, the advice given in the video is WRONG! The example starts at the 0:56 point, which goes as follows in the first stage:
Triangle: paper, scissors
Circle: paper, rock
The Recruiter says Triangle should play paper, because he will either tie or win. The alternative will lead to lose or win.
However, if Circle predicted this, he would player paper as well, leading to a tie. Circle would prefer a tie, since he is at a disadvantage at this point, with 1 way, 1 to tie and 2 ways to lose.
As was discussed earlier in the thread with a different example:
- Triangle should pick rock with probability 1/3 and paper 2/3.
- Circle should pick scissors with probability 1/3 and paper 2/3.
This is an ideal problem for a Poisson integration and the application of the very useful property that the expected number of trials for an event to happen is equal to the sum of the probabilities of the event never happening over all timeQuote: WizardQuote: ThatDonGuy
For N-sided dice from 2 to 20, I get these fractions:
2: 5 / 2
3: 26 / 9
4: 103 / 32
5: 2,194 / 625
6: 1,223 / 324
7: 472,730 / 117,649
8: 556,403 / 131,072
9: 21,323,986 / 4,782,969
10: 7,281,587 / 1,562,500
11: 125,858,034,202 / 25,937,424,601
12: 180,451,625 / 35,831,808
13: 121,437,725,363,954 / 23,298,085,122,481
14: 595,953,719,897 / 110,730,297,608
15: 26,649,932,810,926 / 4,805,419,921,875
16: 3,211,211,914,492,699 / 562,949,953,421,312
17: 285,050,975,993,898,158,530 / 48,661,191,875,666,868,481
18: 549,689,343,118,061 / 91,507,169,819,844
19: 640,611,888,918,574,971,191,834 / 104,127,350,297,911,241,532,841
20: 4,027,894,135,040,576,041 / 640,000,000,000,000,000
I am trying to find a formula, but so far it has eluded me
link to original post
I agree with your values for 6- and 20-sided dice, the only ones I worked out that you did. For a 100-sided die, I get 13.20996063.
I too have failed at finding a simple formula for even an estimate. It came to me later this is similar to the common birthday problem.
link to original post
For an n-sided die, the expected number of rolls to hit any side twice is the integral from zero to infinity of:
(((x/n)+ 1) / e^(x/n))^n dx
So, for example, the expected number of rolls to hit any side twice for a 200-sided die is the integral from zero to infinity of
(((x/200)+ 1) / e^(x/200))^200 dx
Which evaluates to ~18.398 rolls. I can post the exact answer but it's a very long fraction
The Poisson terms of the aforementioned formula can be easily expanded to calculate the expected number of rolls to hit any side k times. For instance, the expected number of rolls to hit any side five times for a 200-side die is the integral from zero to infinity of :
(((x/200)^4/4! + (x/200)^3/3! + (x/200)^2/2 + (x/200) + 1) / e^(x/200))^200 dx
A quite accurate estimate is: 1 - ((365.5 - n/2)/365)^n. Accurate to well within 1/2 percent relative for all nQuote: WizardAs far as I know, nobody has come up with a handy formula for the probability of a common birthday among n people.
link to original post
You have 50 red balls and 50 blue balls and two urns. Call them Urn A and Urn B.
You put the balls in to the urns in any combination, Maybe you want to put all the red balls in Urn A and all the blue balls in Urn B, or vice versa. Maybe you want to put all the balls in Urn B. Whatever!
Your friend will choose an urn at random and pull only one ball at random from that urn, if there is one in there. If there is no ball, he doesn't get another go.
If he pulls a red ball he wins a prize, which he will share with you.
How can you best distribute the balls to maximise his probability of winning, without any collusion. What is that probability and why.
Prove it.
You can show it's a local maximum by considering Urn A has r reds and b blues.
Pr = 1/2 ( r/(r+b) + (50-r)/(100-r-b) )
When r=1 this is 1/2 (1/1 + 49/99). Adding any blues makes 1/1 turn into 1/2 (or smaller) whereas 49/99 only turns into 49/98 etc. The difference lost between 1/1 and 1/2 cannot be made up by 49/98 etc. This actually gets to a mirror result of r=49,b=50.
Similar there is little point in having more reds as 48/98 is smaller than 49/99.
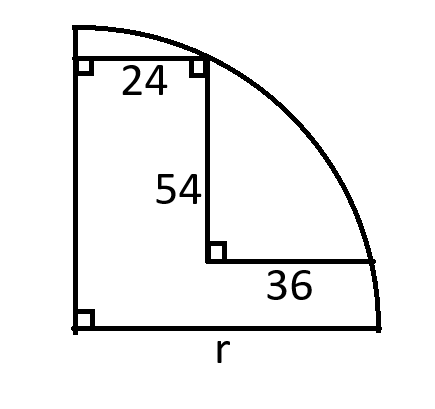
The following three segments are inscribed in a quarter-circle of radius r. Find r.
Quote: Wizard
The following three segments are inscribed in a quarter-circle of radius r. Find r.
link to original post
Let x be the distance from the line segment of 36 to the bottom of the quarter-circle.
Pythagorean Theorem: r^2 = 60^2 + x^2 = 24^2 + (54 + x)^2
x = 1, so r = sqrt(60^2 + 1) = sqrt(3601)
Quote: ThatDonGuy
Let x be the distance from the line segment of 36 to the bottom of the quarter-circle.
Pythagorean Theorem: r^2 = 60^2 + x^2 = 24^2 + (54 + x)^2
x = 1, so r = sqrt(60^2 + 1) = sqrt(3601)
link to original post
I agree!
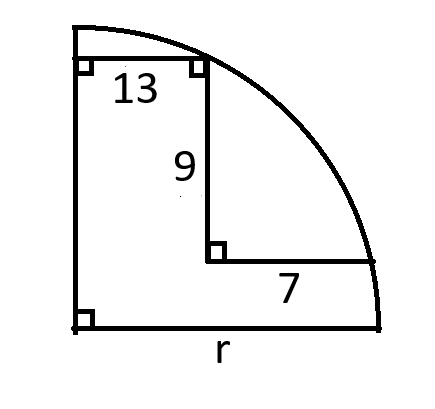
Here is another set of numbers where the math is cleaner.
Solution:
Let x be the vertical distance from the right hand end of the seven unit line to the horizontal radius line.
r² = 20² + x²
= x² + 400
Also, r² = 13² + (x + 9)²
= x² + 18x + 250
By simultaneous equations:
x² + 400 = x² + 18x + 250
x = 50/6
By deduction:
r² = 20² + (50/6)²
r = 21.666
Quote: davethebuilder
Solution:
Let x be the vertical distance from the right hand end of the seven unit line to the horizontal radius line.
r² = 20² + x²
= x² + 400
Also, r² = 13² + (x + 9)²
= x² + 18x + 250
By simultaneous equations:
x² + 400 = x² + 18x + 250
x = 50/6
By deduction:
r² = 20² + (50/6)²
r = 21.666
link to original post
I agree!
E.g., the king asks "Does 1 + 1?" The logician would say "Yes," and the politician would say "No." If the lawyer says "Yes," the king would choose the only "No" respondent, and know that he is the politician. If the Lawyer says "No," the king would choose the only "Yes" respondent, knowing he is the Logician.
Quote: JoemanIf all he is worried about is not picking the lawyer, the king could ask just about any question. Since the logician's and politician's answers would always be opposite, he would choose the one whose answer differs from the other two.
E.g., the king asks "Does 1 + 1?" The logician would say "Yes," and the politician would say "No." If the lawyer says "Yes," the king would choose the only "No" respondent, and know that he is the politician. If the Lawyer says "No," the king would choose the only "Yes" respondent, knowing he is the Logician.
link to original post
Yes, I agree.


