Poll
 | 25 votes (49.01%) | ||
 | 16 votes (31.37%) | ||
 | 7 votes (13.72%) | ||
 | 4 votes (7.84%) | ||
 | 12 votes (23.52%) | ||
 | 3 votes (5.88%) | ||
 | 6 votes (11.76%) | ||
 | 5 votes (9.8%) | ||
 | 12 votes (23.52%) | ||
 | 10 votes (19.6%) |
51 members have voted
Quote: WizardTrack B. I would have to resort to hand-waving logic to explain why.
Quote: charliepatrickB.
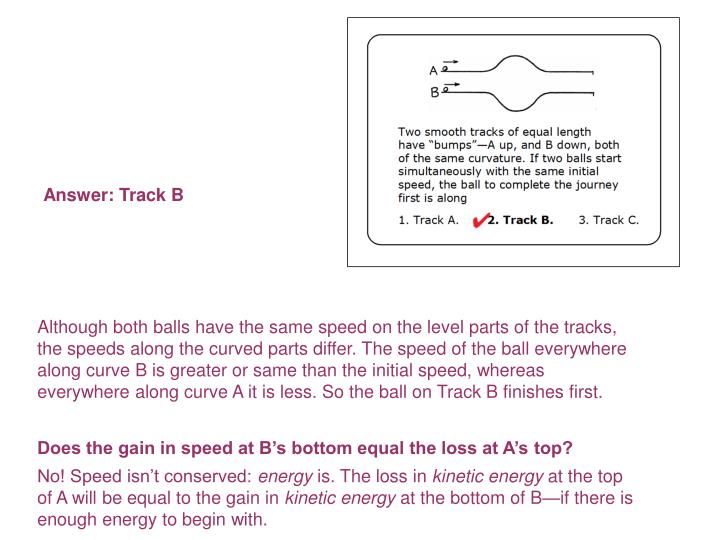
The distance travelled by the two balls is the same, so the only difference is the velocity (or speed). Since there is no force the energy would remain constant, thus as ball A rises, it gains potential energy so will lose kinetic energy, thus it will slow down. For ball B, it loses potential energy so gains kinetic energy, thus it speeds up down into the valley. So at the mid point ball A is going slower than ball B, hence ball B will get to the finish earlier.
An easier way to think of it is if a driver of a car goes up a hill they tend to slow down and then speed up downhill.
Quote: acesideI choose answer number 2, because I calculated the traveling times for the two cases.
On another note, this problem is related to Brachistochrone curve, the track for the least possible traveling time.
Quote: ThatDonGuyI've seen this one before somewhere...
When I first saw it, I answered, "3, since the amount that it goes up equals the amount that it goes down in both cases."
However, I am pretty sure the answer is 2, because the velocity both when it starts the upward path and when it starts the downward path is greater in B than in A, so while the final velocities may be the same, the time it takes is less in B.
Quote: gordonm888I am a physicist, so I had better get this one correct.
The answer is B. Both courses will result in the same final velocity, which is equal to the initial velocity in this frictionless example.. But in course A the ball is decelerated then reaccelerated to the original speed, whereas in B the ball is accelerated to a higher speed and then decelerated to a lower speed. The integral over speed = v(t) will yield a higher average speed for course B, and thus the Ball in B will arrive first.
Note, I am assuming the speeds are all low relative to the speed of light and thus relativistic concerns are not a factor, lol.
Quote: ChesterDog
While A and B are traveling on the flat regions, they are both going at the same speed. So, A's and B's flat regions' times would be equal. So, we can ignore the flat regions.
To make the problem an "easy" problem, realize that a movie of the experiment would be almost the same going forward and backward. The only difference would be the backward movie would be a mirror image of the forward movie.
In other words, the time for B to go down the hill equals the time for B to go up the hill. And the same applies for A. But of course, B takes less time to go down the hill than A does to go up the hill because B is speeding up while A is slowing down.
So, B would definitely complete the course before A.
Correct!!
-----------------------------------------

A bag contains 100 marbles, and each marble is one of three different colors. If you were to draw three marbles at random, the probability that you would get one of each color is exactly 20 percent.
How many marbles of each color are in the bag?
[Note that figuring out if this is done with or without replacement is part of the puzzle.]
Without replacement. If correct I'll show the method
My method was essentially the same. Though I started evaluating the triples from the largest possible multiple of eleven (77) which I thought was easier since the remainders are smaller that wayQuote: charliepatrickThe logic is that suppose the chances of picking each colour was x,y and z. Then x+y+z=1 (i.e. each ball is one of the three colours). The other thing is that you can get a correct result from any of six ways to X followed by Y followed by Z.(XYZ, XZY, YXZ etc.) The chances of one of these ways is the number X * the number of Y * the number of Z divided by (either 100*100*100 or 100*99*98). Thus any order has the same chance, so each one must have a probability of 1/30. This is impossible with integers with replacement (as the numerator would have to be 333333. 1/3, thus the numerator is 100*99*98/30=has to be 32340. So you have to find X,Y,Z such that X+Y+Z=100 and XYZ=32340. The factors of this are 2 2 3 5 7 7 11, so one of the numbers is either 11,22,33,44 etc. Looking at using the factors of 7: 11 49 60 thru 55 49 12 don't work, 11 35 84!, 22 35 42 !, 33 35 28 !, 44 35 21 works. So the answer is 44 35 21.
link to original post
Quote: charliepatrickThe logic is that suppose the chances of picking each colour was x,y and z. Then x+y+z=1 (i.e. each ball is one of the three colours). The other thing is that you can get a correct result from any of six ways to X followed by Y followed by Z.(XYZ, XZY, YXZ etc.) The chances of one of these ways is the number X * the number of Y * the number of Z divided by (either 100*100*100 or 100*99*98). Thus any order has the same chance, so each one must have a probability of 1/30. This is impossible with integers with replacement (as the numerator would have to be 333333. 1/3, thus the numerator is 100*99*98/30=has to be 32340. So you have to find X,Y,Z such that X+Y+Z=100 and XYZ=32340. The factors of this are 2 2 3 5 7 7 11, so one of the numbers is either 11,22,33,44 etc. Looking at using the factors of 7: 11 49 60 thru 55 49 12 don't work, 11 35 84!, 22 35 42 !, 33 35 28 !, 44 35 21 works. So the answer is 44 35 21.
link to original post
What I am wondering about is this.
After eliminating the replacement scenario, we have two equations with three unknowns:
k + m + n = 100, k,m,n are integers
and
6* (k*m*n) / (100*99*98) = 0.2.
Now its always possible that there might be no integer solutions. But shouldn't it also be possible for a set of equations like this that there are two or more solutions? For totals different than 100, or fractions different than 0.2, are there examples where there are more than one set of three integers that are solutions? If not, why are two equations with three unknowns limited to a maximum of one solution?
Quote: Ace244, 35, 21
Without replacement. If correct I'll show the method
Quote: charliepatrickThe logic is that suppose the chances of picking each colour was x,y and z. Then x+y+z=1 (i.e. each ball is one of the three colours). The other thing is that you can get a correct result from any of six ways to X followed by Y followed by Z.(XYZ, XZY, YXZ etc.) The chances of one of these ways is the number X * the number of Y * the number of Z divided by (either 100*100*100 or 100*99*98). Thus any order has the same chance, so each one must have a probability of 1/30. This is impossible with integers with replacement (as the numerator would have to be 333333. 1/3, thus the numerator is 100*99*98/30=has to be 32340. So you have to find X,Y,Z such that X+Y+Z=100 and XYZ=32340. The factors of this are 2 2 3 5 7 7 11, so one of the numbers is either 11,22,33,44 etc. Looking at using the factors of 7: 11 49 60 thru 55 49 12 don't work, 11 35 84!, 22 35 42 !, 33 35 28 !, 44 35 21 works. So the answer is 44 35 21.
Correct!!
Excellent work.
Next, there were six different ways to draw three marbles of different colors:
red, green, blue
red, blue, green
green, red, blue
green, blue, red
blue, red, green
blue, green, red
Moreover, each of these six permutations was equally likely. For example, the probability of first drawing a red was r/100. The probability of then drawing a green was g/99, since there were now only 99 marbles remaining in the bag. Finally, the probability of drawing a blue was then b/98. That meant the probability of each permutation was rgb/(100·99·98). To find the probability of drawing three different colors, you simply had to add these six probabilities together, giving you 6·rgb/(100·99·98), or rgb/161,700.
Finally, you were told that this probability equaled 20 percent, which told you that rgb/161,700 = 1/5. Multiplying both sides by 161,700 gave you rgb = 32,340.
At this point, you were looking for three whole numbers whose sum was 100 and whose product was 32,340. For some solvers, It was helpful to write 32,340 as a product of primes: 22·3·5·72·11. From here, you could try out different ways of separating these primes into three larger factors. For example, suppose these factors were 22·5, 3·7 and 7·11. Their product was indeed 32,340 (no surprise), but their sum was 20+21+77, or 118. Because the sum was not 100, these factors were not the correct values of r, g and b.
Many solvers found a trio of factors that worked by trial and error. Pramod Mathai applied Maclaurin’s inequality to show that r, g and b were all at least 20 and at most 50, which significantly reduced the number of possibilities to check.
Matt Enlow, the puzzle’s author, restricted this range a little further. Suppose the largest of the three factors was 49. To maximize their product, the other two factors (which added up to 51) would have been 25 and 26. However, 25·26·49 = 31,850, a few hundred shy of the required product — 32,340. That meant all three factors were at most 48, and that the primes 7, 7 and 11 all had to occur in different factors. In the end, the unique trio of factors was 21, 35 and 44.
By the way, another way to interpret the problem was to place each marble back in the bag after is was selected (known as picking with replacement). Again, you needed r+g+b to equal 100. However, this time, the product rgb had to equal approximately 33,333.33. Since there was no way to multiply integers and get a non-integer product, picking with replacement was not an option.
-------------------------------------------------------
:strip_icc()/pic6529597.png)
21 35 44
I'll explain my solution, if I'm right.
Quote: gordonm888What I am wondering about is this.
After eliminating the replacement scenario, we have two equations with three unknowns:
k + m + n = 100, k,m,n are integers
and
6* (k*m*n) / (100*99*98) = 0.2.
Now its always possible that there might be no integer solutions. But shouldn't it also be possible for a set of equations like this that there are two or more solutions? For totals different than 100, or fractions different than 0.2, are there examples where there are more than one set of three integers that are solutions? If not, why are two equations with three unknowns limited to a maximum of one solution?
link to original post
Yes.
Change 100 to 14 and 0.2 to 18/91:
6 * (8 * 3 * 3) / (14 * 13 * 12) = 18/91
6 * (6 * 6 * 2) / (14 * 13 * 12) = 18/91
For that matter, change 100 to 14 and 0.2 to 10/91:
6 * (10 * 2 * 2) / (14 * 13 * 12) = 10/91
6 * (8 * 5 * 1) / (14 * 13 * 12) = 10/91
Rephrase the problem: you are looking for positive values p and q (where p is the total number of marbles and q the probability) such that a + b + c = p and 6 abc / (p (p-1) (p-2)) = q for multiple unordered triples (a, b, c).
The second equation can be rewritten as abc = p (p-1) (p-2) q / 6.
One factor that limits the solutions in this particular case is, q is a probability, so q < 1 is a boundary condition.
Here are the only pairs of (total number of balls, probability of getting one of each) that have more than one solution:
Edit - probabilities have been corrected
13, 18/143 has (9, 2, 2) and (6, 6, 1)
14, 10/91 has (10, 2, 2) and (8, 5, 1)
14, 18/91 has (8, 3, 3) and (6, 6, 2)
16, 9/56 has (10, 3, 3) and (9, 5, 2)
17, 18/85 has (9, 4, 4) and (8, 6, 3)
19, 48/323 has (12, 4, 3) and (9, 8, 2)
20, 3/38 has (15, 3, 2) and (10, 9, 1)
21, 48/665 has (16, 3, 2) and (12, 8, 1)
21, 12/95 has (14, 4, 3) and (12, 7, 2)
21, 24/133 has (12, 5, 4) and (10, 8, 3)
22, 18/77 has (10, 6, 6) and (9, 8, 5)
23, 360/1771 has (12, 6, 5) and (10, 9, 4)
24, 30/253 has (16, 5, 3) and (12, 10, 2)
25, 18/115 has (15, 6, 4) and (12, 10, 3)
26, 63/1300 has (21, 3, 2) and (18, 7, 1)
26, 27/260 has (18, 5, 3) and (15, 9, 2)
26, 36/325 has (18, 4, 4) and (12, 12, 2)
27, 56/975 has (21, 4, 2) and (14, 12, 1)
28, 44/819 has (22, 4, 2) and (16, 11, 1)
28, 80/819 has (20, 4, 4) and (16, 10, 2)
29, 20/203 has (20, 6, 3) and (15, 12, 2)
31, 40/899 has (25, 4, 2) and (20, 10, 1)
31, 45/899 has (25, 3, 3) and (15, 15, 1)
32, 117/2480 has (26, 3, 3) and (18, 13, 1)
34, 63/1496 has (28, 3, 3) and (21, 12, 1)
34, 135/2992 has (27, 5, 2) and (18, 15, 1)
35, 8/187 has (28, 5, 2) and (20, 14, 1)
39, 297/9139 has (33, 3, 3) and (27, 11, 1)
42, 36/1435 has (36, 4, 2) and (32, 9, 1)
43, 360/12341 has (36, 5, 2) and (30, 12, 1)
That said, I tested all 3^7=2187 possible combinations of the prime factors and can confirm that 21, 35, and 44 is the only possible answer.

To celebrate Thanksgiving, you and 19 mathematicians are seated at a circular table. Everyone at the table would like a helping of cranberry sauce, which happens to be in front of you at the moment.
Instead of passing the sauce around in a circle, you decide to pass it randomly to the person seated directly to your left or to your right. They then do the same, passing it randomly either to the person to their left or right. This continues until everyone has, at some point, received the cranberry sauce.
Of the 20 people in the circle, who has the greatest chance of being the last to receive the cranberry sauce?
Quote: ThatDonGuy
Rephrase the problem: you are looking for positive values p and q (where p is the total number of marbles and q the probability) such that a + b + c = p and 6 abc / (p (p-1) (p-2)) = q for multiple unordered triples (a, b, c).
The second equation can be rewritten as abc = p (p-1) (p-2) q / 6.
link to original post
Thank you for your generous answer.
I am running off to my Thanksgiving feast and don't have the time to study your results closely. I am wondering what the properties are of 91 that it (or its multiples) are in the denominator of every q.
Quote: gordonm888I am running off to my Thanksgiving feast and don't have the time to study your results closely. I am wondering what the properties are of 91 that it (or its multiples) are in the denominator of every q.
link to original post
That was my mistake - I was assuming p = 14 for each one when I calculated them.
The probabilities have been corrected in my earlier post.
I also just realized that those may not be all of the answers where the probability < 1.
Quote: ThatDonGuy
Yes.
Change 100 to 14 and 0.2 to 18/91:
6 * (8 * 3 * 3) / (14 * 13 * 12) = 18/91
6 * (6 * 6 * 2) / (14 * 13 * 12) = 18/91
For that matter, change 100 to 14 and 0.2 to 10/91:
6 * (10 * 2 * 2) / (14 * 13 * 12) = 10/91
6 * (8 * 5 * 1) / (14 * 13 * 12) = 10/91
Rephrase the problem: you are looking for positive values p and q (where p is the total number of marbles and q the probability) such that a + b + c = p and 6 abc / (p (p-1) (p-2)) = q for multiple unordered triples (a, b, c).
The second equation can be rewritten as abc = p (p-1) (p-2) q / 6.
One factor that limits the solutions in this particular case is, q is a probability, so q < 1 is a boundary condition.
Here are the only pairs of (total number of balls, probability of getting one of each) that have more than one solution:
Edit - probabilities have been corrected
13, 18/143 has (9, 2, 2) and (6, 6, 1)
14, 10/91 has (10, 2, 2) and (8, 5, 1)
14, 18/91 has (8, 3, 3) and (6, 6, 2)
16, 9/56 has (10, 3, 3) and (9, 5, 2)
17, 18/85 has (9, 4, 4) and (8, 6, 3)
19, 48/323 has (12, 4, 3) and (9, 8, 2)
20, 3/38 has (15, 3, 2) and (10, 9, 1)
21, 48/665 has (16, 3, 2) and (12, 8, 1)
21, 12/95 has (14, 4, 3) and (12, 7, 2)
21, 24/133 has (12, 5, 4) and (10, 8, 3)
22, 18/77 has (10, 6, 6) and (9, 8, 5)
23, 360/1771 has (12, 6, 5) and (10, 9, 4)
24, 30/253 has (16, 5, 3) and (12, 10, 2)
25, 18/115 has (15, 6, 4) and (12, 10, 3)
26, 63/1300 has (21, 3, 2) and (18, 7, 1)
26, 27/260 has (18, 5, 3) and (15, 9, 2)
26, 36/325 has (18, 4, 4) and (12, 12, 2)
27, 56/975 has (21, 4, 2) and (14, 12, 1)
28, 44/819 has (22, 4, 2) and (16, 11, 1)
28, 80/819 has (20, 4, 4) and (16, 10, 2)
29, 20/203 has (20, 6, 3) and (15, 12, 2)
31, 40/899 has (25, 4, 2) and (20, 10, 1)
31, 45/899 has (25, 3, 3) and (15, 15, 1)
32, 117/2480 has (26, 3, 3) and (18, 13, 1)
34, 63/1496 has (28, 3, 3) and (21, 12, 1)
34, 135/2992 has (27, 5, 2) and (18, 15, 1)
35, 8/187 has (28, 5, 2) and (20, 14, 1)
39, 297/9139 has (33, 3, 3) and (27, 11, 1)
42, 36/1435 has (36, 4, 2) and (32, 9, 1)
43, 360/12341 has (36, 5, 2) and (30, 12, 1)
link to original post
In ThatDonGuy's table, the denominator of the probability q tends to be the product of the two (or even three) largest prime factors of
p*(p-1)*(p-2)
For example, when p= 19, the value of q = 48/323. Note that 323 = p *(p-2) or 19*17. This makes perfect sense as the solutions must generally require small prime factors -with no large prime factors - for p*(p-1)*(p-2)*q/6. So the q is a fraction that has a denominator that wipes out the large prime factors and replaces them with a numerator that has many small prime factors.
Note the last row on the list, for p =43. The product p*(p-1)*(p-2) is 43*42*41 and the probability q is 360/12341 and 12341 =43*41*7. Thus, the value of q that works for p=43 is one for which the denominator eliminates the large prime factors of p*(p-1)*(p-2) and introduces a set of small prime factors with its numerator.
Quote: GialmereHappy Thanksgiving!!Of the 20 people in the circle, who has the greatest chance of being the last to receive the cranberry sauce?
link to original post
Happy Thanksgiving to you too and all the math geniuses of the forum!
My solution is I answered this for the 3, 4, and 5-person case and all of them showed, hopefully correctly, that everyone had an equal chance of being last. I can explain how I did those cases on request, but I thought of it like a coin flipping game where the goal was to keep flipping until you won $x or lost $y.
Extending the logic for those three cases, I say regardless of the number of mathematicians, everyone will have an equal chance to be last.
Quote: WizardHappy Thanksgiving to you too and all the math geniuses of the forum!
I think everyone has an equal chance to be last.
My solution is I answered this for the 3, 4, and 5-person case and all of them showed, hopefully correctly, that everyone had an equal chance of being last. I can explain how I did those cases on request, but I thought of it like a coin flipping game where the goal was to keep flipping until you won $x or lost $y.
Extending the logic for those three cases, I say regardless of the number of mathematicians, everyone will have an equal chance to be last.
Correct!!
Very good.
Yes. It's a surprisingly fair way to determine who gets stuck with the last helping (if you don't mind spending hours at the table passing food around).
------------------------------------------------------------
Bad Thanksgiving jokes...
Quote: GialmereVery good.
Yes. It's a surprisingly fair way to determine who gets stuck with the last helping (if you don't mind spending hours at the table passing food around).
I'm working on a better way to state my answer. Here is what I'm trying to prove:
Consider a gambler playing a 50/50 game that pays even money. Gambler bets one unit at a time. I submit these chances are the same:
1. Gambler wins $(a+b) before losing one unit.
2. Gambler loses $a and wins $b in the same session, before exceeding either marker.
It's seems intuitive to me, but working how to put an argument in words.
Quote:Bad Thanksgiving jokes...
To get to the other sides.but some people say that’s irrational.Scared the hell out of everyone else in the grocery store.
link to original post
Those were so bad they're good!
Quote: gordonm888
In ThatDonGuy's table, the denominator of the probability q tends to be the product of the two (or even three) largest prime factors of
p*(p-1)*(p-2)
For example, when p= 19, the value of q = 48/323. Note that 323 = p *(p-2) or 19*17. This makes perfect sense as the solutions must generally require small prime factors -with no large prime factors - for p*(p-1)*(p-2)*q/6. So the q is a fraction that has a denominator that wipes out the large prime factors and replaces them with a numerator that has many small prime factors.
Note the last row on the list, for p =43. The product p*(p-1)*(p-2) is 43*42*41 and the probability q is 360/12341 and 12341 =43*41*7. Thus, the value of q that works for p=43 is one for which the denominator eliminates the large prime factors of p*(p-1)*(p-2) and introduces a set of small prime factors with its numerator.
link to original post
There's a reason they "tend to be" that way.
Let a, b, c be the three numbers, with p = a + b + c and q = abc.
The probability of drawing colors a, b, c, in that order is a / p * b / (p - 1) * c / (p - 2) = q / (p (p-1)(p-2)).
Since the order of colors is arbitrary, and there are six orders, the overall probability is 6q / (p (p-1) (p-2)).
I have also discovered the following:
39 balls and probability 1,200/9,139 has 3 solutions:
25, 8, 6
24, 10, 5
20, 15, 4
118 balls and probability 3,150/22,243 has 4 solutions:
72, 25, 21
70, 30, 18
63, 40, 15
54, 50, 14
185 balls and probability 4,158/51,911 has 5 solutions:
135, 28, 22
132, 35, 18
126, 44, 15
110, 63, 12
90, 84, 11
400 balls and probability 1,512/18,905 has 6 solutions:
288, 70, 42
280, 84, 36
270, 98, 32
252, 120, 28
245, 128, 27
196, 180, 24
511 balls and probability 56,160/631,669 has 7 solutions:
364, 75, 72
360, 91, 60
336, 130, 45
325, 144, 42
315, 156, 40
280, 195, 36
260, 216, 35
Quote: GialmereQuote: WizardHappy Thanksgiving to you too and all the math geniuses of the forum!
I think everyone has an equal chance to be last.
My solution is I answered this for the 3, 4, and 5-person case and all of them showed, hopefully correctly, that everyone had an equal chance of being last. I can explain how I did those cases on request, but I thought of it like a coin flipping game where the goal was to keep flipping until you won $x or lost $y.
Extending the logic for those three cases, I say regardless of the number of mathematicians, everyone will have an equal chance to be last.
Correct!!
Very good.
Yes. It's a surprisingly fair way to determine who gets stuck with the last helping (if you don't mind spending hours at the table passing food around).
How can the first person possibly be the last person - unless you are not counting the first person as having the cranberries until (and unless) they are passed to that person from someone else - i.e. the problem asks for the last person to have the cranberries passed to them?
Quote: ThatDonGuyQuote: gordonm888
In ThatDonGuy's table, the denominator of the probability q tends to be the product of the two (or even three) largest prime factors of
p*(p-1)*(p-2)
For example, when p= 19, the value of q = 48/323. Note that 323 = p *(p-2) or 19*17. This makes perfect sense as the solutions must generally require small prime factors -with no large prime factors - for p*(p-1)*(p-2)*q/6. So the q is a fraction that has a denominator that wipes out the large prime factors and replaces them with a numerator that has many small prime factors.
Note the last row on the list, for p =43. The product p*(p-1)*(p-2) is 43*42*41 and the probability q is 360/12341 and 12341 =43*41*7. Thus, the value of q that works for p=43 is one for which the denominator eliminates the large prime factors of p*(p-1)*(p-2) and introduces a set of small prime factors with its numerator.
link to original post
There's a reason they "tend to be" that way.
Let a, b, c be the three numbers, with p = a + b + c and q = abc.
The probability of drawing colors a, b, c, in that order is a / p * b / (p - 1) * c / (p - 2) = q / (p (p-1)(p-2)).
Since the order of colors is arbitrary, and there are six orders, the overall probability is 6q / (p (p-1) (p-2)).
I have also discovered the following:
39 balls and probability 1,200/9,139 has 3 solutions:
25, 8, 6
24, 10, 5
20, 15, 4
118 balls and probability 3,150/22,243 has 4 solutions:
72, 25, 21
70, 30, 18
63, 40, 15
54, 50, 14
185 balls and probability 4,158/51,911 has 5 solutions:
135, 28, 22
132, 35, 18
126, 44, 15
110, 63, 12
90, 84, 11
400 balls and probability 1,512/18,905 has 6 solutions:
288, 70, 42
280, 84, 36
270, 98, 32
252, 120, 28
245, 128, 27
196, 180, 24
511 balls and probability 56,160/631,669 has 7 solutions:
364, 75, 72
360, 91, 60
336, 130, 45
325, 144, 42
315, 156, 40
280, 195, 36
260, 216, 35
link to original post
Yes, I have been abstracting this "balls with three colors" problem and thinking of it as:
Can multiple partitions of a number, p, with the same length (in this case, length 3) have the identical set of prime factors? And if so, under what circumstances?
or
What are the characteristics of a set of prime numbers (which may include multiples) that can generate multiple partitions of the same length of the same number?
Stated like that, the problem is no longer one of probability, or balls of different colors. Thus, it was disturbing me that the existence of partitions of the same length with the same set of prime factors might depend upon the values of (p-1) and (p-2). What I was struggling to say is that the denominator of q decouples the partitions of length 3 from the prime factors of the product p*(p-1)*(p-2) and that it is the numerator of q that is central in defining the prime factor set that generates multiple partitions of p of the same length. I agree that when viewed in the framework of the original problem, my previous post was childishly obvious. I had lost myself down the rabbit hole of partitions and their sets of prime factors.
Your results continue to be fascinating. I assume those examples above are the smallest numbers that have n solutions, n = 3-7?
EDIT: A better way to say all this is that your triplets have the same sum and the same product!! Example:
25+8+ 6 = 24+10+5 = 20+15+4
and,
25*8*6 = 24*10*5 = 20*15*4
and its unnecessary to express their common products as a fraction q of p*(p-1)*(p-2)/6.
See: OEIS A103277
"Smallest i such that there exists j such that i = x + y + z, j = x*y*z has exactly n solutions in positive integers x <= y <= z".
3, 13, 39, 118, 185, 400, 511, 1022, 1287, 2574, 4279, 8558, 11777, 24377, 23554, 46111, 99085, 165490
Edit: Interesting that it does not monotonically increase. the 15th term, 23554 is smaller than the 14th term.
Quote: GialmereQuote: WizardHappy Thanksgiving to you too and all the math geniuses of the forum!
I think everyone has an equal chance to be last.
My solution is I answered this for the 3, 4, and 5-person case and all of them showed, hopefully correctly, that everyone had an equal chance of being last. I can explain how I did those cases on request, but I thought of it like a coin flipping game where the goal was to keep flipping until you won $x or lost $y.
Extending the logic for those three cases, I say regardless of the number of mathematicians, everyone will have an equal chance to be last.
Correct!!
Very good.
Yes. It's a surprisingly fair way to determine who gets stuck with the last helping (if you don't mind spending hours at the table passing food around).
link to original post
I am not getting this. What I get is:
If the first person is included in having "touched" the cranberries at the start, then yes, everybody but that person has an equal chance.
If the first person is not included until the cranberries are passed to that person by someone else, then the two people adjacent to the first person are half as likely as the others.
Quote: GialmereHere is a link to the Riddler page containing the official solve (at the bottom). I would just post it here but the rather fun pie chart animations from Twitter will not embed.
link to original post
Thank you. At first I thought that was a "hand waving" kind of proof, but now I can see the logic in it. Here is how I might word it.
Let the starting position be x and y be any other specific person you wish to find the probability of being last.
Eventually, the cranberry sauce will find it's way to someone to either side of y. Then, for y to be last, the sauce must immediately move away from y and eventually get all the way to y's other neighbor, without ever getting to y from the other side. That is 19 steps it must move.
If you have one unit to gamble with on a 50/50 game, your chances of winning n units before losing the one unit is 1/(1+n). I can prove this upon request, but I hope it's obvious.
So, in the case of the sauce moving 18 steps before moving one from the starting point is 1/19.
You can use this logic for anyone at the table.

A man had a 10-gallon keg of wine and a jug. One day, he drew a jugful of wine and then topped off the keg with water. Later on, when the wine and water had gotten thoroughly mixed, he drew another jugful and again topped off the keg with water. The keg then contained equal quantities of wine and water.
What was the capacity of the jug?
Quote: Gialmere...What was the capacity of the jug?
Quote: Gialmere
A man had a 10-gallon keg of wine and a jug. One day, he drew a jugful of wine and then topped off the keg with water. Later on, when the wine and water had gotten thoroughly mixed, he drew another jugful and again topped off the keg with water. The keg then contained equal quantities of wine and water.
What was the capacity of the jug?
link to original post
Let J = volume of the jug.
Now, follow the water. Initially, the volume of water in the wine keg is 0. The first step (removing J wine from the keg) does not change the volume of water in the keg.
The second step adds a volume of J water to the keg.
The third step removes a fraction (J/10) of the previously added water. So (J/10)*J water has been removed.
The last step adds J water to the keg.
So, the water in the jug is now 0 + 0 + J - J2/10 + J which should equal 5 if the volume of water in the keg equals the volume of wine in the keg.
Solve this equation for J:
J2 - 20J + 50 = 0
Add 50 to both sides and manipulating:
J2 - 20J + 100 = 50
(J - 10)2 = 50
J - 10 = +/- 501/2
J = 10 - 501/2 or J = 10 + 501/2
J = 2.92893... or J = 17.0711...
Of course J cannot be greater than 10, so the volume of the jug must be about 2.93 gallons.
Quote: GialmereA man had a 10-gallon keg of wine and a jug. One day, he drew a jugful of wine and then topped off the keg with water. Later on, when the wine and water had gotten thoroughly mixed, he drew another jugful and again topped off the keg with water. The keg then contained equal quantities of wine and water.
What was the capacity of the jug?
link to original post
Let J be the capacity of the jug
At the start, the keg has 10 (gallons) wine and no water
Draw a jug of wine, and replace it with a jug of water:
The keg has 10-J wine and J water
Draw another jug of wine, and replace it with water:
The amount drawn is J (10 - J)/10 = (10 J - J^2) / 10 wine
The amount of wine remaining in the keg is 10 - J - (10 J - J^2) / 10
Since the amount of wine remaining is half the total, 10 - J - (10 J - J^2) / 10 = 5
100 - 10 J - (10 J - J^2) = 50
50 - 20 J + J^2 = 0
J = 10 +/- sqrt(400 - 200) / 2 = 10 +/- 5 sqrt(2)
Since 10 + 5 sqrt(2) is greater than the capacity of the keg, the only solution is 10 - 5 sqrt(2) gallons
Quote: Gialmere...What was the capacity of the jug?
Let j = volume of jug.
Wine after second dipping = wine after first dipping * ratio of wine after first dipping
5 = (10-j)*((10-j)/10)
j^2 - 20j + 50 = 0
j = 10 - 5*sqrt(2)
Quote: ChesterDog
Let J = volume of the jug.
Now, follow the water. Initially, the volume of water in the wine keg is 0. The first step (removing J wine from the keg) does not change the volume of water in the keg.
The second step adds a volume of J water to the keg.
The third step removes a fraction (J/10) of the previously added water. So (J/10)*J water has been removed.
The last step adds J water to the keg.
So, the water in the jug is now 0 + 0 + J - J2/10 + J which should equal 5 if the volume of water in the keg equals the volume of wine in the keg.
Solve this equation for J:
J2 - 20J + 50 = 0
Add 50 to both sides and manipulating:
J2 - 20J + 100 = 50
(J - 10)2 = 50
J - 10 = +/- 501/2
J = 10 - 501/2 or J = 10 + 501/2
J = 2.92893... or J = 17.0711...
Of course J cannot be greater than 10, so the volume of the jug must be about 2.93 gallons.
Quote: ThatDonGuy
Let J be the capacity of the jug
At the start, the keg has 10 (gallons) wine and no water
Draw a jug of wine, and replace it with a jug of water:
The keg has 10-J wine and J water
Draw another jug of wine, and replace it with water:
The amount drawn is J (10 - J)/10 = (10 J - J^2) / 10 wine
The amount of wine remaining in the keg is 10 - J - (10 J - J^2) / 10
Since the amount of wine remaining is half the total, 10 - J - (10 J - J^2) / 10 = 5
100 - 10 J - (10 J - J^2) = 50
50 - 20 J + J^2 = 0
J = 10 +/- sqrt(400 - 200) / 2 = 10 +/- 5 sqrt(2)
Since 10 + 5 sqrt(2) is greater than the capacity of the keg, the only solution is 10 - 5 sqrt(2) gallons
Quote: charliepatrickLet x be the ratio of wine removed into the jug. Then the keg now holds 10*(1-x) wine and 10*x water. So the keg now has a ratio of x water and (1-x) of wine. In the second drawing the ratio of the keg removed is x, so the amount of wine removed is 10*x*(1-x). Thus the total water removed is 10* (x + x*(1-x) ). Since the keg now has equal quantities of wine and water, 2x-x2=1/2. Thus x = ( -2 +/- SQRT(4-2) ) / 2. This gives x=1-SQRT(2)/2, i.e. the keg is about 2.929 gallons.
Quote: WizardI also get 10-5*sqrt(2) =~ 2.9289 gallons
Let j = volume of jug.
Wine after second dipping = wine after first dipping * ratio of wine after first dipping
5 = (10-j)*((10-j)/10)
j^2 - 20j + 50 = 0
j = 10 - 5*sqrt(2)
Correct!!
-----------------------------------------------------------

Quote: WizardCongratulations G! You scored a hat trick in my latest Ask the Wizard column (#357)
Wow! Quite an honor considering that, unlike most of the actual mathematicians here, I'm merely competent at math.
(My actual superpower is running entertaining online contests.)
Cookie Clicker involves (a) clicking on a giant cookie, and (b) constructing buildings to build more cookies; you pay for the buildings with, you guessed it, cookies.
Every time you build a particular building, the cost of building another of that building goes up by 15%.
If you have 400 Idleverses, it costs 22.366 quattrodecillion (i.e. 2.2366 x 1046) cookies to build a 401st one. (Assume that number is exact.)
What is the smallest integer N such that, if you have N Idleverses, it costs you less than one quindecillion (i.e. 1048) cookies to get to 400?
(An Idleverse is a parallel universe where they run "idle games" (games that mainly run in the background) like Cookie Clicker.)
Change the problem to find the smallest N such that it costs less than 100 quattrodecillion (i.e. 1047) to get to 400.

Two information theoreticians, A and B, perform a trick with a shuffled deck of cards, jokers removed. A asks a member of the audience to select five cards at random from the deck. The audience member passes the five cards to A, who examines them, and hands one back. A then arranges the remaining four cards in some way and places them face down, in a neat pile.
B, who has not witnessed these proceedings, then enters the room, looks at the four cards, and determines the missing fifth card, held by the audience member.
How is this trick done?
Note: The only communication between A and B is via the arrangement of the four cards. There is no encoded speech or hand signals or ESP, no bent or marked cards, no clue in the orientation of the pile of four cards...
Quote: GialmereTwo information theoreticians, A and B, perform a trick with a shuffled deck of cards, jokers removed. A asks a member of the audience to select five cards at random from the deck. The audience member passes the five cards to A, who examines them, and hands one back. A then arranges the remaining four cards in some way and places them face down, in a neat pile.
B, who has not witnessed these proceedings, then enters the room, looks at the four cards, and determines the missing fifth card, held by the audience member.
How is this trick done?
Note: The only communication between A and B is via the arrangement of the four cards. There is no encoded speech or hand signals or ESP, no bent or marked cards, no clue in the orientation of the pile of four cards...
link to original post
By the Pigeonhole Principle, at least one suit must have two of the five cards.
Take two cards of the same suit; if the higher rank (assume ace high) is 1-6 higher than the
lower rank, give the higher rank card to the audience member; otherwise, give the lower rank
card back. (i.e. if the hand includes the 2 and 7 of spades, give the 7 back; if it includes
the 2 and 9 of spades, give the 2 back).
Arrange the cards so that the card that was in the original "two cards of the same suit" is
first, then the other three are in an order that indicates a number from 1 to 6. The rankings
of the cards can be first by rank (ace high), then suit (use bridge bidding order; spades,
hearts, diamonds, clubs).
If the cards are A, B, C from lowest to highest, then order ABC represents 1, ACB is 2, BAC is 3,
BCA is 4, CAB is 5, and CBA is 6.
Add the corresponding number to the rank of the first card; if it is more than 13, subtract 13
from the value.
Example:
The five cards are 2, 6, and King of spades, 3 of hearts, and 3 of diamonds
Give the audience member back the 6 of spades, then arrange the cards in this order:
2 of spades, 3 of hearts, King of spades, 3 of diamonds.
The second magician looks at the last three cards and sees that they are in order B, C, A,
which is number 4, so the missing card is the same suit as the first card, and 4 more than
its rank; the (2 + 4) = 6 of spades.
Quote: ThatDonGuy
By the Pigeonhole Principle, at least one suit must have two of the five cards.
Take two cards of the same suit; if the higher rank (assume ace high) is 1-6 higher than the
lower rank, give the higher rank card to the audience member; otherwise, give the lower rank
card back. (i.e. if the hand includes the 2 and 7 of spades, give the 7 back; if it includes
the 2 and 9 of spades, give the 2 back).
Arrange the cards so that the card that was in the original "two cards of the same suit" is
first, then the other three are in an order that indicates a number from 1 to 6. The rankings
of the cards can be first by rank (ace high), then suit (use bridge bidding order; spades,
hearts, diamonds, clubs).
If the cards are A, B, C from lowest to highest, then order ABC represents 1, ACB is 2, BAC is 3,
BCA is 4, CAB is 5, and CBA is 6.
Add the corresponding number to the rank of the first card; if it is more than 13, subtract 13
from the value.
Example:
The five cards are 2, 6, and King of spades, 3 of hearts, and 3 of diamonds
Give the audience member back the 6 of spades, then arrange the cards in this order:
2 of spades, 3 of hearts, King of spades, 3 of diamonds.
The second magician looks at the last three cards and sees that they are in order B, C, A,
which is number 4, so the missing card is the same suit as the first card, and 4 more than
its rank; the (2 + 4) = 6 of spades.
Correct!!
In any group of five cards, there must be at least two of the same suit. A selects one of the cards from a duplicate suit and hands it back to the audience member. The other card (or one of the others, if there is more than one) is placed first in the set of four, and will indicate the suit.
Next, think of the remaining three cards as Low, Medium, and High values (their actual values don't matter.) Any pre-agreed order will suffice; for example, ascending face value (Ace to King), with the suit used as a tie-breaker, if necessary. We could use an alphabetical suit order: Clubs, Diamonds, Hearts, Spades. So, for example, 3S would come before 7C; and, using the tie-breaker, 7C would come before 7H. Hence the Low-Medium-High ordering in this case would be: 3S, 7C, 7H.
Given Low, Medium, and High, we can encode a number between one and six as follows:
LMH = 1, LHM = 2, MLH = 3, MHL = 4, HLM = 5, HML = 6.
This still leaves us short, as the hidden card could be one of 12. However, there is one opportunity to impart information we have not yet used: A gets to decide which of the cards from the duplicate suit to retain, and which to hand back to the audience member. How can the retained card be used to indicate more than just the suit?
Imagine the 13 face values (Ace to King) arranged in a circle. The shorter path between two cards, counting forward from one card to the other, is never more than six places. Therefore, A chooses to retain a card that begins the shorter path, and hands the other card to the audience member. B uses the encoded number to count forward from the first card in the hand of four.
Example: the audience member selects the following cards - 2C, 5D, JC, 5H, KS - and passes them to A. The only duplicate suit is clubs. Counting forward from JC to 2C is four places, so A retains JC and hands back 2C to the audience member. The first card in A's hand will therefore be JC. Of the other three cards, 5D is Low, 5H is Medium (using the suit tie-breaker), and KS is High. To represent four, A uses the ordering MHL. So the four cards are: JC, 5H, KS, 5D.
To decode, B notes that he must count forward from JC. He notes that the natural ordering of the other three cards is: 5D, 5H, KS, and so the cards 5H, KS, 5D represent ordering MHL, which encodes the number four. He therefore counts forward four places from JC and announces the two of clubs!
With a little practice, this trick can be made to flow quite smoothly. If performed repeatedly before the same audience, it is advisable to permute the position of the suit card, to make the trick harder to read. For example, on the nth performance, place the suit card in the (n modulo 4)th position.
---------------------------------------------
A Spanish magician was doing a magic trick. He said, “Uno, dos…”
and then he disappeared without a tres.

Find the smallest natural number greater than 1 billion (10^9) that has exactly 1000 positive divisors.
(The term divisor includes 1 and the number itself. So, for example, 9 has three positive divisors.)
Quote: Gialmere
Find the smallest natural number greater than 1 billion (10^9) that has exactly 1000 positive divisors.
(The term divisor includes 1 and the number itself. So, for example, 9 has three positive divisors.)
link to original post
810,810,000 = 24345471111131
?
My answer was wrong.
Quote: ChesterDog
810,810,000 = 24345471111131
?
...you're definitely on the right path as 810,810,000 is the smallest number with 1,000 divisors.
1,060,290,000 = 2^3 * 3^3 * 5^3 * 7 * 11 * 17
Quote: GialmereQuote: ChesterDog
810,810,000 = 24345471111131
?
...you're definitely on the right path as 810,810,000 is the smallest number with 1,000 divisors.
link to original post
1,969,110,000 = 243454111131171
?
Quote: Wizard
1,060,290,000 = 2^3 * 3^3 * 5^3 * 7 * 11 * 17
link to original post
Quote: ThatDonGuy
2^4 x 3^4 x 5^4 x 7 x 11 x 17 = 1,060,290,000
link to original post
Correct!!
Let n = p1a1 · ... · prar , where p1 ... pr are prime numbers, and a1 ... ar are positive integers.
Now, each divisor of n is composed of the same prime factors, where the ith exponent can range from 0 to ai.
Hence there are a1 + 1 choices for the first exponent, a2 + 1 choices for the second, and so on.
Therefore the number of positive divisors of n is (a1 + 1)(a2 + 1) ... (ar + 1).
The unique prime factorization of 1000 is 23 · 53, which contains six prime factors.
So if n has exactly 1000 positive divisors, each ai + 1 is a divisor of 1000, where i may take any value between 1 and 6.
At one extreme, when i = 1, a1 + 1 = 1000, so a1 = 999, and the smallest integer of this form is 2999, a number with 301 decimal digits.
At the other extreme, when i = 6, a1 = a2 = a3 = 1, and a4 = a5 = a6 = 4.
In this latter case, the smallest integer of this form is clearly 24 · 34 · 54 · 7 · 11 · 13 = 810,810,000.
In fact, of all the natural numbers with exactly 1000 divisors, 810,810,000 is the smallest. A demonstration by enumeration follows. Having established this result, it will be a simple matter to find the smallest integer greater than 1 billion that has 1000 divisors.
Let n = 24 · 34 · 54 · 7 · 11 · 13.
The smallest integer that can be obtained by combining the six prime factors in various ways is:
5 · 5 · 5 · 4 · 2, yielding 24 · 34 · 54 · 73 · 11 = (72/13)n > 3n
10 · 5 · 5 · 2 · 2, yielding 29 · 34 · 54 · 7 · 11 = (25/13)n > 2n
25 · 5 · 2 · 2 · 2, yielding 224 · 34 · 5 · 7 · 11 = (220/53 · 13)n > 500n
8 · 5 · 5 · 5, yielding 27 · 34 · 54 · 74 = (23 · 73/11 · 13)n > 10n
10 · 5 · 5 · 4, yielding 29 · 34 · 54 · 73 = (25 · 72/11 · 13)n > 10n
10 · 10 · 5 · 2, yielding 29 · 39 · 54 · 7 = (25 · 34/11 · 13)n > 15n
20 · 5 · 5 · 2, yielding 219 · 34 · 54 · 7 = (215/11 · 13)n > 200n
25 · 5 · 4 · 2, yielding 224 · 34 · 53 · 7 = (220/5 · 11 · 13)n > 1000n
Any other combination of the prime factors would contain a power of 2 greater than 30, which, on its own, would yield an integer greater than 1 billion.
Therefore, the smallest natural number with exactly 1000 divisors is 810,810,000.
To find the smallest number greater than 1 billion with exactly 1000 divisors, we must substitute larger prime(s) in the factorization of 810,810,000.
Logically, the smallest such substitution must be either: replace 54 · 7 with 5 · 74, or replace 13 with 17.
Arithmetically, we find that 24 · 34 · 5 · 74 · 11 · 13 = 2,224,862,640, while 24 · 34 · 54 · 7 · 11 · 17 = 1,060,290,000.
Therefore the smallest natural number greater than 1 billion that has exactly 1000 positive divisors is 1,060,290,000.
------------------------------------------------------------
Quote: GialmereFind the smallest natural number greater than 1 billion (10^9) that has exactly 1000 positive divisors.
2^4 x 3^4 x 5^4 x 7 x 11 x 17 = 1,060,290,000
First note the prime factorization of 1,000 is 5^3 * 2^3
Our answer should be in the form a^4 * b^4 * c^4 * d * e * f, where these terms are not necessarily different.
Why? Let's call the ultimate answer n.
All 1,000 factors of n will be have a prime factorization including 0 to 4 a's, for example. In other words, it can have 0 a's, 1 a, 2 a's, or 3 a's. That is five (0 to 4) possibilities for the number of a's in a factor.
All 1,000 factors of n will also have 0 to 4 b's, 0 to 4 c's, 0 or 1 d, 0 or 1 e, and 0 or 1 f.
This makes 5*5*5*2*2*2 = 1,000 possible factorizations.
Now, what should a to f be? It's obviousl they should all be prime. Let's start with the a=2, b=3, c=5, d=7, e=11, and f=13. 2^4 * 3^4 * 5^4 * 7 * 11 * 13 = 810,810,000, which is too small.
Let's increase f to 17 and we have 2^4 * 3^4 * 5^4 * 7 * 11 * 17 = 1,060,290,000, which meets the condition of being greater than a billion.
I realize this isn't proving there isn't another solution that is between a billion and my answer, but I have it on good authority my answer is correct.
Quote: WizardGood puzzle. I think I'll make an Ask the Wizard question out of it. Here is a draft of my "solution."
First note the prime factorization of 1,000 is 5^3 * 2^3
Our answer should be in the form a^4 * b^4 * c^4 * d * e * f, where these terms are not necessarily different.
Why? Let's call the ultimate answer n.
All 1,000 factors of n will be have a prime factorization including 0 to 4 a's, for example. In other words, it can have 0 a's, 1 a, 2 a's, or 3 a's. That is five (0 to 4) possibilities for the number of a's in a factor.
All 1,000 factors of n will also have 0 to 4 b's, 0 to 4 c's, 0 or 1 d, 0 or 1 e, and 0 or 1 f.
This makes 5*5*5*2*2*2 = 1,000 possible factorizations.
Now, what should a to f be? It's obviousl they should all be prime. Let's start with the a=2, b=3, c=5, d=7, e=11, and f=13. 2^4 * 3^4 * 5^4 * 7 * 11 * 13 = 810,810,000, which is too small.
Let's increase f to 17 and we have 2^4 * 3^4 * 5^4 * 7 * 11 * 17 = 1,060,290,000, which meets the condition of being greater than a billion.
I realize this isn't proving there isn't another solution that is between a billion and my answer, but I have it on good authority my answer is correct.
link to original post
Actually, in the form a^4 * b^4 * c^4 * d * e * f, they do all have to be differrent. If, say, e = f, then you are counting the values with one f but not two twice.
It needs to be:
1000 = (P2 + 1) * (P3 + 1) * (P5 + 1) * (P7 + 1) * ...
where Pn is the number of times prime factor n appears in the number.
It could be a^4 b^4 c^4 d e f, but it could also be a^4 b^4 c^4 d^3 e, or a^4 b^4 c^4 d^7, or a^9 b^4 c^4 d e, among other possibilities. Add 1 to each exponent, and the product of the results = 1000.
Quote: Gialmere
...what color would your Lamborghini be?
link to original post
I like the quote - the billion dollars has probably already been through the charities over the years that are looking at solutions to famine, obviously not enough, so the billion probably wouldn't end world hunger.
For the record, I'd go Yellow, authentic.
Quote: ThatDonGuy
First note the prime factorization of 1,000 is 5^3 * 2^3
Our answer should be in the form a^4 * b^4 * c^4 * d * e * f, where these terms are not necessarily different.
Why? Let's call the ultimate answer n.
All 1,000 factors of n will be have a prime factorization including 0 to 4 a's, for example. In other words, it can have 0 a's, 1 a, 2 a's, or 3 a's. That is five (0 to 4) possibilities for the number of a's in a factor.
All 1,000 factors of n will also have 0 to 4 b's, 0 to 4 c's, 0 or 1 d, 0 or 1 e, and 0 or 1 f.
This makes 5*5*5*2*2*2 = 1,000 possible factorizations.
Now, what should a to f be? It's obviousl they should all be prime. Let's start with the a=2, b=3, c=5, d=7, e=11, and f=13. 2^4 * 3^4 * 5^4 * 7 * 11 * 13 = 810,810,000, which is too small.
Let's increase f to 17 and we have 2^4 * 3^4 * 5^4 * 7 * 11 * 17 = 1,060,290,000, which meets the condition of being greater than a billion.
I realize this isn't proving there isn't another solution that is between a billion and my answer, but I have it on good authority my answer is correct.
link to original post
Actually, in the form a^4 * b^4 * c^4 * d * e * f, they do all have to be differrent. If, say, e = f, then you are counting the values with one f but not two twice.
It needs to be:
1000 = (P2 + 1) * (P3 + 1) * (P5 + 1) * (P7 + 1) * ...
where Pn is the number of times prime factor n appears in the number.
It could be a^4 b^4 c^4 d e f, but it could also be a^4 b^4 c^4 d^3 e, or a^4 b^4 c^4 d^7, or a^9 b^4 c^4 d e, among other possibilities. Add 1 to each exponent, and the product of the results = 1000.
link to original post
Thanks, I agree.


