Behold, a square?
April 2nd, 2025 at 3:59:32 AM
permalink
Can't be correct?
Craps is paradise (Pair of dice).
Lets hear it for the SpeedCount Mathletes :)
April 2nd, 2025 at 5:07:59 AM
permalink
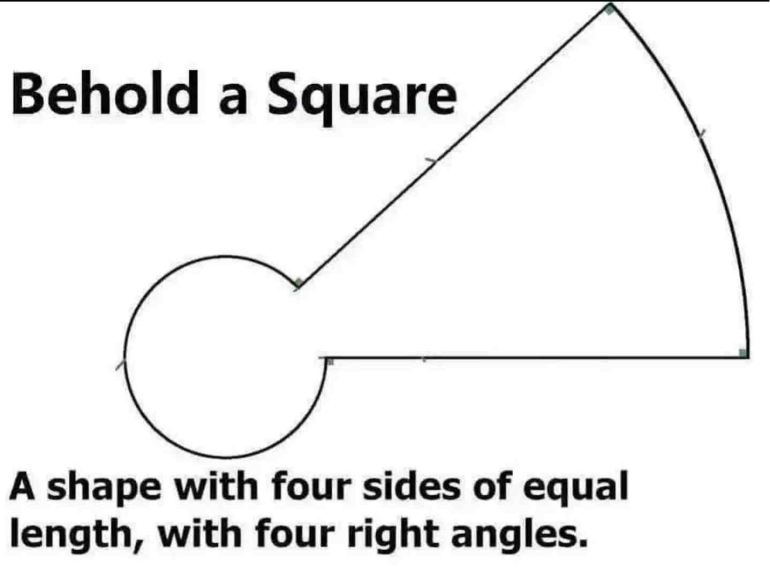
Can't be correct.
THIS is a square.
THIS is a square.
May the cards fall in your favor.
April 2nd, 2025 at 5:10:44 AM
permalink
A square is a four sided figure - each side is equal in length and adjacent sides are perpendicular.
What you have shown is a keyhole.
What you have shown is a keyhole.
Casino Enemy No.1
April 2nd, 2025 at 6:05:22 AM
permalink
Quote: 100xOdds
Can't be correct?
link to original post
Those two corners are not 90 degrees near the circle area. In retrospect, none of those corners look like they are 90 degrees.
You can't know everything, but you can know anything.
April 2nd, 2025 at 7:32:44 AM
permalink
Quote: 100xOdds
Can't be correct?
link to original post
To be honestly fair, the picture looks like a chess piece ♟️. 😀
In both The Hunger Games and in gambling, may the odds be ever in your favor. :D
"Man Babes" #AxelFabulous
"Olive oil is processed but it only has one
ingredient, olive oil."-Even Bob, March 27/28th. :D The 2 year war is over! Woo-hoo! :D
I sometimes speak in metaphors. ;) Remember this. ;)
Crack the code. :D 8.9.13.25.14.1.13.5.9.19.14.1.20.8.1.14! :D
"For about the 4096th time, let me offer a radical idea to those of you who don't like Nathan -- block her and don't visit Nathan's Corner. What is so complicated about it?" Wizard, August 21st. :D
April 2nd, 2025 at 7:51:24 AM
permalink
It reminds me of Felix's old foe, The Master Cylinder.
The older I get, the better I recall things that never happened
April 2nd, 2025 at 8:12:37 AM
permalink
First, technically, none of the angles are "right angles"; their tangents may be right angles, but that's different.
Second, the four sides of a square have to be line segments.
Second, the four sides of a square have to be line segments.
April 2nd, 2025 at 8:16:41 AM
permalink
Quote: ThatDonGuyFirst, technically, none of the angles are "right angles"; their tangents may be right angles, but that's different.
Second, the four sides of a square have to be line segments.
link to original post
Isn't a curve a line? The equator is a straight line that curves around the globe, no?
The older I get, the better I recall things that never happened
April 2nd, 2025 at 10:44:19 AM
permalink
Quote: billryanQuote: ThatDonGuyFirst, technically, none of the angles are "right angles"; their tangents may be right angles, but that's different.
Second, the four sides of a square have to be line segments.
link to original post
Isn't a curve a line? The equator is a straight line that curves around the globe, no?
link to original post
Not in Euclidian geometry. A "straight" line cannot curve.
I am not exactly sure what the "strict" definition of line is, but I am pretty sure it is something like, "A path such that, given any two different points A and B on that path, the path from A to B that has the shortest distance is entirely on the line's path."
April 2nd, 2025 at 11:40:02 AM
permalink
There may be a bit about all parts of a line segment are coplanar, for all planes including the endpoints of the line segment.
(This does not hold true for arcs.)
(This does not hold true for arcs.)
May the cards fall in your favor.
April 2nd, 2025 at 11:56:36 AM
permalink
Quote: ThatDonGuyQuote: billryanQuote: ThatDonGuyFirst, technically, none of the angles are "right angles"; their tangents may be right angles, but that's different.
Second, the four sides of a square have to be line segments.
link to original post
Isn't a curve a line? The equator is a straight line that curves around the globe, no?
link to original post
Not in Euclidian geometry. A "straight" line cannot curve.
I am not exactly sure what the "strict" definition of line is, but I am pretty sure it is something like, "A path such that, given any two different points A and B on that path, the path from A to B that has the shortest distance is entirely on the line's path."
link to original post
If a line extends infinitely in both directions, and we live on a globe, aren't all lines circles ?
If I start at Point A and walk in a straight line, I'll eventually end up back at the same spot.
What am I missing?
The older I get, the better I recall things that never happened
April 2nd, 2025 at 12:36:04 PM
permalink
Quote: billryanQuote: ThatDonGuyQuote: billryanQuote: ThatDonGuyFirst, technically, none of the angles are "right angles"; their tangents may be right angles, but that's different.
Second, the four sides of a square have to be line segments.
link to original post
Isn't a curve a line? The equator is a straight line that curves around the globe, no?
link to original post
Not in Euclidian geometry. A "straight" line cannot curve.
I am not exactly sure what the "strict" definition of line is, but I am pretty sure it is something like, "A path such that, given any two different points A and B on that path, the path from A to B that has the shortest distance is entirely on the line's path."
link to original post
If a line extends infinitely in both directions, and we live on a globe, aren't all lines circles ?
If I start at Point A and walk in a straight line, I'll eventually end up back at the same spot.
What am I missing?
link to original post
The fact that you are not walking on a "straight line" if you are on a sphere - the line curves along the boundary of the sphere.
Think of it this way: shoot, say, a laser beam from the surface of the earth, parallel (well, tangent) to the surface. Would it go around the earth and return to you, or would it go out into space?
April 2nd, 2025 at 12:38:58 PM
permalink
Quote: ThatDonGuyQuote: billryanQuote: ThatDonGuyFirst, technically, none of the angles are "right angles"; their tangents may be right angles, but that's different.
Second, the four sides of a square have to be line segments.
link to original post
Isn't a curve a line? The equator is a straight line that curves around the globe, no?
link to original post
Not in Euclidian geometry. A "straight" line cannot curve.
I am not exactly sure what the "strict" definition of line is, but I am pretty sure it is something like, "A path such that, given any two different points A and B on that path, the path from A to B that has the shortest distance is entirely on the line's path."
link to original post
d2y/dx2=0 is good enough in Cartesian, no?
