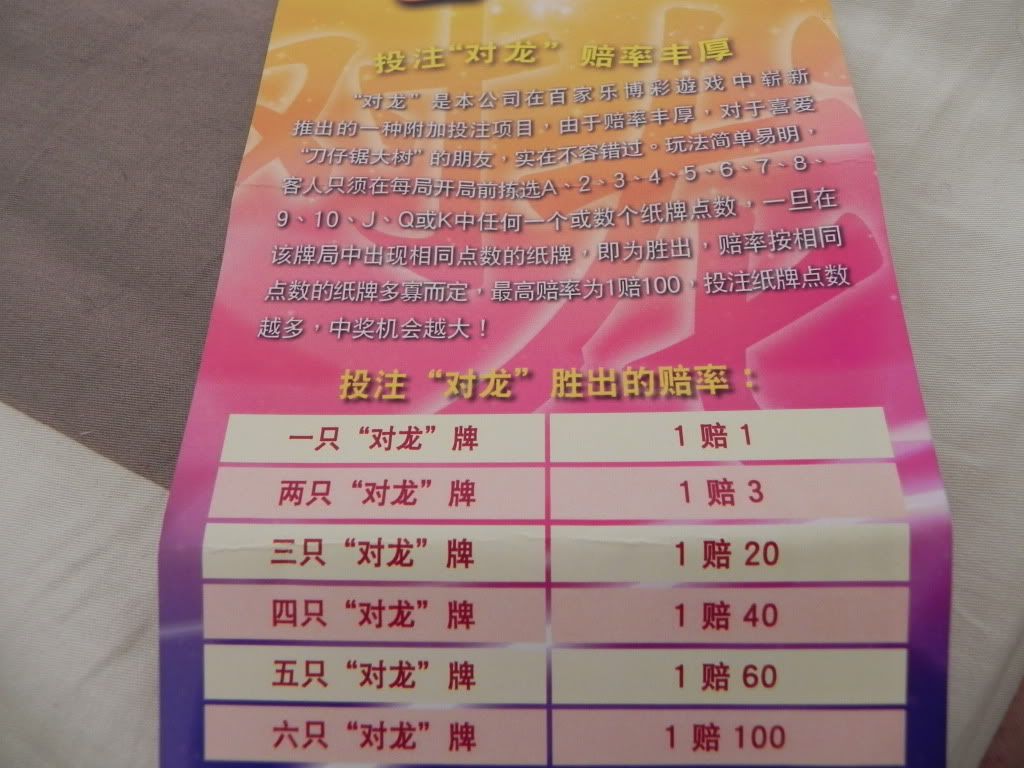
You probably don't read Chinese but i think it's not too hard to get the idea from the picture.
You bet on a rank and if a card of that rank shows up (doesn't matter it's banker or player), you win:
1 card 1:1
2 cards 1:3
3 cards 1:20
4 cards 1:40
5 cards 1:60
6 cards 1:100
Assuming average 5 cards per hand, and just with the 1:1 payout, I worked out that the player had an advantage when any rank's concentration reach 13%.
My question is, assuming 6 or 8 decks, how often would you see a rank reaching 13% concentration?
I.E. Only if both player and banker are holding two aces, twos, sixes, sevens or tens/faces will you see a fifth card that could possibly win you the 1:60 or 1:100 payout. That limits the number of opportunities you'll have for the big money.
And for this side bet, faces must be the same rank to qualify.
At least that's the way I see it.
What if player and banker hold different pairs? What if both end up with different sets?
Well, since you asked.Quote: WizardAsk and ye shall receive. Check out my analysis of the Matching Dragon. Comments, questions, suggestions?
Mind you, these are nit-picks....
For the combos chart, the rows go from A thru K as they should. You gotta fix a couple row headings for the other charts.
Columns 5 & 6 for rows 3, 4, 8 & 9 would be blank (or a hyphen) in ALL the charts.
Why not put in the photo of the rule card?
In fact, I still didn't realize that until after I posted my second reply above.
Perhaps that need to be made more clear?
Then again, maybe I'm just thick-skulled about it....
Quote: DJTeddyBearBTW: It occurs to me that when I wrote my first reply above, I did not realize that this is 13 separate side bets.
I did say it was 13 separate bets, but just made some edits to hopefully avoid others having the same confusion.
Quote: rudeboyoiid like to see the layout of this sidebet on the table.
Sorry, all I know about it is the first post in this thread. I had the sign translated, but it said what the OP said it said.
On a separate note, when I view this page in Google Chrome some of the probabilities are formatted to look like phone numbers. In particular, those starting with 0.0041. If I click on them Skype will call the numbers. Does anyone know how to make Chrome not interpret the number as a phone number?
I arrived at an average 5-card hand from The Wizard's page, and just rounded it up.
"In a six-deck the average number of player cards is 2.503332474, banker cards is 2.435277628, and total cards is 4.93861010"
Then I do a (very) rough calculation (0.5) ^ (1/5) = 0.87, meaning the chance that the cards you didn't pick showing up 5 times in a row is less than 50% if the concentration is less than 87%, i.e. the player will have an advantage when any rank reaches 13% or more.
I do realize this 13% is overstated because:
1. the 2 - 6 cards payout is assumed to be just 1:1 in the calculation
2. each unfavorable card drawn will increase the advantage a little bit more.
Now the question is, how likely is it that any rank would reach 13%? With 6 decks, that is like drawing 128 cards out of 312 without one particular rank showing up. Seems quite remote. But with 1 deck, it is like drawing 22 cards out of 52, but remember, you can always draw the first 12 cards without seeing a particular rank, so it doesn't seem to be quite as remote.
Hard to say if the choice of card matters much, all the bets seem to turn profitable around the same spot, too much variability even across 10000 shoes to find the exact spot.
Next steps:
-test whether good betting occasions really happen that often
-simulate betting when concentrations peak over 9% or 9.5%, track profit. Then we can tell if it's worth your time.
If you only bet with a 9.5% or higher density across all cards, profits in 1 simulation of 10000 shoes was 18340 units, or around 2 units per shoe.
Not huge profit, but I'd say enough to make it worth it if you can track all the cards with pen&paper.
12 units per shoe is some serious profit, almost worth a special trip. I suspect the variance will be rough, tough to estimate that through my simulation.
Someone needs to design a counting strategy to estimate when you hit 9.5% or higher.
EDIT: Another 1,000,000 shoe simulation showed a profit of 18 units per shoe. This is countable, but the variance will eat you up
Also, the 9 seems to be the weakest in terms of exploiting for card counting. Best to wait until the 9 hits almost 10% before betting it.
if it is indeed profitable at the 9-10% range, it is much more worthwhile than the 13% estimate. Meanwhile, I will try to reconcile the difference using the primitive way - Excel.
The 2 cards pay 3 and 3 cards pay 20 had a big impact on the return and using my primitive tool (that's Excel) I got a +'ve return at around 9% as well.
Quote: dwheatleyI have confirmed that at least one card will be 9% or better 64% of the time, and 9.5% or better 50% of the time in an 8 deck shoe with 1 deck cut.
If you only bet with a 9.5% or higher density across all cards, profits in 1 simulation of 10000 shoes was 18340 units, or around 2 units per shoe.
Not huge profit, but I'd say enough to make it worth it if you can track all the cards with pen&paper.
Quote: dwheatleyI just ran a 1,000,000 shoe simulation, and got a profit of 12 units per shoe if you bet at 9.5% density or higher for every number.
12 units per shoe is some serious profit, almost worth a special trip. I suspect the variance will be rough, tough to estimate that through my simulation.
Someone needs to design a counting strategy to estimate when you hit 9.5% or higher.
EDIT: Another 1,000,000 shoe simulation showed a profit of 18 units per shoe. This is countable, but the variance will eat you up
Also, the 9 seems to be the weakest in terms of exploiting for card counting. Best to wait until the 9 hits almost 10% before betting it.
Sorry but i want to clarify something. In the first post you said profit is 2 units per shoe, in the second you quoted a figure of 12 and 18. Is the first figure a typo or is the variance really that great?
You can calculate the variance on the bet itself, which will be quite large. The variance will only increase as you play more shoes.
Quote: dwheatleyI just ran a 1,000,000 shoe simulation, and got a profit of 12 units per shoe if you bet at 9.5% density or higher for every number.
12 units per shoe is some serious profit, almost worth a special trip. I suspect the variance will be rough, tough to estimate that through my simulation.
Someone needs to design a counting strategy to estimate when you hit 9.5% or higher.
EDIT: Another 1,000,000 shoe simulation showed a profit of 18 units per shoe. This is countable, but the variance will eat you up
Also, the 9 seems to be the weakest in terms of exploiting for card counting. Best to wait until the 9 hits almost 10% before betting it.
That all depends on how deep they go into the shoe! How deep were you assuming?
Anyhow you may all cancel your flights now, since they changed the payouts:
New Payout
I don't think you need a special counting system, what you have to do is just record how many cards for each rank( Ace to King) left in the shoe and then divide by total cards in the shoe to get the density ! Bet when above the break even point ! Am I right ?
Will run a detail simulation(for old payout) to find out the edge, variance, betting frequency/opportunity and return per shoe.
Will also simulate for new payout.
Old payout : (0,-1), (1,1), (2,3), (3,20), (4,40), (5,60) and (6,100).
New payout : (0,-1), (1,1), (2,2), (3,15), (4,30), (5,60) and (6,100).
Please give your comments/feedback in order to get better simulation.
cheers
BIG hurdle to overcome the new pay table !
Quote: ssho88BIG hurdle to overcome the new pay table !
Again it depends on how deep they go into the shoe. At the moment it is more than 2 decks that remain unseen. With a max bet of HK$1000 (US$125) you can still get a tiny bit of extra pocket money out of it, but it is no longer worth a First Class flight and a hotel suite stay with 3 Michelin Star food (also available in Lisboa).
a) Total 4 cards per round, probability = ?
b) Total 5 cards per round, probability = ?
c) Total 6 cards per round, probability = ?
cheers
Quote: ssho88what is the probability of the no of cards dealt per round for baccarat ?
a) Total 4 cards per round, probability = ?
b) Total 5 cards per round, probability = ?
c) Total 6 cards per round, probability = ?
cheers
See Baccarat Appendix 1.
Quote: ssho88what is the probability of the no of cards dealt per round for baccarat ?
a) Total 4 cards per round, probability = ?
b) Total 5 cards per round, probability = ?
c) Total 6 cards per round, probability = ?
cheers
Which (online live) casinos offer this bet?
YOU CAN FIND IT AT www.m88.com
I need your advise/comments on this :-
By removing each card in turn from an eight-deck shoe, its effect on the house edge can be determined. This allows card counting systems to be developed
For an example, for the game of baccarat( 8 deck, cut card put at 14 cards from the end of shoe), if we want to check the EOR of the "9", removing a 9 leaves 415 cards, then start to simulate first round and say 5 number of cards were dealt and left 410 cards in the shoe.
My question here is whether :-
a) you continue to simulate the 2nd round, 3rd round�.until the 80th round(or until 14 cards left in the shoe) with the same shoe stated above(410 cards just before 2nd round...and left 14 cards+- at round 80th). After that only start with new shoe(remove a 9 of course !)
OR
b) you simulate the 2nd round with a totally new shoe with 415 cards(remove a 9 only), 3rd round with new shoe with 415 card�..means every round use a totally new shoe with 415 cards(without a 9 of course !).
OR
c) remove all "9" from the shoe(left 384 cards), and then start your simulation by using shoe without any "9" ?
cheers
Since this is no doubt long gone, can you disclose the casino now?Quote: WizardAsk and ye shall receive. Check out my analysis of the Matching Dragon. Comments, questions, suggestions?
Quote: teliotSince this is no doubt long gone, can you disclose the casino now?
Old Lisboa
Curious, I just visited this casino last month. Thanks.Quote: acwOld Lisboa
http://www.slot-machine-resource.com/podcasts/wachtel4.mp3


