What decimal equivalents of fractions do you and the general public know?

I've memorized lots of basic calculations, and it took me a long time to realize that most people haven't done the same. As such, they often think I'm a mathematical wizard for being able to "calculate" something so quickly, even though I'm not calculating, I just know the answer. The point of this post is to ask what extra calculations you've memorized, and what you think the general population has also memorized? This post is an offshoot of my other thread about how I saw a 20-something person ask Siri "What's 8 times 5?"

Multiplication
Beyond the 12x12 that's commonly taught in schools, I've memorized various products where one factor goes up to about 17 (e.g., 3x17, 4x17), certainly all the squares of 13-17. This isn't from an effort to memorize, it's just that I've done them so many times that eventually they became part of my memory.

Decimal Equivalents of Fractions
Handypeople and trade workers are always measuring in eighths of an inch, and often sixteenths, so I know the decimal equivalents of 1/8, 3/8, 5/8, and 7/8. I learned the sixteenths from trading stocks back in the days before decimal trading.

Decimal equivalents of sevenths
Years ago I noticed that the decimals for all the sevenths repeat:
1/7 = 0.142857
2/7 = 0.285714
3/7 = 0.428571
4/7 = 0.571428
5/7 = 0.714285
6/7 = 0.857142

So, anyone can ask me the decimal equivalent of a seventh, and to them, it looks like I'm calculating to six decimal places.

Percentages for other fractions
This isn't memorization, but it's a quick way to calculate percentages that most people don't seem to know. Say you want to know 5/6 and don't have it memorized. Most people know that's the same as 5÷6, and would punch that into a calculator if using a calculator, and if tasked with calculating it in their head, would probably try long division, which is possible, but there's an easier way. It's generally easier to divide something into 100 than into the numerator of the given fraction, so that's what I do. 100 ÷ 6 in my head is 16-2/3 without much difficulty, then I multiply that by 5 (the numerator), and get 83-1/3, or 83.333333%.

Yes, I know some fractional equivalents by heart.

Sometimes in my job I have to use fractional equivalents of 1/12 (corresponding to a fractional part of a year, in months). The quarter, thirds and half-year fractional equivalents are easy. The intermediate values I know off the top of my head:
1 month = .0833333 year
2 months = .0166667
5 months = .4166667
7 months = .5833333 (easy = 0.5 + 1 month)
10 months = .8333333 (easy = 1 month x 10)
11 months = .9166667

I also have occasion to use 5/9 of a percent (.0055556) and 5/18 of percent (.0027778).

If somebody asks me how to do something on a computer, I usually can't do it. I have to show them on the screen. But if they've got their eyes closed, I'm wasting my time.

I've also found that almost nobody knows this trick: Multiply hourly wage by 2000 to get yearly (or divide yearly by 2000 to get hourly). Because, of course, 40 hours week x 50 weeks/year = 2000. (If there's paid vacation for the other two weeks, estimate by dividing the yearly total by 3 then add 10% of that to the total.)

I realized that decades ago, the first time I manually multiplied 40 x 50 to do the conversion. Nobody I've talked to about this has realized that you can simply use 2000 as a factor. Again, they think I'm a math whiz when I quickly convert between hourly and yearly.

I've sometimes polled blackjack dealers on what they think fair earnings are for a dealer (wages + tips), either hourly or yearly. If they give it to me hourly then I used to convert to yearly, tell them the yearly amount, and compare it to other professions that pay that much. But then they'd think I'm a math genius and suspect me of counting cards. So now I compare without telling them the conversion.

About that, while I believe in tipping, I've found table games dealers to be quite entitled. One said $39 to $59 would be fair. That's more than the overwhelming majority of other jobs in the U.S., e.g.:

$23 paramedic
$25 paralegal
$30 school teacher
$32 electrician (journeyman)
$33 scientist
$34 medical sonographer
$35 accountant
$37 dental hygienist
$37 web developer
$39 scientist (chemist)
$40 architect
$40 college professor
$41 microbiologist
$41 occupational therapist
$43 nurse
$43 computer programmer
$48 veterinarian
$50 electrical engineer
$56 nuclear engineer
$57 nurse practitioner
$57 aerospace engieer
$60 judge

355/113 is an approximation near to my heart.

It's probably close enough for anything I'm building, given the "mark with chalk, cut with an axe" nature of the physical world.

If my latest 10,000 hands of BJ paid me $100K, how much is that per hour? It depends on if I was playing at 50 hands an hour, or 300 hands an hour on a machine. That means it could take anywhere from 200 hours down to as little as 33.3 hours. I can't really figure an hourly rate from that. I'd have to go by an extreme guesstimate of how many hands were played if I wasn't actually counting them either. If I'm lucky, my losing sessions will only total one-third of my winning sessions for NYS tax purposes. So if I have 2 dozen losing sessions totaling $50K and 1 dozen winning sessions totaling $150K, that's a $100K profit.

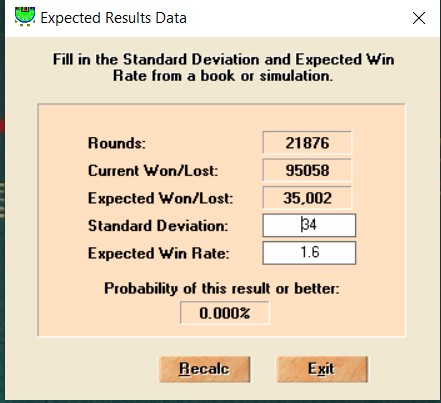
As for Standard Deviation, I know not enough, especially as how it pertains to winning at card counting. But I can compare what card counters are supposed to be winning with what I'm winning, and it's just impossible at 34 SD. BTW, I lost $5K on my first 10K hands. I had to loosen my progressions up considerably to get ahead in the second 10K hands. I guess I'm supposed to multiply the number of hands played by 1.6 (the default number for some unknown reason) and the result is the expected profit for that point in time.

I’ve used the “2” conversion for many years. If someone makes $30/hour, that’s about 30*2 = $60k per year

For people who use slightly more advanced math, it’s very useful to memorize the base 10 logarithms of 1 - 10. 1 and 10 are obviously 0 and 1, 2 is 0.3, 3 is 0.48 etc. You actually only need to memorize 2, 3, 5 and 7 because the other numbers build off of those (for instance, since 6 = 2 * 3 then log(6) is .3 +.48 = .78.

So if someone asks what’s the chance of flipping twenty consecutive heads, the answer is 1 in :

2^20 =~ 10^(.3 *20) = 10^6 = 1,000,000. Within 5% of exact answer of 1,048,576

To calculate the probability of eighteen consecutive yo’s it’s 1 in:
10^((.48 + .78) * 18). You don’t even need to be that careful because you’re often just trying to get the order of magnitude. In this case it’s 10^22…so remote that you know it didn’t happen. RIP Alan.

It’s also useful to know the approximate probabilities for 1,2,3,4,5 and 6 standard deviation events.

You should also know some basic metric like miles/Km, pounds/kg, F/C.

Yet another: one degree of latitude is about seventy miles. Similar for longitude unless you’re close to a Pole

Quote: Ace2

You should also know some basic metric like miles/Km, pounds/kg, F/C.

Yeah, for 12 years I've been trying to teach my foreign-born family how to do these conversions in their head, but they're not interested in learning, probably because they know I'll do it for them.

I'd like to hear from the Wizard what kinds of already-calculated items are part of his mental library. I don't think it's oversharing to say that his wife once told me that she noticed him reciting the digits of pi in his sleep.

The only math most people need to memorize today is their PIN. Everything else is at our fingertips.

Quote: billryan

The only math most people need to memorize today is their PIN. Everything else is at our fingertips.
link to original post

PIN? Just about everything uses facial recognition now

Even US Customs (Global Entry). No passport or forms required to enter the country

Quote: MichaelBluejay

I've also found that almost nobody knows this trick: Multiply hourly wage by 2000 to get yearly (or divide yearly by 2000 to get hourly). Because, of course, 40 hours week x 50 weeks/year = 2000. (If there's paid vacation for the other two weeks, estimate by dividing the yearly total by 3 then add 10% of that to the total.)

I realized that decades ago, the first time I manually multiplied 40 x 50 to do the conversion. Nobody I've talked to about this has realized that you can simply use 2000 as a factor. Again, they think I'm a math whiz when I quickly convert between hourly and yearly.

I've sometimes polled blackjack dealers on what they think fair earnings are for a dealer (wages + tips), either hourly or yearly. If they give it to me hourly then I used to convert to yearly, tell them the yearly amount, and compare it to other professions that pay that much. But then they'd think I'm a math genius and suspect me of counting cards. So now I compare without telling them the conversion.

About that, while I believe in tipping, I've found table games dealers to be quite entitled. One said $39 to $59 would be fair. That's more than the overwhelming majority of other jobs in the U.S., e.g.:

$23 paramedic
$25 paralegal
$30 school teacher
$32 electrician (journeyman)
$33 scientist
$34 medical sonographer
$35 accountant
$37 dental hygienist
$37 web developer
$39 scientist (chemist)
$40 architect
$40 college professor
$41 microbiologist
$41 occupational therapist
$43 nurse
$43 computer programmer
$48 veterinarian
$50 electrical engineer
$56 nuclear engineer
$57 nurse practitioner
$57 aerospace engieer
$60 judge
link to original post

I don’t know where you are getting your info from. I know a few veterinarians who all make well over $100 an hour.

Whenever I saw what anesthesiologists theoretically make on these type lists, it was usually around 50% of the actual figure. I would have been laughed at if I put an ad out for what the listed figure was.

I was gonna say the same. $80k per year for a college professor and $100k for an electrical engineer??

25 years ago maybe

Quote: SOOPOO

I don’t know where you are getting your info from.

I generally get salary info from BLS.gov, sometimes from Salary.com or similar sources. I pulled the figures above a couple years ago, and BLS data is itself a year or two old, but a couple years ago is also when I surveyed the dealer who said $39-59 would be fair earnings for a dealer.

My friend is a traveling nurse and post-covid is getting over a thousand dollars per ten-hour shift, with as much overtime as she wants most weeks.

Medians from BLS.gov • Salary.com (78722) today, with BLS noting that its data is 2021.

Anesthesiologist*: $100 • $210
Veterinarian: $48 • $52
College Professor†: $40 • $54
Electrical Engineer: $31 • $61

* BLS.gov lumps all physicians together, this figure is not anesthetist-specific.
† Mathematics
-------------------------------

Based on your feedback, it seems that BLS significantly underestimates real-world salaries. I'll use other sources from here on out.

The problem with the professor category is that Asst and Assoc Professors are very low paid, and full Professors are much higher paid ($150 - 200K/y at a major university.) I guess there are a ton of minor or fringe universities which might pull the average salaries down to a lower level.

I have been surprised by how much wages have increased over the last two years in my field of software engineering. I would guess they are up close to 30% in the last two years.

A Supreme Court Justice makes less than $300,000 yearly, while Judge Judy makes nearly $30,000,000.

Here's a related topic: I've figured out lots of shortcuts for calculation, or can figure them out on the fly. So people sometimes think I'm a Rainman / mathematical genius, because they think I'm calculating the hard way, when I'm not.

For example, today I saw these two numbers in a report: 133,124, and 7,223.

I wanted to know the percentage (second number divided by first). After a few seconds I came up with the right answer, 5.4%. I didn't do it by dividing 7,223 into 133,124 which would be tortuous. Here was my thinking:

For estimating 7223 ÷ 133,124, I could do 7 ÷ 133. Call it 7.2 ÷ 133 for extra accuracy.

Since I want a percentage, the decimal moves over two digits. So 133 becomes 1.33.

1.33 is about the same as the fraction 4/3.

So:
7223 ÷ 133,124 »
7.2 ÷ 133 »
7.2 ÷ 1.33% »
7.2 ÷ (4/3)% »
7.2 x (3/4)% »
21.6 ÷ 4 %»
(20 ÷ 4) + (1.6 ÷ 4) % »
5 + 0.4 % »
5.4%

Quote: billryan

A Supreme Court Justice makes less than $300,000 yearly, while Judge Judy makes nearly $30,000,000.
link to original post

Not a fair comparison.

SCOTUS are government employees.
Judy is an entertainment superstar.

Quote: MichaelBluejay

I've also found that almost nobody knows this trick: Multiply hourly wage by 2000 to get yearly (or divide yearly by 2000 to get hourly). Because, of course, 40 hours week x 50 weeks/year = 2000. (If there's paid vacation for the other two weeks, estimate by dividing the yearly total by 3 then add 10% of that to the total.)
link to original post

The federal civil service uses a 2087-hour year for converting hourly to yearly wages (and vice versa).

A couple of tricks to remember decimal versions of fractions:
1 / 9 = 0.1111111...
2 / 9 = 0.222222...
and so on
1 / 11 = 0.09090909...
2 / 11 = 0.18181818...
3 / 11 = 0.27272727...
and so on

Here's something to note: the number of digits in the repeating term of the fraction m / n, where n is positive, < n