The Bingo Paradox

In the following video, Matt Parker, of whom I am a big fan, attempts to explain why you see more horizontal than vertical bingos in bingo.


Direct: https://www.youtube.com/watch?v=AHP1T8fTxpQ

He is right that there are more horizontal bingos called in a room full of cards. However, his reasoning is all wrong. He seems to argue that even on a single card, this holds true. To be specific, he says the probability of a horizontal bingo is 3x that of a vertical one. I'm a big fan of Matt Parker and his Stand-Up Maths YouTube channel. However, I have to call him out on this one.

Consider the table he shows at the 23:21 point, that he uses to make is 3x argument.



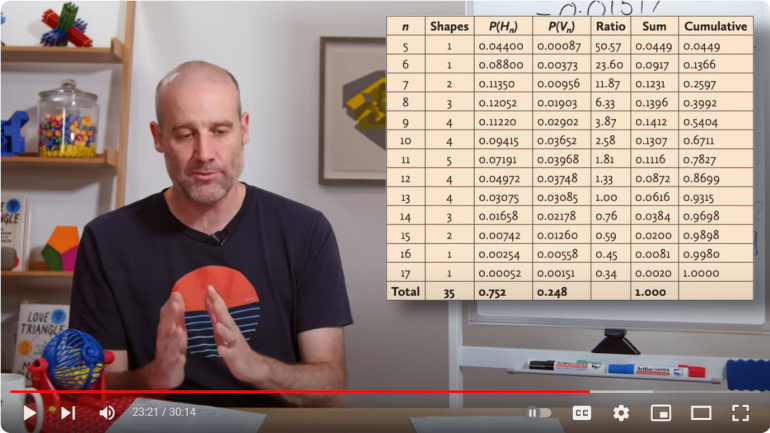
Before going on, he uses an assumption of no free square, which I shall also, to compare apples to apples.

The 0.00087, which he calls P(V₅), is supposed to be the probability of a vertical bingo with 5 daubs. However, he doesn't take into consideration the daub placement in the table. It is easy to see the probability of getting a vertical bingo in 5 daubs is (4/24)*(3/23)*(2/22)*(1/21) = 0.00009411. Matt is not answering the right question. His figure is the probability the first five numbers drawn will be from the same column.

The correct probability of a horizontal bingo in five daubs is also 0.00009411. Not the 0.04400 , which he calls P(H₅).

I attest that with one card, the probability of a horizontal bingo equals a vertical bingo.

Briefly, the reason you see more horizontal bingos in a multi-player game than vertical is many games it takes a long time for one letter to get called, while the other four letters have been called multiple times each. When that fifth letter is finally called, the whole room erupts in "Bingo!" Those bingos are all going to be horizontal. This explanation is based more on experience playing than actual math.

Again, I do not call out Matt Parker lightly and would love to get a third opinion on this. Thank you.

P.S. What is Karen's opinion this?

Starting about the 7:13 point, Matt paraphrases an academic paper on bingo I haven't read, based on 1,000 cards. He doesn't say whether that paper used the free square or whether it tracked diagonal wins. Matt mentions both a 2-1 and 3-1 ratio of horizontal to vertical, but the 2-1 ratio seems to be the figure from the paper.

Here are my results based on my own simulation, with and without the free square.

Win	No Free Square	Free Square
Vertical	35.89%	29.10%
Horizontal	45.82%	30.77%
Diagonal	18.30%	40.13%

The only way I could be in loose agreement with the paper is if diagonal bingos counted as horizontal and no free square.

How is it possible to have a diagonal bingo without using the free square?

Quote: avianrandy

How is it possible to have a diagonal bingo without using the free square?
link to original post

No free square means that there is a 5th N number. It's not like the square is a blocker.

Here are some more detailed results on the winning bingo:

Winning Bingo	No Free Square	Free Square
B column	7.19%	2.89%
I column	7.18%	2.88%
N column	7.17%	17.57%
G column	7.18%	2.88%
O column	7.17%	2.88%
Row 1	9.16%	2.67%
Row 2	9.17%	2.69%
Row 3	9.16%	20.06%
Row 4	9.17%	2.68%
Row 5	9.16%	2.67%
NW to SE diagonal	9.15%	20.04%
SW to NE diagonal	9.14%	20.09%

Sample size (bingos achieved):
No free square: 8,431,726
With free square: 3,545,727

Maybe you can answer a question (via simulation) that I have always wondered about:

Consider 3 types of bingo squares:

a) a square on a diagonal
b) a square in the N column or on the horizontal row of the free space
c) any other square

What is the mean number of rounds (bingo numbers being called before getting a bingo when your first round outcome is (a) or (b) or (c)? What is the mean number of rounds to bingo when your first round outcome is No Match?

Another way to pose a question is:
Consider a BIngo game with four players: Abel, Betty, Carol and Zach
In the first round, Abel gets an 'a' square, Betty gets a 'b' square and Carol gets a 'c' square and Zach gets no square at all (No Match).

What is the probability of winning for each of these four players?

Youtube recommended this video for me today.

the article its based on (it's short and accessible, but uses images for the math instead of latex. if that is not a crime, it should be.)

Quote: itsmejeff

Youtube recommended this video for me today.

the article its based on (it's short and accessible, but uses images for the math instead of latex. if that is not a crime, it should be.)
link to original post

Thank you for finding that article. I can only groan after reading it. It includes this assumption:



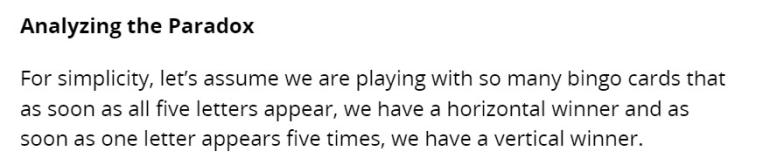
That is simply a lazy assumption. I know sometimes simplifying a problem is called for, but this goes too far.

However, it makes me feel badly for being too harsh on Matt Parker. He simply validated the conclusion of this article. However, that assumption can't be made with only 1,000 cards. I think the correct approach to analyze the "paradox" is a random simulation using actual bingo cards. Of course, that is what I did and it's easy to agree with myself.

One question on this topic. What drives this seems to be that when there are multiple simultaneous BINGOs to end a game, it’s more likely that those multiple people have a horizontal BINGO than a vertical BINGO? Is that accurate?

If so, the phenomenon makes logical sense to me.

Then if you take to an extreme, you could calculate the hypothetical of every possible BINGO card permutation was playing a game:

1) If the first five numbers called were in the B column, how many BINGO cards would be winners?

2) If the first five numbers went B then I then N then G then O, how many BINGO cards would be winners on the horizontal (and separately on the diagonal)?

Quote: unJon

One question on this topic. What drives this seems to be that when there are multiple simultaneous BINGOs to end a game, it’s more likely that those multiple people have a horizontal BINGO than a vertical BINGO? Is that accurate?

If so, the phenomenon makes logical sense to me.

Then if you take to an extreme, you could calculate the hypothetical of every possible BINGO card permutation was playing a game:

1) If the first five numbers called were in the B column, how many BINGO cards would be winners?

2) If the first five numbers went B then I then N then G then O, how many BINGO cards would be winners on the horizontal (and separately on the diagonal)?
link to original post

Good points!

I have been questioning my own post too and would like to correct myself a bit.

Matt's point is that if there were a vast number of bingo cards in play then as soon as there were a possible bingo, somebody would call it.

I might remind he said that probability of the first possible bingo would be, assuming no free square:

Vertical = 24.8%
Horizontal = 75.2%

Assuming a 5th number is called in a column. The probability any given card would have those five numbers is 1/combin(15,5) = 3003.

Assuming the last column to not have a ball called is finally called, enabling a horizontal bingo. Random simulation shows that when this happens the product of balls called in all five columns is 65.3. That makes the probability any given card will have a bingo is 65.3/15^5 = 1 in 11,629.

However, you can't simply multiply the probability of a horizontal/vertical bingo possibility by the probability any given card will have it, because the numbers are correlated by number of balls called.

After spending more hours on this, I retract my earlier comment that there will be more bingos called when they are horizontal.

Bottom line is I think the reason we see fewer vertical bingos in simulations is the effect of removal. Say B15 is the first ball called. The next ball is less likely to be another B than the other letters.

I am still not sure what the "paradox" is. At best, this is a result that would not be intuitive, but even that is not correct as there are more possible ways to draw BINGO than 5x any single column when the number of cards is exactly enough to get a bingo in n balls.

I believe that the guy screwed up his number of possible faces at 10:59. He should be permuting as order is somewhat important here. Two different cards with the same numbers in different positions will give different results. though half of them would give same results in this case as orientation does not matter.

Wiz,

If it was effect of removal then this phenomenon would show up with a single bingo card playing the game.

Quote: unJon

Wiz,

If it was effect of removal then this phenomenon would show up with a single bingo card playing the game.
link to original post

Yeah, I was thinking that while exercising this morning. However, I am looking for something in plain English to explain why horizontal bingos are more likely in multi-card games. Any suggestions?

I like Matt Parker’s videos a lot. I thought the bingo video was interesting. The thumbnail was a bit of a red herring. He did a math related outdoor playground video in Japan recently that was very good. Also, I could see Mike volunteering for his “calculate digits of pi by hand” videos.

Somewhat relatedly, I’ve played a fair amount of bingo at Plaza. When someone is 1 away (and using the computer, not paper), you hear a ding sound. Sometimes I’ll be 2 away and wonder just far behind I am. It seems to be the answer is “if someone is 1 step closer, they have a massive edge” but I can’t quantify it. Typically there are 35-70 people in the room.

Let me just type out some thoughts, maybe something here will spark an idea in someone.

Given bingo game with no free space, only horizontal and vertical bingos are considered.

It takes 5 numbers to make a bingo, so let's look at "groups of five"

This bingo game has combin(75,5) - 17,259,930 different combinations for groups of 5 numbers
Vertical bingos = 5*combin(15,5) = 15,015 combinations
Horizontal bingos = combin(15,1)^5 = 759,375 combinations

Horizontal/Vertical = 50.6 (approx)


Let's just look at a game where 16 numbers have been called: There are 2 columns with 4 numbers,2 columns with 3 numbers, and one column with 2 numbers (4,4,3,3,2).

There are zero groups of 5 vertical numbers and 288 groups of 5 horizontal numbers. With 20 bingo cards (with distinct rows and columns) there is 0 chance of a vertical bingo and a 3.8% chance of a horizontal bingo.

What happens when the 17th number is drawn to a (4,4,3,3,2) distribution ?

P=22/59 (5,4,3,3,2) Vert =1 Horiz = 360
P=24/59 (4,4,4,3,2) Vert =0, Horiz = 384
P=13/59 (4,4,3,3,3) Vert =0, Horiz = 432
So, roughly 0.0025 probability of a vertical (with 20 bingo cards) and 0.051 probability of a horizontal.
***********************************
This approach provides a methodology for calculating the probabilities of vertical and horizontal bingos as a function of the number of numbers that have been called and the number of bingo cards.

If 20 numbers have been called:
List all the possible distributions from (4,4,4,4,4) to (15,5,0,0,0) and their probabilities. Ex:
(4,4,4,4,4) 1024 of the possible 759,375 possible horizontal bingos and 0/15015 possible vertical bingos
(15,5,0,0,0) no possible horizontal bingos and 100% probability of a vertical bingo
It is straightforward (and perhaps tedious) to calculate a probabilistically averaged number of horizontal and vertical bingo combinations for any number of bingo balls(numbers) that have been drawn. (again assuming no free space).
**********************
Edit: I realize the math for going from number of combinations to probabilities is incorrect; but I was in a hurry to write out the ideas.

Wiz, I’m not sure as I don’t have my mind wrapped around the problem yet.

Let’s make a simpler version of bingo with a two by two card where each column can have 5 numbers.

So B can have 1-5 and I have 6-10.

Let’s say I’m playing by myself with the card:

1 6
2 7

Seems clear that there is an equal chance of winning vertical, horizontal or diagonal. Any pairwise combo (say 1,2 vs 1,6) has the same chance of coming first.

Now let’s say that every single possible card was going to be in play. So the game would end after 2 balls every time.

Seems clear that it’s more likely that the first two balls will be in two different columns than the same column. So there will be more horizontal and more diagonal bingos than vertical bingos. That’s the Wiz effect of removal in action.

Outstanding post above, thank you!

To put it in my own words, imagine bingo cards are 2x2 only. There are ten balls in the hopper. 1-5 are assigned to the B column and 6 to 10 to the I.

Since position matters in bingo cards, the number of possible bingo cards are p(5,2)*p(5,2) = 20^2 = 400. Where p(x,y) = number of permutations of y items out of x.

Let's assume all 400 cards are in play.

If the first two balls drawn are from the same column, we will have 2*20=40 winners. 2 for the ways the two winning numbers can be positioned on the card and 20 for the ways the other column can contains p(5,2) = 20 numbers.

If the first two balls drawn are from the different columns, we will have 2*16=32 winners. 2 for the ways the two winning numbers can be positioned on the card (top or bottom row) and 16 for the ways the other two numbers on the card that were not called.

The number of ways the first two balls called can be in the same column is 4 and different is 5.

So total winning cards are:

vertical: 4*20 = 80
horizontal: 5*16 = 80

Hmmm. Am I right or where is our point of departure?

Just out of curiosity, I redid the 1000-card simulation with no free square and no diagonals. Here are the win counts:

Horizontal = 438317 (58%)
Vertical = 317091 (42%)
Total = 775408

Quote: Wizard

Quote: unJon

One question on this topic. What drives this seems to be that when there are multiple simultaneous BINGOs to end a game, it’s more likely that those multiple people have a horizontal BINGO than a vertical BINGO? Is that accurate?

If so, the phenomenon makes logical sense to me.

Then if you take to an extreme, you could calculate the hypothetical of every possible BINGO card permutation was playing a game:

1) If the first five numbers called were in the B column, how many BINGO cards would be winners?

2) If the first five numbers went B then I then N then G then O, how many BINGO cards would be winners on the horizontal (and separately on the diagonal)?
link to original post

Good points!

I have been questioning my own post too and would like to correct myself a bit.

Matt's point is that if there were a vast number of bingo cards in play then as soon as there were a possible bingo, somebody would call it.

I might remind he said that probability of the first possible bingo would be, assuming no free square:

Vertical = 24.8%
Horizontal = 75.2%

Assuming a 5th number is called in a column. The probability any given card would have those five numbers is 1/combin(15,5) = 3003.

Assuming the last column to not have a ball called is finally called, enabling a horizontal bingo. Random simulation shows that when this happens the product of balls called in all five columns is 65.3. That makes the probability any given card will have a bingo is 65.3/15^5 = 1 in 11,629.

However, you can't simply multiply the probability of a horizontal/vertical bingo possibility by the probability any given card will have it, because the numbers are correlated by number of balls called.

After spending more hours on this, I retract my earlier comment that there will be more bingos called when they are horizontal.

Bottom line is I think the reason we see fewer vertical bingos in simulations is the effect of removal. Say B15 is the first ball called. The next ball is less likely to be another B than the other letters.
link to original post

Based on your “bottom line” comments, perhaps (some of) you have already realized this… If we compare the math behind bingo and video poker, one could say that vertical bingos resemble flushes and horizontal (including diagonal) bingos resemble straights. (Don’t make the mistake of confusing diagonals with straight flushes, as they are merely a specific case of horizontals.) I thought this analogy might help explain the phenomenon qualitatively…

PS I have no insight ATM regarding the 2X2 query presented.

Here is a simulation where various numbers of cards from 100 to 1000 were in play at once, and what percentage of the time the winning card had a horizontal, a vertical, or a diagonal bingo.

The numbers in each row add up to more than 100% as if, say, a game ended with a horizontal bingo on one card and a vertical on another (or, for that matter, on the same card), it counted as both.

The number of games played is in millions; for example, there were 2,065,000,000 games with 100 cards played.

# of cards	Millions of games	% Horizontal	% Vertical	% Diagonal
100	2,065	37.3553	29.9806	39.4867
150	1,377	37.4182	29.013	41.1205
200	1,033	37.455	28.464	42.2644
250	826	37.6588	27.9315	43.1366
300	689	37.8649	27.5951	43.7846
350	590	37.9625	27.2837	44.5281
400	517	37.9083	27.1692	45.1114
450	459	38.222	26.8481	45.6383
500	413	38.3511	26.701	46.0191
550	376	38.446	26.6114	46.442
600	345	38.6525	26.54	46.7478
650	318	38.7991	26.4472	47.0814
700	295	38.8047	26.3464	47.462
750	276	38.9018	26.1855	47.879
800	259	39.0807	26.1151	48.1822
850	243	39.1012	26.0761	48.4679
900	230	39.5083	25.8391	48.7861
950	218	39.4986	26.0917	48.9317
1000	207	39.6749	26.0623	49.0333

Good comments above. What still keeps me up at night is the probability is equal for a vertical and horizonal bingo for a single card, but not when many cards are competing. I am still searching for a solid mathematical explanation.

I'm already going down the path of pure math with a 3x3 card, but it's getting messy. Even that may not get at the "why" issue.

Quote: Wizard

Outstanding post above, thank you!

To put it in my own words, imagine bingo cards are 2x2 only. There are ten balls in the hopper. 1-5 are assigned to the B column and 6 to 10 to the I.

Since position matters in bingo cards, the number of possible bingo cards are p(5,2)*p(5,2) = 20^2 = 400. Where p(x,y) = number of permutations of y items out of x.

Let's assume all 400 cards are in play.

If the first two balls drawn are from the same column, we will have 2*20=40 winners. 2 for the ways the two winning numbers can be positioned on the card and 20 for the ways the other column can contains p(5,2) = 20 numbers.

If the first two balls drawn are from the different columns, we will have 2*16=32 winners. 2 for the ways the two winning numbers can be positioned on the card (top or bottom row) and 16 for the ways the other two numbers on the card that were not called.

The number of ways the first two balls called can be in the same column is 4 and different is 5.

So total winning cards are:

vertical: 4*20 = 80
horizontal: 5*16 = 80

Hmmm. Am I right or where is our point of departure?
link to original post

I concur. Let me step back and ask what your simulation is counting. If a bingo game ends with multiple winners, then does each winner count towards the total of vertical or horizontal winners?

If not, then you would still get 5/9 of the winners games ending with horizontal bingos and 4/9 ending in vertical bingos on a 2x2 bingo card with 10 numbers.

If so, then I wonder if the two extremes may be equal but not say if some number of cards between 1 card and every card is in play.

Quote: unJon

I concur. Let me step back and ask what your simulation is counting. If a bingo game ends with multiple winners, then does each winner count towards the total of vertical or horizontal winners?

Yes, each winner counts equally.

Quote:

If so, then I wonder if the two extremes may be equal but not say if some number of cards between 1 card and every card is in play.
link to original post

I tend to think not, but have no good argument why. I think if we expanded this to a 3x3 card, where there could be 3 to 5 numbers called before a bingo, then we would start to see a bias towards the horizontal bingos. I started down that path in Excel, but probably won't finish.

Here's another extreme "bounding case": a 5x5 grid with 70/75 balls already drawn, and only one bingo card

XXXXO
XXXOX
XXOXX
XOXXX
OXXXX

When the 71st ball is drawn, you will have both a horizontal and a vertical bingo. Somehow this bounding case is part of the same mathematical system as a 5x5 grid with one million players, or a 2x2 grid with ten numbers. And I don't think you can understand this mathematical system by examining its extremums. Its like trying to derive the theory of prime numbers by examining the smallest prime number "2". It's not merely a limiting case, it's a trivial and non-typical case.

I suspect that the ratio of horizontal to vertical bingos is a function of how many rounds it takes (on average) for the first bingo to appear. And examining games with an nxn grid with a zillion players in which it takes only n or n+1 rounds to end is probably not going to reveal the answer. Because the essential factors driving the mathematics are not present.

Previously, I posted a rambling discussion about developing a combinatorial math approach to calculating the liklihood of vertical and horizontal bingos on a single bingo card as a function of the round in the bingo game. I'm still looking at that, and think I may have a chance at the Fields Medal, if only I weren't over 40 years of age.

Here is a new table based on a game with no free square and no diagonal wins, with every possible card in play. It shows, by simulation, the probability any given card wins, according to the type of possible bingo.

Bingo Type	Probability First Possible Bingo	Probability Random Card Wins	Product	Ratio
Horizontal	75.20%	0.000121	0.000091	52.3%
Vertical	24.80%	0.000333	0.000083	47.7%
Total	100.00%		0.000173	100.0%

You can see it shows that 52.34% of bingos will be horizontal. However, by direction simulation, I get 54.15%. I do not understand the reason for the 2% difference.

The 0.000121 figure that a random card wins on a horizontal bingo is based on a mixture of math and 18.313 combinations of daubs on the other four columns, per a simulation.

Edit: Perhaps the difference is my simulation of an overall game used 1,000 cards. I'm going to increase that to see how things change...

I made a number of changes to my simple multi-card simulation program other than increasing the number of cards to 10,000.

After running it, I get these results:

Vertical = 41.9%
Horizontal = 58.1%

I'm not happy these results are so much different that 1,000 cards. I suspect one of the simulations, at least, had a bug.

I reran the simulation for 1,000 cards are got these results:

Vertical = 42.14%
Horizontal = 57.86%

For one card, it still comes out 50/50.

This talk about two columns reminds me of a bingo game I occasionally seen called duo dab. Each card has 25 spaces with the free space in the middle. Each space has 2 numbers in it. Once of the the numbers was called the space was considered marked. This was always a coverall game. Made in interesting. Coverall normally took about 35 numbers with these cards

Quote: Wizard

I made a number of changes to my simple multi-card simulation program other than increasing the number of cards to 10,000.

After running it, I get these results:

Vertical = 41.9%
Horizontal = 58.1%

I'm not happy these results are so much different that 1,000 cards. I suspect one of the simulations, at least, had a bug.

I reran the simulation for 1,000 cards are got these results:

Vertical = 42.14%
Horizontal = 57.86%

For one card, it still comes out 50/50.
link to original post

I’m still confused by this.

What makes logical sense to me is that if a horizontal bingo is about 1.5x as likely then there should also be 1.5x as many horizontal bingo cards winning such that the EV of winning vertically and horizontally is the same when you factor in chance of sharing bingo prize.

But the simulation does not appear to show that?

I have taken a pretty deep dive into this. My latest direction is a pure math solution, like Matt Parker did. Here is an expansion of the table in his video that also shows the probability that any given card will form a vertical bingo given the number of daubs per column. This is assuming the O column gets the next ball, making a horizontal bingo possible. Of course, I multiply by 5 in the "Winning Card" column to adjust that it could be any column which gets the last ball.

B	I	N	G	Balls before bingo	Ways	Probability Calls	Winning Card	Product
1	1	1	1	4	1	0.04399779	0.00000658	0.00000029
1	1	1	2	5	4	0.08799558	0.00001317	0.00000116
1	1	1	3	6	4	0.03315775	0.00001975	0.00000065
1	1	2	2	6	6	0.08034379	0.00002634	0.00000212
1	1	1	4	7	4	0.01023989	0.00002634	0.00000027
1	1	2	3	7	12	0.07167926	0.00003951	0.00000283
1	2	2	2	7	4	0.03859653	0.00005267	0.00000203
1	1	2	4	8	12	0.02567615	0.00005267	0.00000135
1	1	3	3	8	6	0.01854389	0.00005926	0.0000011
1	2	2	3	8	12	0.05991103	0.00007901	0.00000473
2	2	2	2	8	1	0.00806495	0.00010535	0.00000085
1	1	3	4	9	12	0.01517227	0.00007901	0.0000012
1	2	2	4	9	12	0.02450906	0.00010535	0.00000258
1	2	3	3	9	12	0.03540197	0.00011852	0.0000042
2	2	2	3	9	4	0.0190626	0.00015802	0.00000301
1	1	4	4	10	6	0.00350129	0.00010535	0.00000037
1	2	3	4	10	24	0.03267874	0.00015802	0.00000516
1	3	3	3	10	4	0.0078671	0.00017778	0.0000014
2	2	2	4	10	4	0.00879812	0.0002107	0.00000185
2	2	3	3	10	6	0.0190626	0.00023704	0.00000452
1	2	4	4	11	12	0.00842499	0.0002107	0.00000178
1	3	3	4	11	12	0.01216943	0.00023704	0.00000288
2	2	3	4	11	12	0.01965831	0.00031605	0.00000621
2	3	3	3	11	4	0.00946511	0.00035556	0.00000337
1	3	4	4	12	12	0.00695396	0.00031605	0.0000022
2	2	4	4	12	6	0.00561666	0.0004214	0.00000237
2	3	3	4	12	12	0.0162259	0.00047407	0.00000769
3	3	3	3	12	1	0.00195312	0.00053333	0.00000104
1	4	4	4	13	4	0.00145809	0.0004214	0.00000061
2	3	4	4	13	12	0.01020662	0.0006321	0.00000645
3	3	3	4	13	4	0.0049143	0.00071111	0.00000349
2	4	4	4	14	4	0.0023425	0.0008428	0.00000197
3	3	4	4	14	6	0.00507542	0.00094815	0.00000481
3	4	4	4	15	4	0.00253771	0.0012642	0.00000321
4	4	4	4	16	1	0.00051614	0.0016856	0.00000087
				Total		0.75177861	0	0.00009064

This shows that the probability a random card gets a horizontal bingo as soon as a bingo is possible is 0.00009064. We divide that by 0.75177861, to get the probability of a horizontal bingo given that all five columns were called before 5 balls were drawn in any one column. That conditional probability is 0.000120572.

Let's go back to a table I have tried to construct before showing the overall probability of a vertical and horizontal bingo assuming all possible cards are in play.

Overall	Matt Parker	Prob bingo formed	Product	Ratio
Horizontal	0.75177861	0.00012057	0.00009064	0.52304015
Vertical	0.24822139	0.000333	0.00008266	0.47695985
Total	1	0	0.0001733	1

So, bottom line is a 52.3% chance a bingo is horizontal.

I admit this is not in agreement with my 10,000 card simulation at 58.07%. Why, I am not sure. However, it does agree the results in this post.

I welcome all comments and corrections.

Kind of off topic, but if cards are created using this paten, only 2971 of the possible 3003 G row combinations of numbers are used.

Quote: ThatDonGuy

Here is a simulation where various numbers of cards from 100 to 1000 were in play at once, and what percentage of the time the winning card had a horizontal, a vertical, or a diagonal bingo.

The numbers in each row add up to more than 100% as if, say, a game ended with a horizontal bingo on one card and a vertical on another (or, for that matter, on the same card), it counted as both.

The number of games played is in millions; for example, there were 2,065,000,000 games with 100 cards played.

# of cards	Millions of games	% Horizontal	% Vertical	% Diagonal
100	2,065	37.3553	29.9806	39.4867
150	1,377	37.4182	29.013	41.1205
200	1,033	37.455	28.464	42.2644
250	826	37.6588	27.9315	43.1366
300	689	37.8649	27.5951	43.7846
350	590	37.9625	27.2837	44.5281
400	517	37.9083	27.1692	45.1114
450	459	38.222	26.8481	45.6383
500	413	38.3511	26.701	46.0191
550	376	38.446	26.6114	46.442
600	345	38.6525	26.54	46.7478
650	318	38.7991	26.4472	47.0814
700	295	38.8047	26.3464	47.462
750	276	38.9018	26.1855	47.879
800	259	39.0807	26.1151	48.1822
850	243	39.1012	26.0761	48.4679
900	230	39.5083	25.8391	48.7861
950	218	39.4986	26.0917	48.9317
1000	207	39.6749	26.0623	49.0333

link to original post

I'm looking for something to check my own simulation against. So, I ran mine with 1,000 cards, a free square and diagonal wins and get these figures:

Vertical: 29.14%
Horizontal: 30.50%
Diagonal: 40.35%

If I divide your figures, Don, by the sum of the probabilities, 1.147705, we still don't agree. What did you do if two different cards won for the same type of bingo at the same time? Personally, I count all wins.

Quote: miplet

Kind of off topic, but if cards are created using this paten, only 2971 of the possible 3003 G row combinations of numbers are used.
link to original post

Hmmm. Can you tell us in layman's terms why the patent is blocking certain cards?

Quote: Wizard

Quote: miplet

Kind of off topic, but if cards are created using this paten, only 2971 of the possible 3003 G row combinations of numbers are used.
link to original post

Hmmm. Can you tell us in layman's terms why the patent is blocking certain cards?
link to original post

It uses relative Prime numbers. 3001, 2999, 1321, 2971, and 2998 for the rows. And 113 (23 for n) for the order of the rows.

I felt good enough about my "every possible card" answer to publish it today in Ask the Wizard #406.

Quote: Wizard

I'm looking for something to check my own simulation against. So, I ran mine with 1,000 cards, a free square and diagonal wins and get these figures:

Vertical: 29.14%
Horizontal: 30.50%
Diagonal: 40.35%

If I divide your figures, Don, by the sum of the probabilities, 1.147705, we still don't agree. What did you do if two different cards won for the same type of bingo at the same time? Personally, I count all wins.
link to original post

I don't. I am trying to count what percentage of the games ended with, say, a horizontal win. It doesn't matter how many cards have the horizontal win at once; it is the number of games that matters.

Quote: ThatDonGuy

Quote: Wizard

I'm looking for something to check my own simulation against. So, I ran mine with 1,000 cards, a free square and diagonal wins and get these figures:

Vertical: 29.14%
Horizontal: 30.50%
Diagonal: 40.35%

If I divide your figures, Don, by the sum of the probabilities, 1.147705, we still don't agree. What did you do if two different cards won for the same type of bingo at the same time? Personally, I count all wins.
link to original post

I don't. I am trying to count what percentage of the games ended with, say, a horizontal win. It doesn't matter how many cards have the horizontal win at once; it is the number of games that matters.
link to original post

If all simultaneous winners chop the prize, then it would make sense to count a fractional win when more than one card wins on a certain ball. This would mean the win counts would sum to the number of games played. With one card in play, it is possible that all three types of bingos hit on one ball. It is imperative to give each type (H, V, D) a fair share of the win when there are simultaneous wins on one card or multiple cards.

With a million cards in play, simultaneous wins on one card is less important. The winning cards will almost never make more than one bingo.

I am writing a generalized simulator that can handle 2x2 to 5x5 cards. I can create 100,000 unique 5x5 bingo cards per second. I will use a single integer bitwise operation to check whether any of the 12 bingos occurred. This will be very fast and allow a lot of pipelining of the card scoring algorithm. I should be able to give Mike some numbers to check against his sims.

Quote: Mental

If all simultaneous winners chop the prize, then it would make sense to count a fractional win when more than one card wins on a certain ball. This would mean the win counts would sum to the number of games played. With one card in play, it is possible that all three types of bingos hit on one ball. It is imperative to give each type (H, V, D) a fair share of the win when there are simultaneous wins on one card or multiple cards.
link to original post

I agree that fractional wins make logical sense. I have posted before where I did chop the pot. However, for now, I'm trying to keep it simple and get at how many bingos are horizontal/vertical if there are thousands of cards in play. I'm still looking for a confirmation that my simulator is working correctly, which counts all wins equally.

i've used a different method to look at bingo lines starting with 0-0-0-0-0 and going up to 5-5-5-5-5, using blackjack logic that says what's the chances of the next [card] and those chances of winning. While 5-1-1-1-1 has both a vertical and horizontal winner, you would have already had a winner earlier (e.g. 4-1-1-1-1 or 5-x-x-x-x).
The net is I get the same chances of the winner being vertical as 0.248221389.
Obviously when there's a vertical winner it's when the first 5 numbers in a column come out, so as you say 1 in 3003 cards win. With a horizontal win, it depends on how many numbers in different columns have come out. So typically there will be multiple winning lines.

Quote: Wizard

I agree that fractional wins make logical sense. I have posted before where I did chop the pot. However, for now, I'm trying to keep it simple and get at how many bingos are horizontal/vertical if there are thousands of cards in play. I'm still looking for a confirmation that my simulator is working correctly, which counts all wins equally.
link to original post

Here you go - a simulation of games with anywhere from 100 to 2000 cards, with the number of horizontal, vertical, and diagonal winning cards per 100 games:

Quote: ThatDonGuy

1000

2,309

49.2317

46.4605

64.1357

link to original post

I'm happy to report my own simulation is in close agreement with these figures. Here are my exact results:

Horizontal: 261870
Vertical: 247642
Diagonal: 341765

How do you calculate how many cards are needed to simulate the results? It is some number between 2 and all possible cards, but it does not appear to be 1000.

Also, the rules are such that diagonal bingos do not matter. If a horizontal bingo is possible, it must exist. Therefore, any diagonal will exist with at least one horizontal (minimum number of calls) or many of them.

Quote: itsmejeff

How do you calculate how many cards are needed to simulate the results? It is some number between 2 and all possible cards, but it does not appear to be 1000.

Also, the rules are such that diagonal bingos do not matter. If a horizontal bingo is possible, it must exist. Therefore, any diagonal will exist with at least one horizontal (minimum number of calls) or many of them.
link to original post

Each simulation is based on a predetermined number of cards. If you check my post that Wizard responds to, you will see that I have simulations for different numbers of cards from 100 to 2000. The number of cards has a definite effect on the results.

As for your second statement, it is true only if every possible card is in play. If the first four numbers happen to all be on the same diagonal of one card, then it is highly unlikely that any card has the same numbers in the middle row of the card (they can't be on any other row as it would not be a bingo since no N numbers have been called yet).

NB: I may have got my maths wrong (as I have to go out so can't check it, but you see the idea).
I don't know if this helps but technically (although some will be mirror images of others) there are 3003 ways to have five numbers in any column, however they can be in a different order, so actually it is 15x14x13x12x11 = 360,360.
The other four columns also have the same perms. So the total number of "unique" tickets are 360360⁵ (call this N).

Just considering five numbers drawn causing a win.
If there are five numbers in one column then there are 360360⁴ ways for the other columns and 5x4x3x2x1 ways to organise the five drawn numbers. This is N/3003 - i.e. 1 in 3003 tickets win.

If there are five numbers, one in each column, then
(i) each column has to have the drawn number in that column (and hence any other 14) =14x13x12x11/4/3/2/1. So initially, ignoring the order within each column, this is 1001⁵. This is N/((15*24)⁵).
(ii) Now while a card might have the winning numbers, they have to appear in one of seven lines (five across or two diagonal).
This means, for each of those lines, the winning number has to be in the correct position and the other four can be in any order.
So the multiplier is (4x3x2x1)⁵*7 = 55738368.
This means 1 in 108482 cards win.

The chances of a 5 numbers in a column being called = 1*14/89...11/86 = 1 in 2439.
The chances of 5 numbers, 1 in each column = 1*60/89...15/86 = 1 in 48 (or 1 in 50.77 times more likely)

So N/3003/2439.186 + N/108482/48.2296 cards (about N/7324878+N/5232056) will win on the 5th number. (This agrees with, if you were playing one card, then it has 5 ways to win on a vertical column and 7 ways to win on a horizontal or diagonal one.)

The problem with 9th numbers and beyond is you have to cater for cards with two winning lines.

The only thing I could see is going from 16 numbers (no winner) to 17 numbers is there are 15 numbers to make a horizontal winner and 44 to make a vertical winner.

Let Ph = the probability that a horizontal line hits for the first win.
Let Pv = the probability that a vertical line hits for the first win.
Let Pd = the probability that a diagonal line hits for the first win.
I weight my probabilities for split wins. For example, if I have 1000 cards and there are 10 simultaneous winners, then I divide Nh, Nv, and Nd by 10 and add that to my total winners for each category. If I simulate G games, I divide the totals by G to get a normalized probability. As a result, Ph + Pv + Pd = 1.0.

In order to understand the 'paradox', I examined a very simple bingo game. The simplest bingo game is a square 1x1 with one number in it. Clearly, Ph = Pv = 1/4 and Pd = 1/2. That is, the first number called always results in a H and V win and two D wins on a winning card. This is not a very interesting game.

If I uses a 2x2 bingo card, and I only have two balls per row, I get a more interesting game. Ph = Pv = Pd = 1/3. If I break the probabilities down to the six unique bingo lines:
V0 0.167
V1 0.167
H2 0.167
H3 0.167
D4 0.167
D5 0.167
In this game, the first two numbers always make a bingo on every card. There are only four numbers on a card and only four balls numbered 1-4.

Now, I change my game to allow numbers 1-3 in the first column and 4-6 in the second column. I use 1000 cards and simulate 1,000,000 games. (This takes 4 seconds of CPU time.)
V0 0.200
V1 0.200
H2 0.150
H3 0.150
D4 0.150
D5 0.150
You can prove this is correct because there are 15 equally probable ways for the first two balls to come up, and three of these (12, 13, and 23) make V0 a winner. The cases where one ball is in the range 1,2,3 and the other ball is in the range 4,5,6 are equally likely to make a H or D winner.

When I use 15 balls per column, I get an unexpected result: Ph < Pd
V0 0.243
V1 0.241
H2 0.128
H3 0.128
D4 0.130
D5 0.130
I have not thought this through yet to come up with the mathematical explanation.

What I want to emphasize is that the 'paradox' is easily explained for the 2x2 bingo game with 6 balls, but it is OPPOSITE in direction compared to the bingo paradox in the OP. There are more vertical bingo winners compared to horizontal bingo winners.

My code is very efficient and is parameterized. I can change the size from 2x2 to 5x5 by changing one parameter. Likewise, the number of balls per column is changed by changing another parameter. Here are the results for a 4x4 bingo game with 15 balls per column. Note that the paradox flips to favor H and D winners.
V0 0.085
V1 0.085
V2 0.086
V3 0.085
H4 0.110
H5 0.110
H6 0.109
H7 0.111
D8 0.109
D9 0.110

I am able to use two bitwise operations ((win & board) == win) to determine if a bingo was hit. This makes the program very fast, but it limits me to 64 balls in play to stuff the bits into a 64-bit number. This means that I can simulate a 4x4x15 game, but I need to limit myself to only 12 numbers per column for a 5x5 game.

I ran a 5x5 bingo game where there are only 5 numbers per column. Above, I show a random card out of the 1000 cards that I generated. The 12 winning lines are shown above as 25-bit numbers. A # indicates that a bit is set. For the V lines, the 25-bit number for a winner has the first 5 bits set. The H and D lines have one bit set per group of 5 bits.

As I simulate a game, I start with a 25-bit number representing the board. I randomly add bits to the board simulating the random picking of balls from the hopper. I use the logical AND operator between a winning line and the board to see if the board has all of the bits for the winner set. If (H5 AND BOARD == H5), there is a bingo on H5. Below, I show what this process looks like after seven numbers have been called.

The probabilities for the 12 lines are very biased against vertical lines. Remember that I am splitting the bingo prize with all bingo cards that have a simultaneous bingo. If the number 1-5 are called first, then every single card will have a V0 bingo. Each card will only get credit for 0.001 prizes. The result is that less than 7% of the prize pool goes to vertical bingos.

V0 0.0123
V1 0.0124
V2 0.0123
V3 0.0123
V4 0.0126
H5 0.1336
H6 0.1333
H7 0.1352
H8 0.1338
H9 0.1345
Da 0.1331
Db 0.1348

If you count raw V, H, and D bingos, then you will get a different result than mine. The 'paradox' is partly the result of the smaller number of combinations that produce a vertical bingo and the large number of cards that share numbers within a vertical column. In my extreme example, all columns on every card require the same 5 numbers to be called.