Best chance of reaching profit target with Martingale

Threads: 25
Posts: 2463

Joined: Mar 29, 2011

May 15th, 2015 at 6:50:26 AM permalink

ok
nice question
what do you think?

using a coin flip example (50/50) i get the chance to hit the target B4 the first BIG loss
= 77.247620% 4step
for 5 step = 77.569988%

so looks to be a close race
i made a correction below:
in a simulation, because after the 1st loss there could still be some $$$$ to play a shorter Marty
(or bold play back to the starting bankroll would be my choice)
i get
4step: 80%
5step: 80%

close is close in a 50/50 game
i say
=========================
why the interest?

I Heart Vi Hart

OnceDear

Threads: 64
Posts: 7534

Joined: Jun 1, 2014

May 15th, 2015 at 9:27:51 AM permalink

Indeed a good question, which is unusual from a Martyman.

From totally anecdotal experiment, I suggest that more steps makes for better probability up until the variance gets too smoothed out.

'd suggest starting stake in the region of 32nd of bankroll.

Drooling over the prospects of Sally's math proof.

Psalm 25:16 Turn to me and be gracious to me, for I am lonely and afflicted. Proverbs 18:2 A fool finds no satisfaction in trying to understand, for he would rather express his own opinion.

ThatDonGuy

ThatDonGuy

Threads: 122
Posts: 6678

Joined: Jun 22, 2011

May 15th, 2015 at 12:33:14 PM permalink

Quote: OnceDear
Drooling over the prospects of Sally's math proof.

I don't know about Sally, but here's what I get:

Assuming you are only interested in which is more likely to win: eight consecutive 4-step Martingales, or four consecutive 5-step ones, the 5-step winning 4 times is always more likely (except, of course, when the probability of each individual bet winning is either 0 or 1).

EDIT: Say, here's a crazy idea; how about I calculate the probabilities for the question you actually asked - eight 5-step Martingales vs four 4-step ones

Let P5 = the probability of the eight 5-step Martingales winning, and P4 = the probability of the four 4-step martingales winning.
p = the probability of a specific bet losing

P5 = (1 - p⁵)⁸ = 1 - 8 p⁵ + 28 p¹⁰ - 56 p¹⁵ + 70 p²⁰ - 56 p²⁵ + 28 p³⁰ - 8 p³⁵ + p⁴⁰
P4 = (1 - p⁴)⁴ = 1 - 4 p⁴ + 6 p⁸ - 4 p¹² + p¹⁶
P5 > P4 when P5 - P4 > 0
P5 - P4 = 4 p⁴ - 8 p⁵ - 6 p⁸ + 28 p¹⁰ + 4 p¹² - 56 p¹⁵ - p¹⁶+ 70 p²⁰ - 56 p²⁵ + 28 p³⁰ - 8 p³⁵ + p⁴⁰

Some quick spreadsheet analysis shows that P5 - P4 > 0 for all values of p between 0 and 0.50866 - that is, if the probability of an individual bet winning is 0.49134 or greater - and P5 - P4 < 0 for all values of p between 0.50866 and 1.
In other words, if the probability of winning is 0.49134 or greater, the 5-step 8-bet Martingale is better, and if it is less, then the 4-step 4-bet Martingale is better.
For double-zero roulette, the winning probability = 0.48718 < 0.49134, so in that case, the 4-step Martingale is better.
For the pass line in craps, the winning probability = 0.49293 > 0.49134, so in that case, the 5-step is better.

surrender88s

surrender88s

Threads: 20
Posts: 291

Joined: Jun 23, 2013

May 15th, 2015 at 12:43:10 PM permalink

I'll add the chances of winning with a bet of $200 is 75%.

Chances of winning with $100 original bet is 76.5625%.

These are simple calculations and assume the biggest possible loss as total failure. Actually, 800-200-400=200, so you'd actually have a better chance than 75% if you were willing to keep going. Same story with the $100 bets, where you still have $100 (to turn into $1000).

"Rule No.1: Never lose money. Rule No.2: Never forget rule No.1." -Warren Buffett on risk/return

EvenBob

EvenBob

Threads: 442
Posts: 29515

Joined: Jul 18, 2010

May 15th, 2015 at 1:35:01 PM permalink

Quote: surrender88s
I'll add the chances of winning with a bet of $200 is 75%.

So you win $25 3 out of 4 times, and
lose $200 in 1 out of 4? Hitting your
head with a hammer sounds less
painful than that..

"It's not called gambling if the math is on your side."

surrender88s

surrender88s

Threads: 20
Posts: 291

Joined: Jun 23, 2013

May 15th, 2015 at 1:37:29 PM permalink

no, you win $200 75% of the time and lose $600 25% of the time. (on a no house edge 50/50/bet).

"Rule No.1: Never lose money. Rule No.2: Never forget rule No.1." -Warren Buffett on risk/return

mustangsally

Threads: 25
Posts: 2463

Joined: Mar 29, 2011

May 15th, 2015 at 2:15:11 PM permalink

Quote: ThatDonGuy
Assuming you are only interested in which is more likely to win:
eight consecutive 4-step Martingales,
or
four consecutive 5-step ones,

the 5-step winning 4 times is always more likely

is that so?
for the example in the OP, as a start,
it really depends on what the rule is to stop after a system loss

a major loss at 4 or 5 step means that one can not continue the 4 or 5 step full betting
so even with bankroll remaining is the system aborted?

if true
the 00 roulette 18/38 = p
4step-4win $50: 72.662129% (p=50%: 77.247620%)
5step-8win $25: 71.907173% (p=50%: 77.569988%)

that is why i simulated so after a major loss
the betting continues until ruin
the 18/38 00 roulette (had to edit because of error in min bet)
4step-4win $50: 74.34%
5step-8win $25: 74.45%

MAYbe time to calculate using a Markov chain

winner winner chicken dinner (i had last night)

I Heart Vi Hart

mustangsally

Threads: 25
Posts: 2463

Joined: Mar 29, 2011

May 15th, 2015 at 2:19:48 PM permalink

Quote: EvenBob
So you win $25 3 out of 4 times, and lose $200 in 1 out of 4? Hitting your head with a hammer sounds less painful than that..

sounds MAYbe,
how that feels, no

I Heart Vi Hart

EvenBob

EvenBob

Threads: 442
Posts: 29515

Joined: Jul 18, 2010

May 15th, 2015 at 4:00:10 PM permalink

Quote: surrender88s
no, you win $200 75% of the time and lose $600 25% of the time. (on a no house edge 50/50/bet).

If the progression is 25-50-100-200, you win
$25 3 times, and lose $200 once.

"It's not called gambling if the math is on your side."

ThatDonGuy

ThatDonGuy

Threads: 122
Posts: 6678

Joined: Jun 22, 2011

May 15th, 2015 at 6:59:54 PM permalink

Quote: mustangsally
is that so?

Well, it does if you compare 4 5-step Martingales against 8 4-step ones, which is what I was doing...oh, and you missed where I had something raised to the 4th power on one side of an equation and the same value raised to the 5th power on the other.

mustangsally

Threads: 25
Posts: 2463

Joined: Mar 29, 2011

May 15th, 2015 at 9:59:09 PM permalink

Quote: ThatDonGuy
oh, and you missed where I had something raised to the 4th power on one side of an equation and the same value raised to the 5th power on the other.

i was playing with a laptop today and having fun with video stuff...

then back to this
I also corrected my first post
the 4 and 5 step probability to turn $800 into $1000 in a 50/50 game = 800/1000 or 80%
i set the wrong min bet in me sim

the only difference is the avg # of trials
9 for 5 step
17 for 4 step

the OP should think about doubling the proper bankroll for each Marty as one big loss then upsets trying to keep the same Marty going

of course, the OP is still out to dinner

I Heart Vi Hart

mustangsally

Threads: 25
Posts: 2463

Joined: Mar 29, 2011

May 16th, 2015 at 4:55:08 PM permalink

Quote: ThatDonGuy
For the pass line in craps, the winning probability = 0.49293 > 0.49134, so in that case, the 5-step is better.

i still need to digest your data
i see what you are doing but
after 2 days of rain and lots of food eating
I can go all night on the dance floor and will again!

i finally made a few absorbing transition matrices that deal with a Marty
yes
i be the 1st on my street!

for the pass line example
OP
says an $800 bankroll and a $1000 target ("a goal to profit $200 with $800 to risk")

i turned this onto units just because i can and it for me is way more fun

4 step success = 0.787912107
5 step success = 0.785512109

4 step from 16 units ($50)

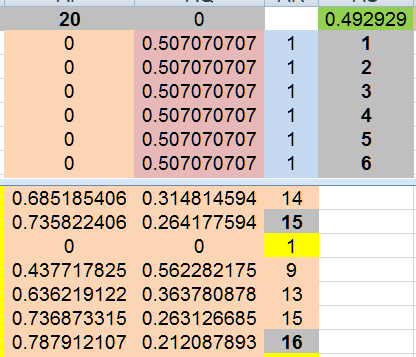
5 step from 32 units ($25)

this was fun
more on the way tomorrow

I Heart Vi Hart

imluckynotgood

Threads: 1
Posts: 7

Joined: May 15, 2015

May 17th, 2015 at 3:24:31 AM permalink

Thanks everyone for the discussion and feedback! I've been away from the computer for a couple days so just now seeing the responses. After initial skimming it looks as if the probability differences aren't that significant. The question arose because since I live 3 minutes from the strip and see casino life every day, the gamble of a quick couple hundred profit is more fun and exciting than being able to last at the table for 4 hours without going broke (maybe more so what a tourist would be in to).

Thanks again for the continued doscussion!

imluckynotgood

Threads: 1
Posts: 7

Joined: May 15, 2015

May 17th, 2015 at 3:36:15 AM permalink

Also, I was assuming whatever leftover is not used of the $800 would not be utilized. So really:

Make $200 with $750 (50,100,200,400 stop)
Make $200 with $775 (25,50,100,200,400 stop)
Make $200 with $700 (100,200,400 stop) etc.

OnceDear

Threads: 64
Posts: 7534

Joined: Jun 1, 2014

May 17th, 2015 at 3:59:13 AM permalink

Quote: imluckynotgood
Also, I was assuming whatever leftover is not used of the $800 would not be utilized. So really:

Make $200 with $750 (50,100,200,400 stop)
Make $200 with $775 (25,50,100,200,400 stop)
Make $200 with $700 (100,200,400 stop) etc.

Hi Lucky,

You don't need to Marty to have a good chance of turning your 800 into 1000. You can stand a good chance flat betting in a way that I do quite often....

I'll describe by analogy, if I may.

Lets say, for the sake of interest, that you are staggering randomly along a track between two parellel lines 10 metres apart, walking generally in parallel with the lines. You start out 8 metres from the line on your left and 2 metres from the line on your right. There's a slight sidewind blowing you right to left (that's the house edge)

Now, if you are taking tiny stems (wagers) then your stagger will tend to follow a steady curve which will eventually meet the left line ( loss of bankroll). If the steps are really tiny, then the randomness of the stagger will be barely noticeable and you'll never get near the right line (winning objective met).

But, what if you're lurching is a bit wild, say half metre swings to left or right for each step forward, then your proximity to the right hand line (win goal) will make it more likely that you will hit that line (win goal) before the left line of ruin. Does that make sense when you visualise it?

Try it maybe as a scribbled random line on a bit of paper between two straight parallel lines. You hit the nearest line first on most occasions,as long as you don't go too wild with the randomness.

Back to reality.....
Embrace the variance.

With your $800 stake and $1000 goal, assuming a 50:50 type of wager, why not flat bet at about $25 to $50 each time. I'd guestimate a nearly a 80% probability of meeting win goal before going broke.

Then you could get silly and set a new $1100 win goal, then another :o) That's the behaviour that usually ruins me and which is a bit like the Russian roulette of Marty.

Have fun. Don't try it too often.

Psalm 25:16 Turn to me and be gracious to me, for I am lonely and afflicted. Proverbs 18:2 A fool finds no satisfaction in trying to understand, for he would rather express his own opinion.

imluckynotgood

Threads: 1
Posts: 7

Joined: May 15, 2015

May 17th, 2015 at 4:32:17 AM permalink

Thanks for the analogy OD. My appeal to the Martingale is that since my game of choice is BJ, it's always a nice added bonus to get that massive 3:2 when you're in defcon 2 or 1 haha. Of course splitting 88s can get a bit hairy

OnceDear

Threads: 64
Posts: 7534

Joined: Jun 1, 2014

May 17th, 2015 at 4:57:00 AM permalink

Quote: imluckynotgood
Thanks for the analogy OD. My appeal to the Martingale is that since my game of choice is BJ, it's always a nice added bonus to get that massive 3:2 when you're in defcon 2 or 1 haha. Of course splitting 88s can get a bit hairy

I know what you mean. About 4 or 5 times now, my sessions at a real casino have culminated with splitting up to about 5 hands and doubling on some of those. That's real 5h1t or bust territory. Last time, it busted my session out, I uttered the immortal words 'fcuk me rigid'. There was a smile and a gasp from Ms. Fellow player: The dealer chuckled and said that she could not believe I'd just said that. Fortunately it was all in good humour and nobody got at all upset. Oh how we all laughed. It was worth losing (about £100) on that hand, just for the comedy value at my expense.

You can still get the thrill of the doubles, splits and blackjacks playing it my way. Try it with a simulator or free online game. Always hold a bit back for that inevitable double Ace against 5.

$:o)

Psalm 25:16 Turn to me and be gracious to me, for I am lonely and afflicted. Proverbs 18:2 A fool finds no satisfaction in trying to understand, for he would rather express his own opinion.

mustangsally

Threads: 25
Posts: 2463

Joined: Mar 29, 2011

May 17th, 2015 at 6:55:27 AM permalink

Quote: imluckynotgood
Thanks for the analogy OD. My appeal to the Martingale is that since my game of choice is BJ

what game of BJ?
in other words the rules of BJ that you like to play

does it have surrender and do you use it?

if you play BS then the distribution of one round can be calculated (better yet and faster simulated) to get the probability distribution
something like this (without the probability)
Net win (unit)
8
7
6
5
4
3
2
1.5
1
0
-0.5
-1
-2
-3
-4
-5
-6
-7
-8

for one round. Then a calculation can be made for the Marty success

let us know for fun!

I Heart Vi Hart

mustangsally

Threads: 25
Posts: 2463

Joined: Mar 29, 2011

May 17th, 2015 at 7:08:38 AM permalink

Quote: OnceDear
With your $800 stake and $1000 goal, assuming a 50:50 type of wager, why not flat bet at about $25 to $50 each time.

MAYbe time is a factor
the chance to win $200 flat betting $25 starting with $800 is at best 70% (craps pass line no odds)
and 00 Roulette (18/38) drops to abouts 42%

but the expected number of bets abouts 280 give or take
TIME

the chance to win $200 flat betting $50 starting with $800 is at best 75% (craps pass line no odds)
and 00 Roulette (18/38) drops to abouts 61%

but the expected number of bets abouts 70 give or take
again TIME

Quote: OnceDear
I'd guestimate a nearly a 80% probability of meeting win goal before going broke.

for flat betting
Use the Gambler's Ruin formula that has been around since the 1600s

i figured correctly the OP wants to Marty BJ
so i MAY see the results first if I attempt it, unless someone simulates this 1st
and i can do that also
my 1st feeling is one can win $200 at pass line at craps or even red/black at 0 Roulette
faster and more often than BJ with Marty
i could be way wrong here too
but
TIME
MAY
tell

many will cry out "NEVER Marty playing BJ"

well, i say
thank you for that opinion
and let them cry out

I Heart Vi Hart

OnceDear

Threads: 64
Posts: 7534

Joined: Jun 1, 2014

May 17th, 2015 at 7:38:03 AM permalink

Quote: mustangsally
MAYbe time is a factor
the chance to win $200 flat betting $25 starting with $800 is at best 70% (craps pass line no odds)
and 00 Roulette (18/38) drops to abouts 42%

but the expected number of bets abouts 280 give or take

TIME

many will cry out "NEVER Marty playing BJ"

well, i say
thank you for that opinion
and let them cry out

Ah. Valid point I guess. Time may be a a factor.
I think we can agree that the difference in ROR between flat betting 10% bankroll and 5 step Marty is not huge. Marty does it quicker win or lose.
I suspect your 70 bets is a tiny bit on the high side. Wagering 10% of bankroll, I'd expect win goal in a few dozen hands of BJ ( from limited experience)

But then what's the hurry? The risk that we take is for the benefit of entertainment, not profit. I don't mind spinning out my wagering for extended playtime. Hitting win goal in 5 hands of Blackjack with Marty would not keep me from under the wife's feet for long. Reverse Marty can be fun too and is especially good for extending playtime.

And I don't cry 'Never Marty playing BJ.' Marty is fun and I even do it myself for fun, just like Russian roulette would be fun (with a water pistol. I'm not insane.)

Psalm 25:16 Turn to me and be gracious to me, for I am lonely and afflicted. Proverbs 18:2 A fool finds no satisfaction in trying to understand, for he would rather express his own opinion.

ThatDonGuy

ThatDonGuy

Threads: 122
Posts: 6678

Joined: Jun 22, 2011

May 17th, 2015 at 8:05:58 AM permalink

Quote: mustangsally

4 step success = 0.787912107
5 step success = 0.785512109

I have a feeling we're solving two slightly different problems - for example, you're considering this as a Gambler's Ruin problem.

Let p = 244/495 = the probability of winning a pass line bet in craps
The probability of 4 wins without a run of 4 losses win a row = (1 - (1 - p)⁴)⁴ = 78.3946843%
The probability of 8 wins without a run of 5 losses win a row = (1 - (1 - p)⁵)⁸ = 78.9566619%

mustangsally

Threads: 25
Posts: 2463

Joined: Mar 29, 2011

May 17th, 2015 at 8:21:18 AM permalink

Quote: ThatDonGuy
I have a feeling we're solving two slightly different problems - for example, you're considering this as a Gambler's Ruin problem.

yes it is fun
i did it both ways too (not for the pass)

Quote: ThatDonGuy
Let p = 244/495 = the probability of winning a pass line bet in craps
The probability of 4 wins without a run of 4 losses win a row = (1 - (1 - p)⁴)⁴ = 78.3946843%
The probability of 8 wins without a run of 5 losses win a row = (1 - (1 - p)⁵)⁸ = 78.9566619%

Excel and Wolfram do agree not to agree with your answers but agree with your formula
but you are close

The probability of 4 wins without a run of 4 losses win a row = 76.064311%
The probability of 8 wins without a run of 5 losses win a row = 76.125868%

i agree p=244/495
looks to me you have p=251/495

now on the Blackjack
and i say it (BJ) performs even worse compared using a Marty

I Heart Vi Hart

imluckynotgood

Threads: 1
Posts: 7

Joined: May 15, 2015

May 18th, 2015 at 1:17:17 AM permalink

Quote: mustangsally
what game of BJ?
in other words the rules of BJ that you like to play

does it have surrender and do you use it?

According to WoV BJ survey it's a 0.29 house edge game. 6 decks, stand S17, surrender on any, DAS, RSA etc. Assume proper basic strategy with no counting or play variations

imluckynotgood

Threads: 1
Posts: 7

Joined: May 15, 2015

May 18th, 2015 at 1:21:24 AM permalink

Quote: OnceDear

But then what's the hurry? The risk that we take is for the benefit of entertainment, not profit.

imluckynotgood

Threads: 1
Posts: 7

Joined: May 15, 2015

May 18th, 2015 at 1:23:04 AM permalink

Quote: imluckynotgood
The question arose because since I live 3 minutes from the strip and see casino life every day, the gamble of a quick couple hundred profit is more fun and exciting than being able to last at the table for 4 hours without going broke (maybe more so what a tourist would be looking for.

:)

mustangsally