November 17th, 2021 at 7:59:28 AM
permalink
I知 interested in the answer, but I知 more interested in the method for how to approach this. In the middle of a dream last night I thought of this number:
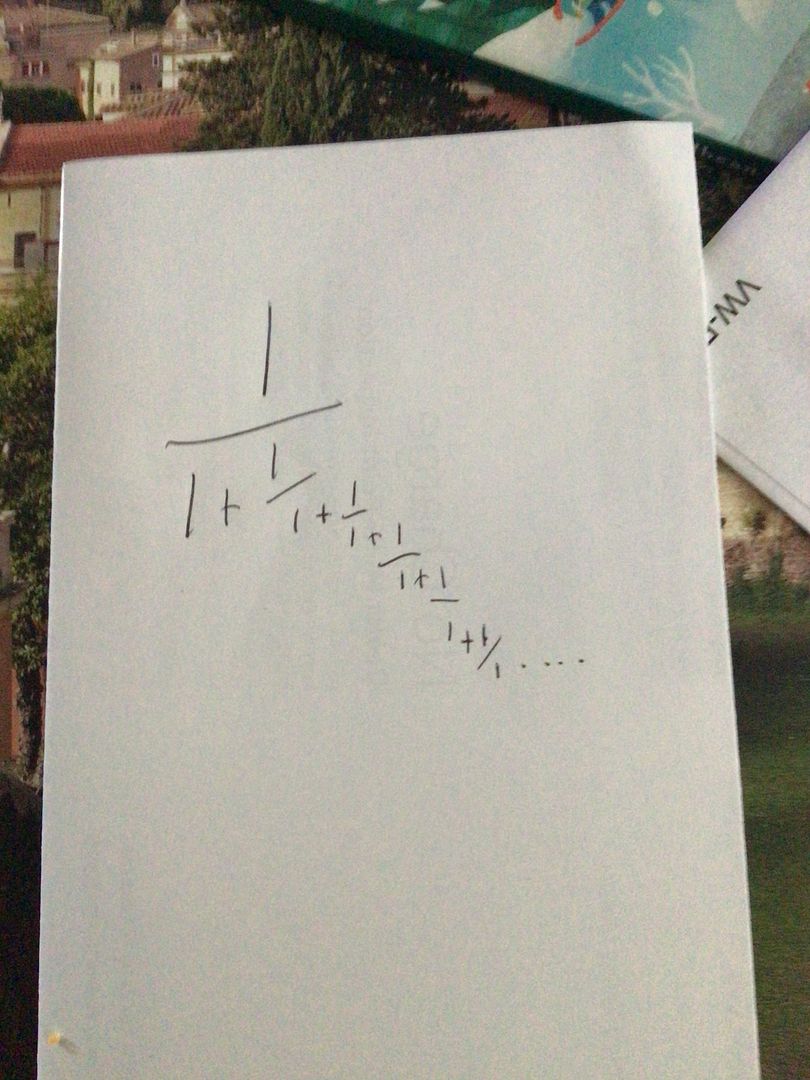
And in the dream I was trying to figure out what number it was. I knew (in the dream) that it approached a limit, but since I was dreaming it just turned into one of those circular dreams where I kept getting dumped to the beginning.
It looks like it痴 just a tick over 1/2, but how do I think about it to find out what it really is?
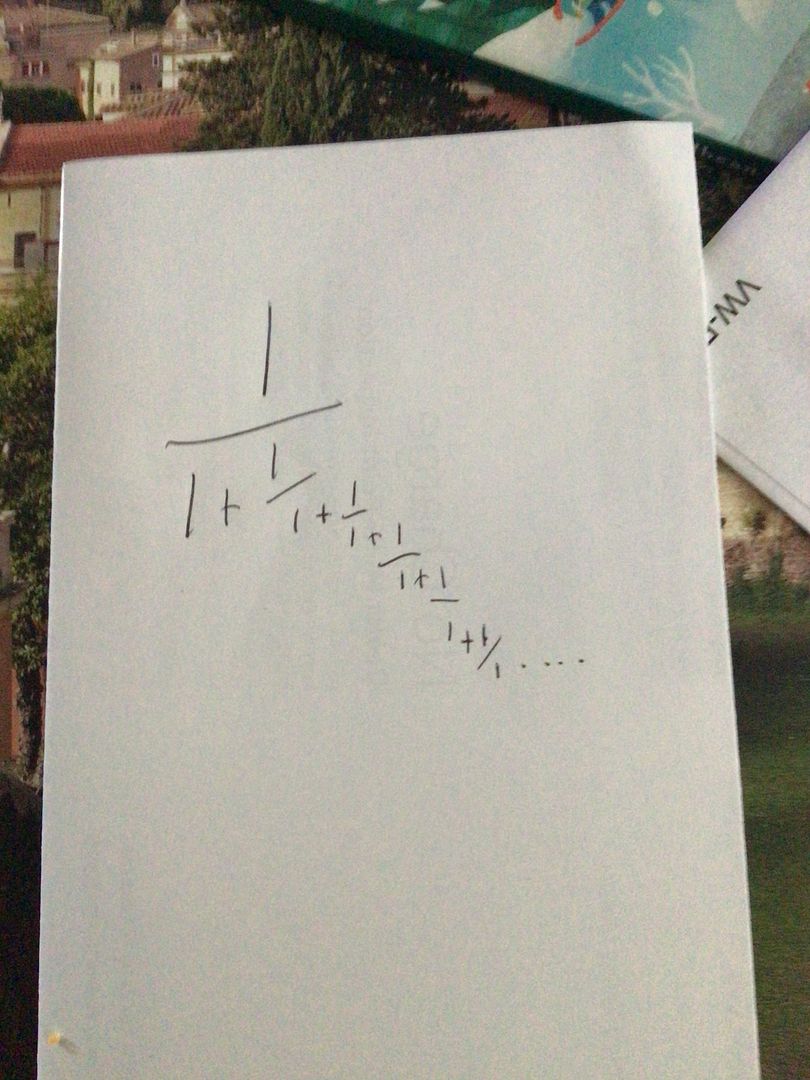
And in the dream I was trying to figure out what number it was. I knew (in the dream) that it approached a limit, but since I was dreaming it just turned into one of those circular dreams where I kept getting dumped to the beginning.
It looks like it痴 just a tick over 1/2, but how do I think about it to find out what it really is?
A falling knife has no handle.
November 17th, 2021 at 8:02:54 AM
permalink
An easy one...
Let X = 1 / (1 + 1 / (1 + 1 / (1 + 1 / (....)
1 / X = 1 + 1 / (1 + 1 / (1 + 1 / (....)
1 / X - 1 = 1 / (1 + 1 / (1 + 1 / (....) = X
X - 1 / X + 1 = 0
X^2 + X - 1 = 0
X = -1/2 +/- sqrt(5)/2
but in this case, X > 0, so X = (sqrt(5) - 1) / 2
Let X = 1 / (1 + 1 / (1 + 1 / (1 + 1 / (....)
1 / X = 1 + 1 / (1 + 1 / (1 + 1 / (....)
1 / X - 1 = 1 / (1 + 1 / (1 + 1 / (....) = X
X - 1 / X + 1 = 0
X^2 + X - 1 = 0
X = -1/2 +/- sqrt(5)/2
but in this case, X > 0, so X = (sqrt(5) - 1) / 2
November 17th, 2021 at 8:07:55 AM
permalink
Quote: ThatDonGuyAn easy one...
Let X = 1 / (1 + 1 / (1 + 1 / (1 + 1 / (....)
1 / X = 1 + 1 / (1 + 1 / (1 + 1 / (....)
1 / X - 1 = 1 / (1 + 1 / (1 + 1 / (....) = X
X - 1 / X + 1 = 0
X^2 + X - 1 = 0
X = -1/2 +/- sqrt(5)/2
but in this case, X > 0, so X = (sqrt(5) - 1) / 2
link to original post
Thanks, ThatDonGuy! I don稚 algebra much, so I had to write that down and look at it as I did it. But I got it. The method is what I was looking for. Dreams are strange.
A falling knife has no handle.


