Three Card side bet - FLUSH3

1) 8 decks, 3 cards each for the dealer and player
2) A side bet called FLUSH3 :-
a) When the winner (dealer or player) has a FLUSH hand, 1 pay 8
b) When both dealer and player have the same rank of FLUSH, e.g. 4s5s9s vs player 4c5c9c, it is a tie.
c) All other outcomes will lose 1 unit, for example:-
i) 3s4s5s vs 4c5c9c, lose 1 unit, because the winner's hand is not FLUSH but a straight flush.
ii) 3s3s7s vs 6c6c6h, lose 1 unit, because the winner's hand is not FLUSH but Three of a kind.

3) Order of rank: Three of a kind, Straight Flush, Flush, Straight, Pair . . . .

What is the house edge ?

Combination analysis shows that ev = + 1.99883%, but I am not confident, can someone help me verify it?

Edited

This game is too complicated. Why not make this simpler for everybody? A player flush is paid 9 to 1.

Is the combination considered to be 3 or 6 cards?
What if the player/dealer has 2 or 4 cards?

So, this is not blackjack? Is it three card poker?

Anyway, I understand that player and dealer are both dealt three cards.

If either the player or the dealer has a flush it is likely (with a probability >97%) that that hand (the flush) will be the winning hand. Therefore, the most important probability is whether either player or dealer has a 3 card flush. The odds of that occurring is about 1 in 8 (an infinite deck approximation.) Actually the odds should be longer than that because sometimes both dealer and player will have a 3-card flush on the same deal. So maybe 0.94 in 8.

With a payout of 8, I don't see any way that this bet could be +EV for the player. (ignoring counting, etc.)

Quote: Dobrij

Is the combination considered to be 3 or 6 cards?
What if the player/dealer has 2 or 4 cards?
link to original post

It is Three Card poker, each player and dealer have 3 cards.

Quote: gordonm888

So, this is not blackjack? Is it three card poker?

Anyway, I understand that player and dealer are both dealt three cards.

If either the player or the dealer has a flush it is likely (with a probability >97%) that that hand (the flush) will be the winning hand. Therefore, the most important probability is whether either player or dealer has a 3 card flush. The odds of that occurring is about 1 in 8 (an infinite deck approximation.) Actually the odds should be longer than that because sometimes both dealer and player will have a 3-card flush on the same deal. So maybe 0.94 in 8.

With a payout of 8, I don't see any way that this bet could be +EV for the player. (ignoring counting, etc.)
link to original post

It is three card poker. Please note that this is 8 decks(penetration about 70%), not a single deck.

The probability to have a FLUSH = 5.867%(For single deck it is 4.96%), and this hand(Flush) will win 97% of the time, so the winning probability = 5.69%, since the winner can come from either side, so the total winning probability is about = 5.69 * 2 = 11.38% - -, So EV = 8*0.1138 -0.8862 = +2.43%, the actual EV should slightly lower than +2.43%.

Quote: ssho88

1) 8 decks, 3 cards each for the dealer and player
2) A side bet called FLUSH3 :-
a) When the winner (dealer or player) has a FLUSH hand, 1 pay 8
b) When both dealer and player have the same rank of FLUSH, e.g. 4s5s9s vs player 4c5c9c, it is a tie.
c) All other outcomes will lose 1 unit, for example:-
i) 3s4s5s vs 4c5c9c, lose 1 unit, because the winner's hand is not FLUSH but a straight flush.
ii) 3s3s7s vs 6c6c6h, lose 1 unit, because the winner's hand is not FLUSH but Three of a kind.

3) Order of rank: Three of a kind, Straight Flush, Flush, Straight, Pair . . . .

What is the house edge ?

Combination analysis shows that ev = + 1.99883%, but I am not confident, can someone help me verify it?

Edited
link to original post

Can you please confirm that “3)” is correct? Although the distribution of the hands changes between 1 and 8 decks, I am not seeing a difference in the hand ranks based on probability. In other words, in order from least to most likely, the ranks are Straight Flush, Trips, Straight, Flush, Pair, Ace High, etc, for both 1 and 8 decks.

Also, with 8 decks, combination hands exist, like suited Trips or suited Pair + Singleton. How are each of these hands ranked?

Can you post the combinations you calculated for each hand type? This should be for each rank. The total I get is 11,912,160.

Thanks for your reply.

Here are the combination analysis and simulations results.



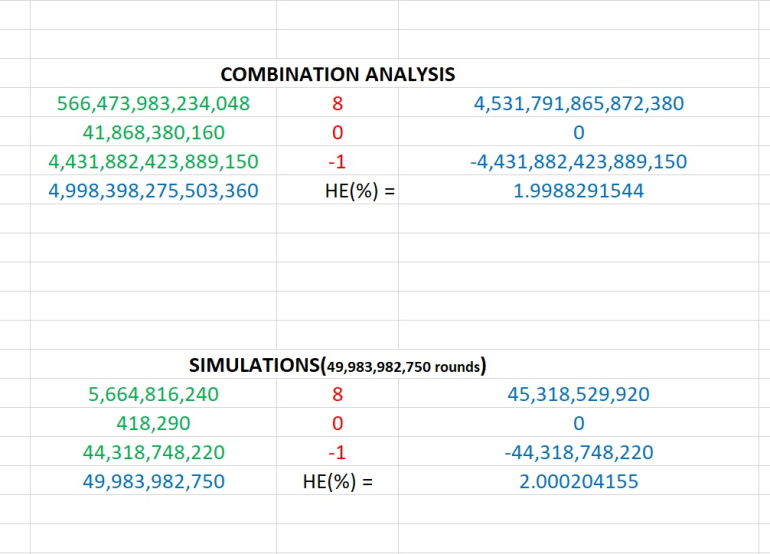
Hand ranks set by the casino : Three of a kind, Straight Flush, Flush, Straight, Pair . . . .it does not matter whether hand ranks based on probability or not, as long as we know who is the winner. I just deliberately simulated 49,983,982,750 rounds to make it easier to compare. You can see that the two results are very close.

If suited Trips, it is considered to be Trips, because Trips is higher then Flush. If (suited Pair + suited Singleton), then it is FLUSH, not Pair.

Quote: camapl

Quote: ssho88

1) 8 decks, 3 cards each for the dealer and player
2) A side bet called FLUSH3 :-
a) When the winner (dealer or player) has a FLUSH hand, 1 pay 8
b) When both dealer and player have the same rank of FLUSH, e.g. 4s5s9s vs player 4c5c9c, it is a tie.
c) All other outcomes will lose 1 unit, for example:-
i) 3s4s5s vs 4c5c9c, lose 1 unit, because the winner's hand is not FLUSH but a straight flush.
ii) 3s3s7s vs 6c6c6h, lose 1 unit, because the winner's hand is not FLUSH but Three of a kind.

3) Order of rank: Three of a kind, Straight Flush, Flush, Straight, Pair . . . .

What is the house edge ?

Combination analysis shows that ev = + 1.99883%, but I am not confident, can someone help me verify it?

Edited
link to original post

Can you please confirm that “3)” is correct? Although the distribution of the hands changes between 1 and 8 decks, I am not seeing a difference in the hand ranks based on probability. In other words, in order from least to most likely, the ranks are Straight Flush, Trips, Straight, Flush, Pair, Ace High, etc, for both 1 and 8 decks.

Also, with 8 decks, combination hands exist, like suited Trips or suited Pair + Singleton. How are each of these hands ranked?

Can you post the combinations you calculated for each hand type? This should be for each rank. The total I get is 11,912,160.
link to original post

Draw 3 cards from 8 deck shoe, based on hand ranks : Three of a kind, Straight Flush, Flush, Straight, Pair, High card

RANK	COMBINATION
TRIPS	64,480
STRAIGHT FLUSH	24,576
FLUSH	700,928
STRAIGHT	368,640
PAIR	1,078,272
HIGH CARD	9,675,264
TOTAL	11,912,160

Quote: ssho88

Quote: camapl

Quote: ssho88

1) 8 decks, 3 cards each for the dealer and player
2) A side bet called FLUSH3 :-
a) When the winner (dealer or player) has a FLUSH hand, 1 pay 8
b) When both dealer and player have the same rank of FLUSH, e.g. 4s5s9s vs player 4c5c9c, it is a tie.
c) All other outcomes will lose 1 unit, for example:-
i) 3s4s5s vs 4c5c9c, lose 1 unit, because the winner's hand is not FLUSH but a straight flush.
ii) 3s3s7s vs 6c6c6h, lose 1 unit, because the winner's hand is not FLUSH but Three of a kind.

3) Order of rank: Three of a kind, Straight Flush, Flush, Straight, Pair . . . .

What is the house edge ?

Combination analysis shows that ev = + 1.99883%, but I am not confident, can someone help me verify it?

Edited
link to original post

Can you please confirm that “3)” is correct? Although the distribution of the hands changes between 1 and 8 decks, I am not seeing a difference in the hand ranks based on probability. In other words, in order from least to most likely, the ranks are Straight Flush, Trips, Straight, Flush, Pair, Ace High, etc, for both 1 and 8 decks.

Also, with 8 decks, combination hands exist, like suited Trips or suited Pair + Singleton. How are each of these hands ranked?

Can you post the combinations you calculated for each hand type? This should be for each rank. The total I get is 11,912,160.
link to original post

Draw 3 cards from 8 deck shoe, based on hand ranks : Three of a kind, Straight Flush, Flush, Straight, Pair, High card

RANK	COMBINATION
TRIPS	64,480
STRAIGHT FLUSH	24,576
FLUSH	700,928
STRAIGHT	368,640
PAIR	1,078,272
HIGH CARD	9,675,264
TOTAL	11,912,160

link to original post

My single-hand analysis agrees with yours above.

Quote: ssho88

Thanks for your reply.

Here are the combination analysis and simulations results.



Hand ranks set by the casino : Three of a kind, Straight Flush, Flush, Straight, Pair . . . .it does not matter whether hand ranks based on probability or not, as long as we know who is the winner. I just deliberately simulated 49,983,982,750 rounds to make it easier to compare. You can see that the two results are very close.

If suited Trips, it is considered to be Trips, because Trips is higher then Flush. If (suited Pair + suited Singleton), then it is FLUSH, not Pair.
link to original post

My 2-hand probability estimates agree closely enough with your “COMBINATION ANALYSIS”. The only number I have difficulty with is the probability of a tie. However, given that our win and loss probabilities agree, and that your total permutations figure is correct for the total number of “Player and Dealer” 3-card hands out of 8 decks, I can’t dispute the 2.0% RTP.

It’s interesting that the casino has ranked the hands as such, as it creates more winners on the side bet. I can’t decide if it does anything to the return of the base game, aside from being confusing! I would imagine that using 8 decks alters optimal strategy from that of single deck. As Q-6-4 is the median hand in single deck, what do you find it to be in 8 deck, and what is the RTP? (I want to say this is discussed in another thread or in an appendix on WoO…)

The best thread I’ve found regarding Three Card Poker delt from multiple decks is here:

https://wizardofvegas.com/forum/gambling/tables/37120-3-card-poker-with-multiple-decks/#post848376

Unfortunately, the analysis of RTP and strategy are for a 4-deck version that someone deals at home. As this analysis was done by none other than YOU 😂😂😂, I assume that you’ve already analyzed the base game of your 8-deck version… Has the casino altered the pays on the Ante Bonus as compared to single deck?

Quote: camapl

The best thread I’ve found regarding Three Card Poker delt from multiple decks is here:

https://wizardofvegas.com/forum/gambling/tables/37120-3-card-poker-with-multiple-decks/#post848376

Unfortunately, the analysis of RTP and strategy are for a 4-deck version that someone deals at home. As this analysis was done by none other than YOU 😂😂😂, I assume that you’ve already analyzed the base game of your 8-deck version… Has the casino altered the pays on the Ante Bonus as compared to single deck?
link to original post

It is actually not a normal 3 CARD POKER, only two spots( "PLAYER" and "BANKER") in a round, each spot draw 3 cards, you can bet the base game on either "PLAYER" or "BANKER", 1 pay 0.95. So there is no BS and no counting strategy for base game. 😂😂😂, I

Quote: ssho88

c) All other outcomes will lose 1 unit, for example:-
i) 3s4s5s vs 4c5c9c, lose 1 unit, because the winner's hand is not FLUSH but a straight flush.
link to original post

I understand what you're trying to do, but you're gonna get a lot of *long* arguments from players when you say that a straight flush isn't a flush. It might be worth tinkering with the numbers to include straight flushes as winners.